Ex 11.2, 8 (i) - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

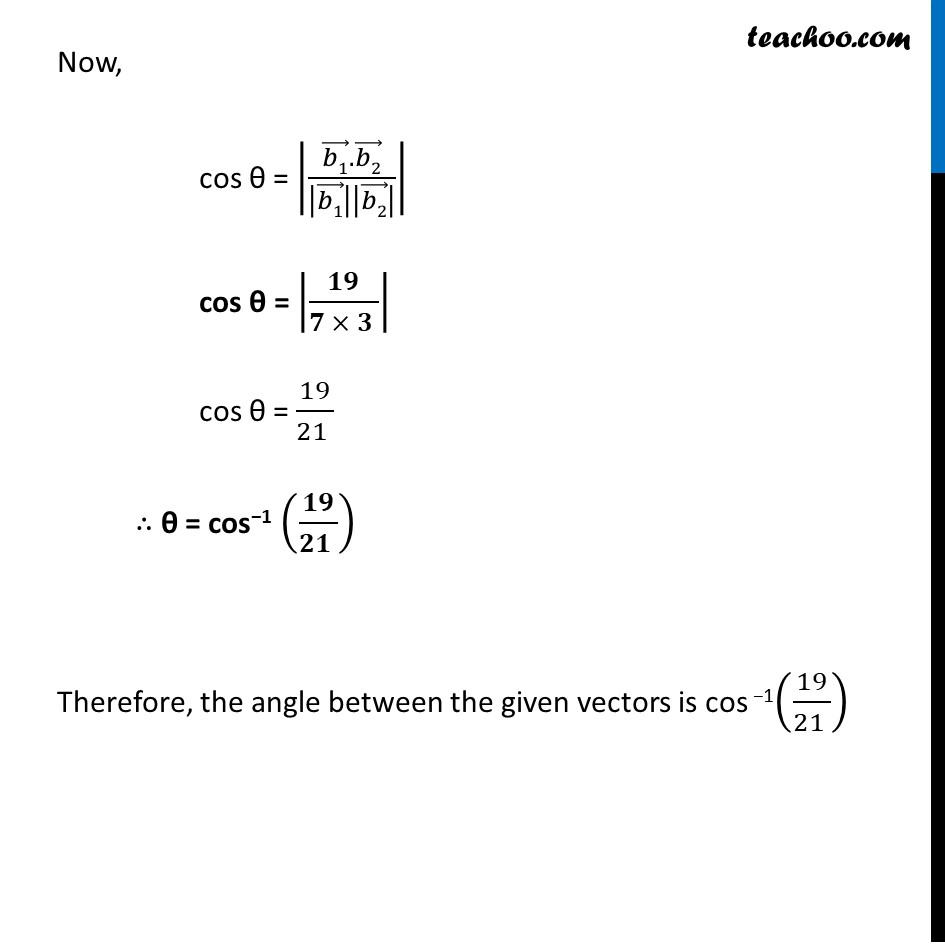
Ex 11.2, 8 Find the angle between the following pairs of lines: (i) 𝑟 ⃗ = 2𝑖 ̂− 5𝑗 ̂ + 𝑘 ̂ + 𝜆 (3𝑖 ̂ + 2𝑗 ̂ + 6𝑘 ̂) and 𝑟 ⃗ = 7𝑖 ̂ – 6𝑘 ̂ + 𝜇(𝑖 ̂ + 2𝑗 ̂ + 2𝑘 ̂) Angle between two vectors 𝑟 ⃗ = (𝑎1) ⃗ + 𝜆 (𝑏1) ⃗ & 𝑟 ⃗ = (𝑎2) ⃗ + 𝜇 (𝑏2) ⃗ is given by cos θ = |((𝒃𝟏) ⃗ . (𝒃𝟐) ⃗)/|(𝒃𝟏) ⃗ ||(𝒃𝟐) ⃗ | | Given, the pair of lines is 𝒓 ⃗ = (2𝒊 ̂ − 5𝒋 ̂ + 𝒌 ̂) + 𝜆 (3𝒊 ̂ + 2𝒋 ̂ + 6𝒌 ̂) So, (𝑎1) ⃗ = 2𝑖 ̂ − 5𝑗 ̂ + 1𝑘 ̂ (𝑏1) ⃗ = 3𝑖 ̂ + 2𝑗 ̂ + 6𝑘 ̂ 𝒓 ⃗ = (7𝒊 ̂ − 6𝒌 ̂) + 𝝁 (𝒊 ̂ + 2𝒋 ̂ + 2𝒌 ̂) So, (𝑎2) ⃗ = 7𝑖 ̂ + 0𝑗 ̂ − 6𝑘 ̂ (𝑏2) ⃗ = 1𝑖 ̂ + 2𝑗 ̂ + 2𝑘 ̂ Now, (𝒃𝟏) ⃗.(𝒃𝟐) ⃗ = (3𝑖 ̂ + 2𝑗 ̂ + 6𝑘 ̂) . (1𝑖 ̂ + 2𝑗 ̂ + 2𝑘 ̂) = (3 × 1) + (2 × 2) + (6 × 2) = 3 + 4 + 12 = 19 Magnitude of (𝑏1) ⃗ = √(32 + 22 + 62) |(𝒃𝟏) ⃗ | = √(9 + 4 + 36) = √49 = 7 Magnitude of (𝑏2) ⃗ = √(12+22+22) |(𝒃𝟐) ⃗ | = √(1+4+4) = √9 = 3 Now, cos θ = |((𝑏1) ⃗.(𝑏2) ⃗)/|(𝑏1) ⃗ ||(𝑏2) ⃗ | | cos θ = |𝟏𝟗/(𝟕 × 𝟑 )| cos θ = 19/(21 ) ∴ θ = cos−1 (𝟏𝟗/(𝟐𝟏 )) Therefore, the angle between the given vectors is cos −1(19/(21 ))