Misc 9 - Chapter 8 Class 11 Sequences and Series

Transcript

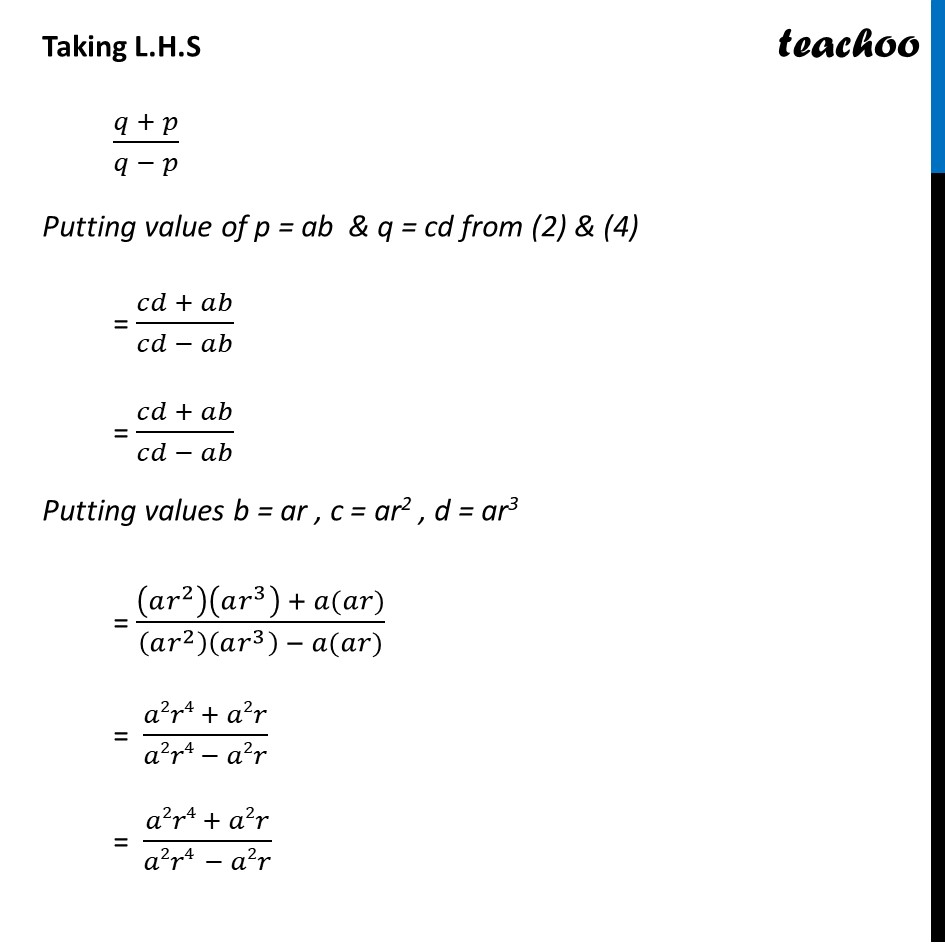
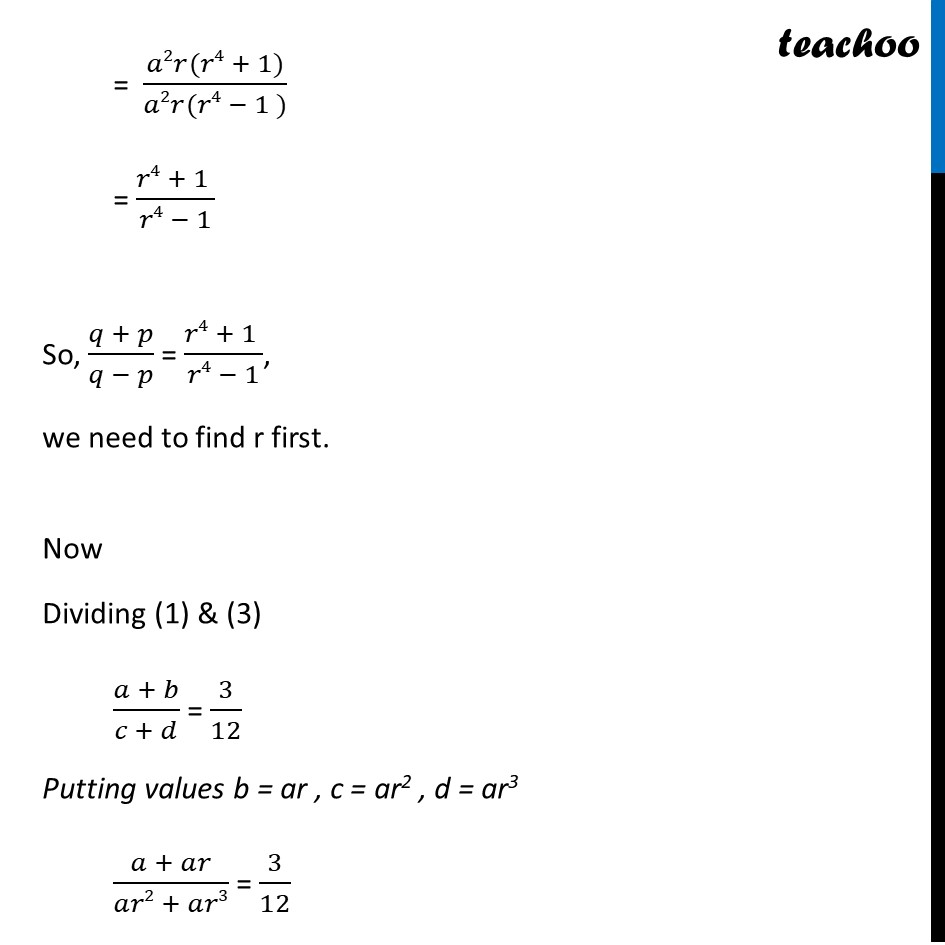
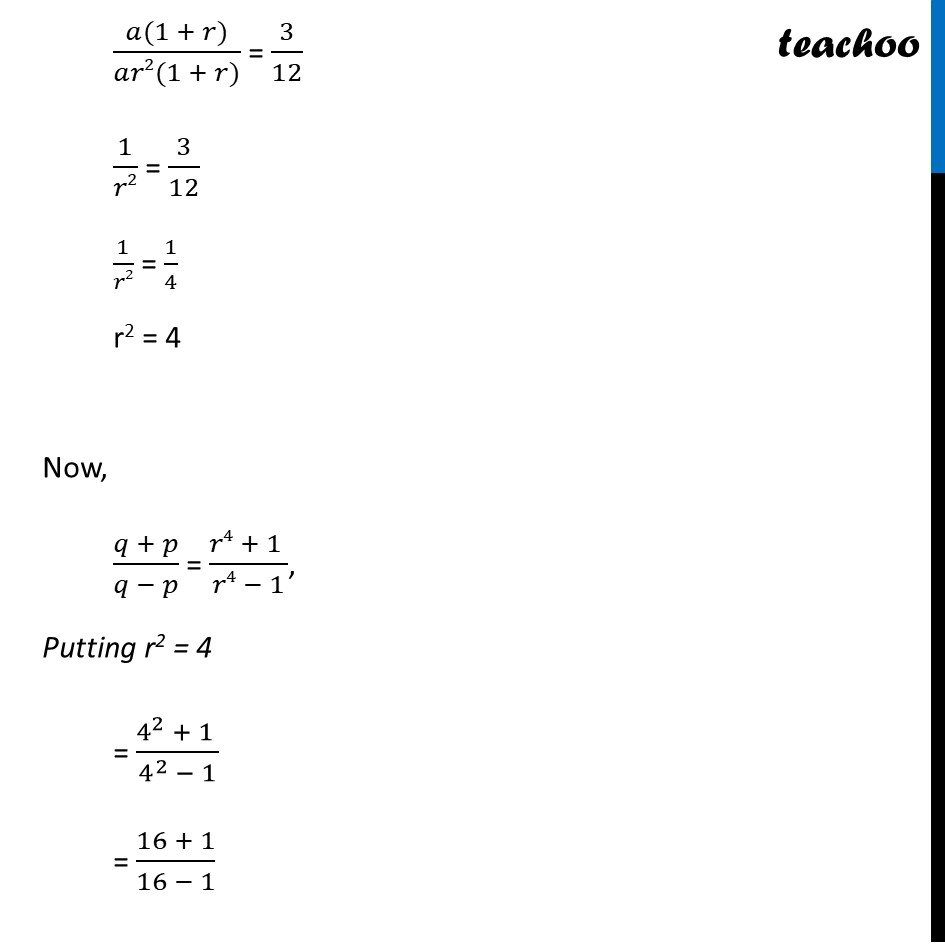
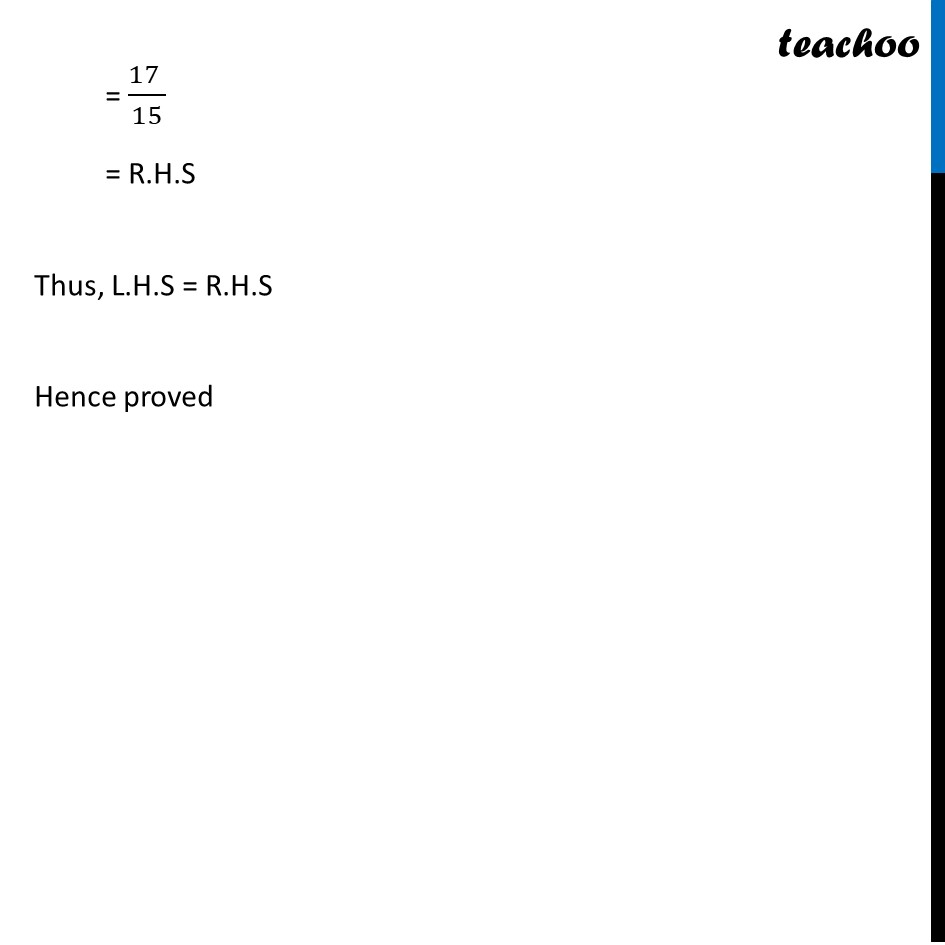
Misc 18 If a and b are the roots of x2 – 3x + p = 0 and c,d are roots of x2 – 12x + q = 0, where a, b, c, d, form a G.P. Prove that (q + p): (q – p) = 17:15. Introduction For quadratic equation ax2 + bx + c = 0 Product of roots = 𝑐/𝑎 & sum of roots = (−𝑏)/𝑎 Misc 18 If a and b are the roots of x2 – 3x + p = 0 and c,d are roots of x2 – 12x + q = 0, where a, b, c, d, form a G.P. Prove that (q + p): (q – p) = 17:15. Introduction For quadratic equation ax2 + bx + c = 0 Product of roots = 𝑐/𝑎 & sum of roots = (−𝑏)/𝑎 Misc 18 If a and b are the roots of x2 – 3x + p = 0 and c,d are roots of x2 – 12x + q = 0, where a, b, c, d, form a G.P. Prove that (q + p): (q – p) = 17:15. We know that a, ar , ar2 , ar3, …. are in G.P. with first term a & common ratio r Given a, b, c, d are in G.P. So, a = a b = ar c = ar2 d = ar3 We have to prove (𝑞 + 𝑝)/(𝑞 − 𝑝) = 17/15 Taking L.H.S (𝑞 + 𝑝)/(𝑞 − 𝑝) Putting value of p = ab & q = cd from (2) & (4) = (𝑐𝑑 + 𝑎𝑏)/(𝑐𝑑 − 𝑎𝑏) We know that a, ar , ar2 , ar3, …. are in G.P. with first term a & common ratio r Given a, b, c, d are in G.P. So, a = a b = ar c = ar2 d = ar3 We have to prove (𝑞 + 𝑝)/(𝑞 − 𝑝) = 17/15 Taking L.H.S (𝑞 + 𝑝)/(𝑞 − 𝑝) Putting value of p = ab & q = cd from (2) & (4) = (𝑐𝑑 + 𝑎𝑏)/(𝑐𝑑 − 𝑎𝑏) We know that a, ar , ar2 , ar3, …. are in G.P. with first term a & common ratio r Given a, b, c, d are in G.P. So, a = a b = ar c = ar2 d = ar3 We have to prove (𝑞 + 𝑝)/(𝑞 − 𝑝) = 17/15 Taking L.H.S (𝑞 + 𝑝)/(𝑞 − 𝑝) Putting value of p = ab & q = cd from (2) & (4) = (𝑐𝑑 + 𝑎𝑏)/(𝑐𝑑 − 𝑎𝑏) = (𝑐𝑑 + 𝑎𝑏)/(𝑐𝑑 − 𝑎𝑏) Putting values b = ar , c = ar2 , d = ar3 = ((𝑎𝑟^2 )(𝑎𝑟^3 ) + 𝑎(𝑎𝑟))/((𝑎𝑟^2 )(𝑎𝑟^3 ) − 𝑎(𝑎𝑟)) = (𝑎2𝑟4 + 𝑎2𝑟)/(𝑎2𝑟4 − 𝑎2𝑟) = (𝑎2𝑟4 + 𝑎2𝑟)/(𝑎2𝑟4 − 𝑎2𝑟) = (𝑎2𝑟(𝑟4 + 1))/(𝑎2𝑟(𝑟4 − 1 )) = (𝑟4 + 1 )/(𝑟4 − 1) So, (𝑞 + 𝑝)/(𝑞 − 𝑝) = (𝑟4 + 1 )/(𝑟4 − 1), we need to find r first. = (𝑐𝑑 + 𝑎𝑏)/(𝑐𝑑 − 𝑎𝑏) Putting values b = ar , c = ar2 , d = ar3 = ((𝑎𝑟^2 )(𝑎𝑟^3 ) + 𝑎(𝑎𝑟))/((𝑎𝑟^2 )(𝑎𝑟^3 ) − 𝑎(𝑎𝑟)) = (𝑎2𝑟4 + 𝑎2𝑟)/(𝑎2𝑟4 − 𝑎2𝑟) = (𝑎2𝑟4 + 𝑎2𝑟)/(𝑎2𝑟4 − 𝑎2𝑟) = (𝑎2𝑟(𝑟4 + 1))/(𝑎2𝑟(𝑟4 − 1 )) = (𝑟4 + 1 )/(𝑟4 − 1) So, (𝑞 + 𝑝)/(𝑞 − 𝑝) = (𝑟4 + 1 )/(𝑟4 − 1), we need to find r first. Now Dividing (1) & (3) (𝑎 + 𝑏)/(𝑐 + 𝑑) = 3/12 Putting values b = ar , c = ar2 , d = ar3 (𝑎 + 𝑎𝑟)/(𝑎𝑟2 +𝑎𝑟3) = 3/12 (𝑎(1 + 𝑟))/(𝑎𝑟2(1 + 𝑟)) = 3/12 1/𝑟2 = 3/12 1/𝑟2 = 1/4 r2 = 4 Now, (𝑞 + 𝑝)/(𝑞 − 𝑝) = (𝑟4 + 1 )/(𝑟4 − 1), Putting r2 = 4 = (4^2 + 1 )/(4^2 − 1) = (16 + 1)/(16 − 1) = (17 )/15 = R.H.S Thus, L.H.S = R.H.S Hence proved