Check sibling questions

Question 23 - NCERT Exemplar - MCQs - Chapter 5 Class 12 Continuity and Differentiability (Term 1)

Last updated at Nov. 18, 2021 by

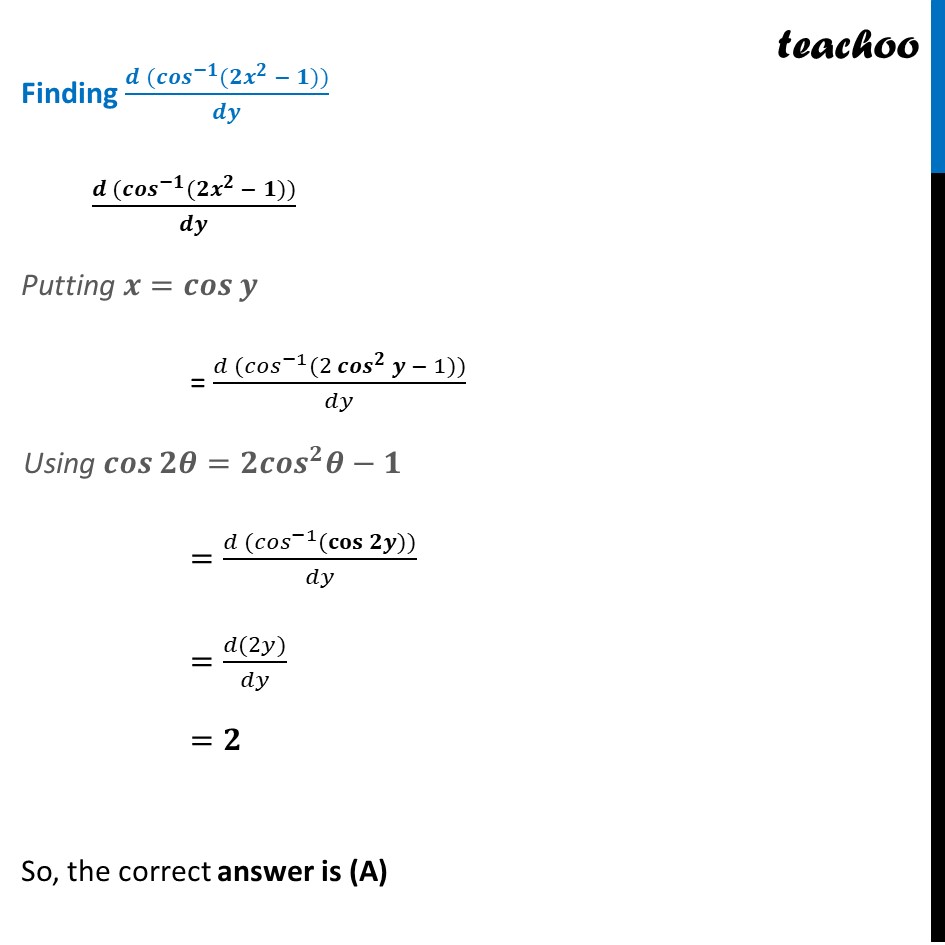
The derivative of cos –1 (2x 2 – 1) w.r.t. cos –1 x is

(A) 2 

(B) (-1)/(2√(1-x 2 ))

(C) 2/x 

(D) 1 − x 2

This question is similar to Question 24 - CBSE Class 12 - Sample Paper for 2022 Boards

Slide101.JPG

Slide102.JPG

Introducing your new favourite teacher - Teachoo Black, at only ₹83 per month

Join Teachoo Black

Transcript

Question 23 The derivative of cos–1 (2x2 – 1) w.r.t. cos–1x is (A) 2 (B) (−1)/(2√(1−𝑥^2 )) (C) 2/𝑥 (D) 1 − x2 Let 𝑦=〖𝑐𝑜𝑠〗^(−1)⁡𝑥 cos⁡〖𝑦=𝑥〗 〖𝒙=𝒄𝒐𝒔〗⁡𝒚 We need to find derivative of 〖𝑐𝑜𝑠〗^(−1)⁡〖(2𝑥−1)〗 w.r.t. 〖𝑐𝑜𝑠〗^(−1)⁡𝑥 i.e., 〖𝒄𝒐𝒔〗^(−𝟏)⁡〖(𝟐𝒙−𝟏)〗 w.r.t. 𝒚 i.e., (𝒅 〖(〖𝒄𝒐𝒔〗^(−𝟏)〗⁡〖(𝟐𝒙−𝟏)〗))/𝒅𝒚 Finding (𝒅 〖(〖𝒄𝒐𝒔〗^(−𝟏)〗⁡〖(𝟐𝒙^𝟐 − 𝟏)〗))/𝒅𝒚 (𝒅 〖(〖𝒄𝒐𝒔〗^(−𝟏)〗⁡〖(𝟐𝒙^𝟐 − 𝟏)〗))/𝒅𝒚 Putting 〖𝒙=𝒄𝒐𝒔〗⁡𝒚 = (𝑑 〖(〖𝑐𝑜𝑠〗^(−1)〗⁡〖(2 〖𝒄𝒐𝒔〗^𝟐⁡𝒚 − 1)〗))/𝑑𝑦 Using 𝒄𝒐𝒔⁡〖𝟐𝜽=𝟐〖𝒄𝒐𝒔〗^𝟐 𝜽−𝟏〗 =(𝑑 〖(〖𝑐𝑜𝑠〗^(−1)〗⁡〖(𝐜𝐨𝐬⁡𝟐𝒚)〗))/𝑑𝑦 =(𝑑(2𝑦))/𝑑𝑦 =𝟐 So, the correct answer is (A)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 12 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.