Question 20 - NCERT Exemplar - MCQs - Chapter 5 Class 12 Continuity and Differentiability

Last updated at Dec. 16, 2024 by Teachoo

If y = √(sin⁡〖x+y〗 ), then dy/dx is equal to

(A) cos⁡x/(2y-1)

(B) cos⁡x/(1-2y)

(C) sin⁡x/(1-2y)

(D) (-4x ³ )/(2y -1)

This question is similar to Example 25 - Chapter 5 Class 12 - Continuity and Differentiability

Next: Question 21 Important → Go Ad-free

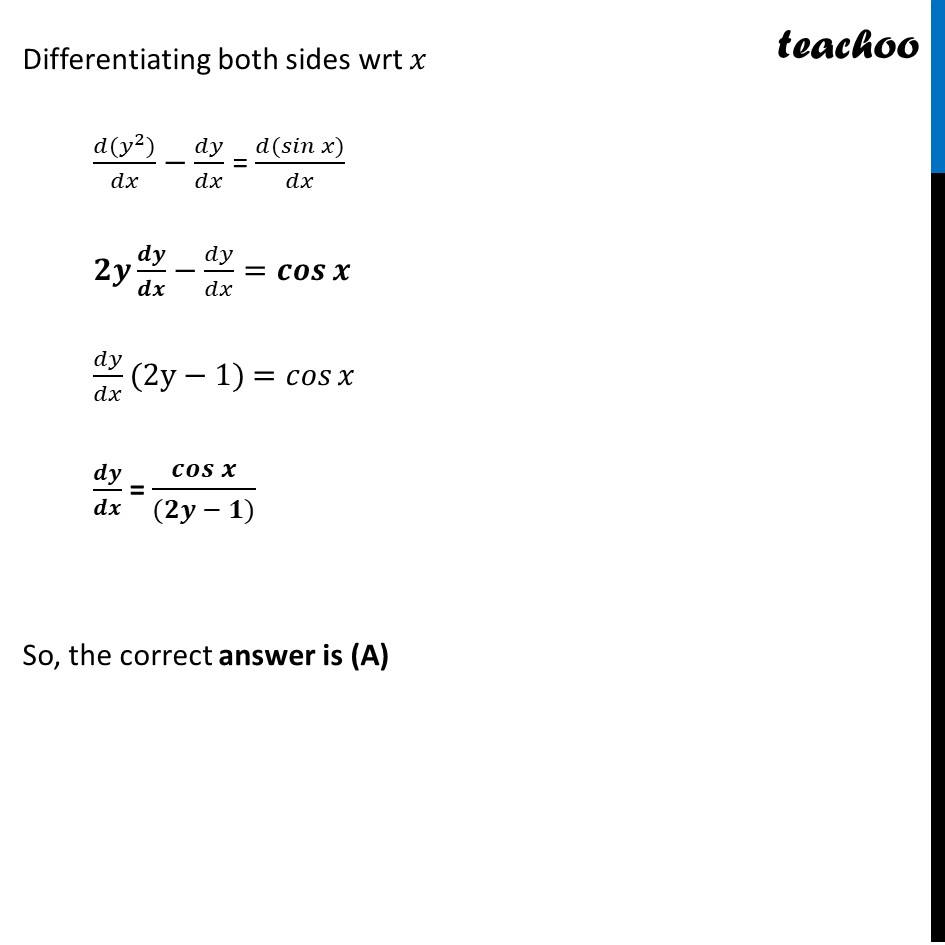
Transcript

Question 20 If y = √(sin⁡〖𝑥+𝑦〗 ), then 𝑑𝑦/𝑑𝑥 is equal to (A) cos⁡𝑥/(2𝑦−1) (B) cos⁡𝑥/(1−2𝑦) (C) sin⁡𝑥/(1−2𝑦) (D) (−4𝑥^3)/(2𝑦 −1) 𝑦=√(𝑠𝑖𝑛⁡〖𝑥+𝑦〗 ) Squaring both sides 𝑦^2=(√(sin⁡〖𝑥+𝑦〗 ))^2 𝒚^𝟐=𝒔𝒊𝒏⁡〖𝒙+𝒚〗 𝑦^2−𝑦=sin⁡𝑥 Differentiating both sides wrt 𝑥 (𝑑〖(𝑦〗^2))/𝑑𝑥−𝑑𝑦/𝑑𝑥 = (𝑑(𝑠𝑖𝑛⁡〖𝑥)〗)/𝑑𝑥 𝟐𝒚 𝒅𝒚/𝒅𝒙−𝑑𝑦/𝑑𝑥=𝒄𝒐𝒔⁡𝒙 𝑑𝑦/𝑑𝑥(2y−1) =𝑐𝑜𝑠⁡𝑥 𝒅𝒚/𝒅𝒙 = 𝒄𝒐𝒔⁡𝒙/((𝟐𝒚 − 𝟏)) So, the correct answer is (A)

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular