Ex 1.1, 10 (ii) - Chapter 1 Class 12 Relation and Functions
Last updated at April 16, 2024 by Teachoo
Ex 1.1
Ex 1.1, 1 (i)
Ex 1.1, 1 (ii)
Ex 1.1, 1 (iii) Important
Ex 1.1, 1 (iv)
Ex 1.1, 1 (v)
Ex 1.1, 2
Ex 1.1, 3
Ex 1.1, 4
Ex 1.1, 5 Important
Ex 1.1, 6
Ex 1.1, 7
Ex 1.1, 8
Ex 1.1, 9 (i)
Ex 1.1, 9 (ii)
Ex 1.1, 10 (i)
Ex 1.1, 10 (ii) You are here
Ex 1.1, 10 (iii) Important
Ex 1.1, 10 (iv)
Ex 1.1, 10 (v)
Ex 1.1, 11
Ex 1.1, 12 Important
Ex 1.1, 13
Ex 1.1, 14
Ex 1.1, 15 (MCQ) Important
Ex 1.1, 16 (MCQ)
Transcript
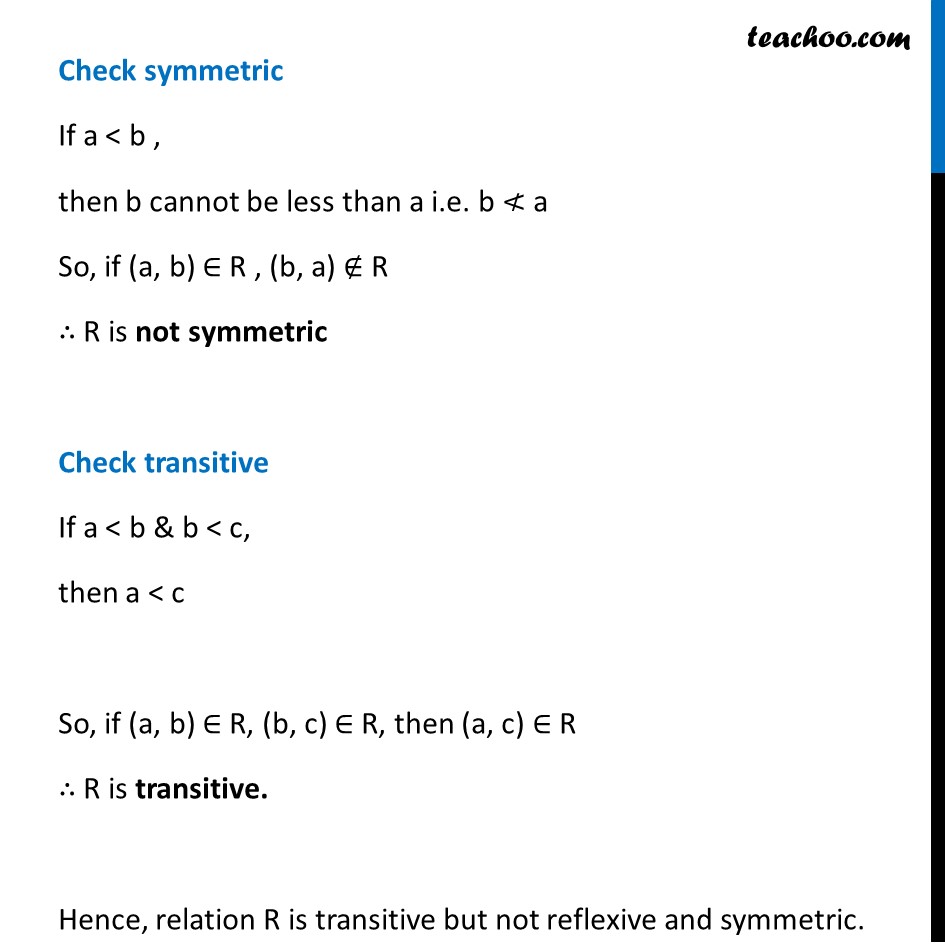
Ex 1.1, 10 Given an example of a relation. Which is (ii) Transitive but neither reflexive nor symmetric. Let R = {(a, b): a < b} Check reflexive Since a cannot be less than a a ≮ a So, (a, a) ∉ R ∴ R is not reflexive. Check symmetric If a < b , then b cannot be less than a i.e. b ≮ a So, if (a, b) ∈ R , (b, a) ∉ R ∴ R is not symmetric Check transitive If a < b & b < c, then a < c So, if (a, b) ∈ R, (b, c) ∈ R, then (a, c) ∈ R ∴ R is transitive. Hence, relation R is transitive but not reflexive and symmetric.
