Ex 1.1, 1 (v) - Chapter 1 Class 12 Relation and Functions

Transcript

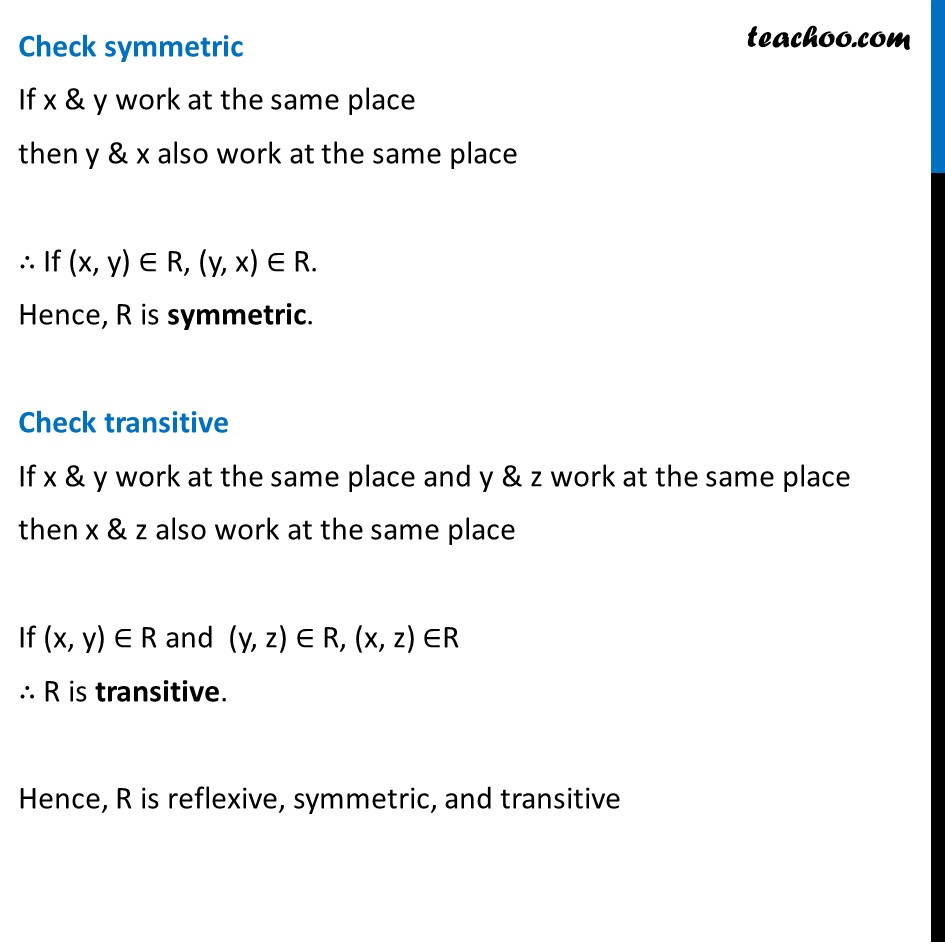
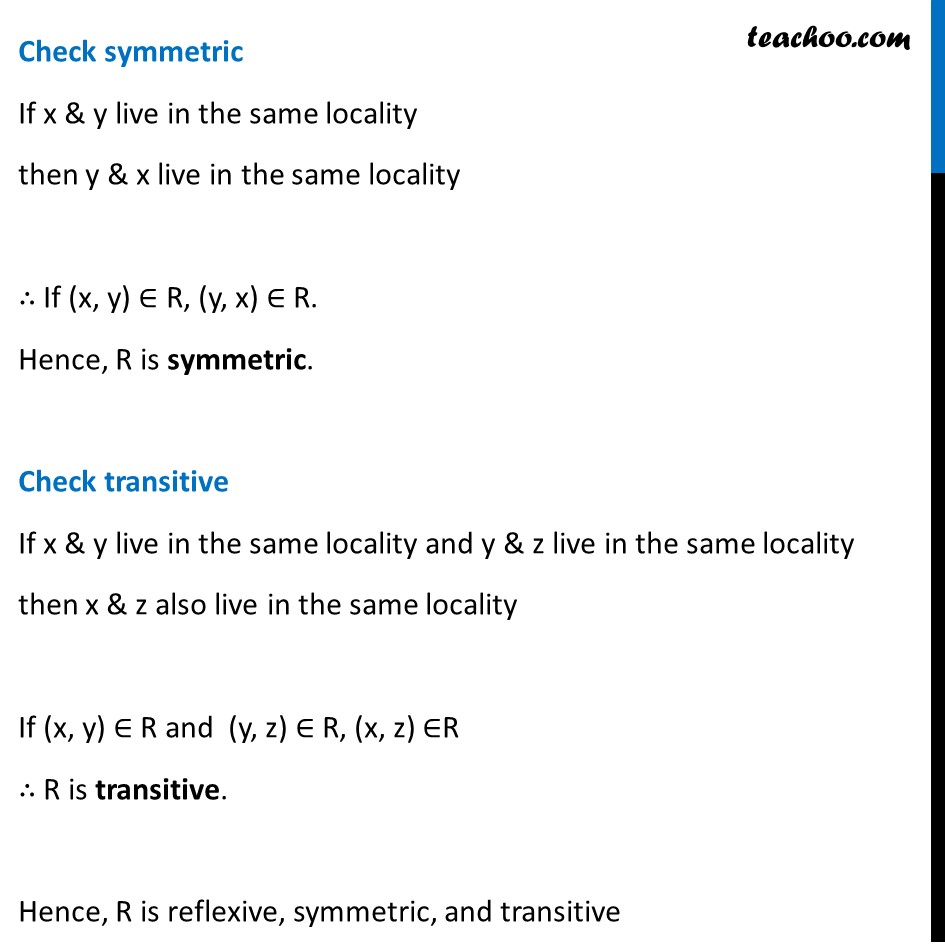
Ex 1.1, 1 (v) Relation R in the set A of human beings in a town at a particular time given by (a) R = {(x, y): x and y work at the same place} R = {(x, y): x and y work at the same place} Check reflexive Since x & x are the same person, they work at the same place So, (x, x) ∈ R ∴ R is reflexive. Check symmetric If x & y work at the same place then y & x also work at the same place ∴ If (x, y) ∈ R, (y, x) ∈ R. Hence, R is symmetric. Check transitive If x & y work at the same place and y & z work at the same place then x & z also work at the same place If (x, y) ∈ R and (y, z) ∈ R, (x, z) ∈R ∴ R is transitive. Hence, R is reflexive, symmetric, and transitive Ex 1.1, 1(v) (b) R = {(x, y): x and y live in the same locality} R = {(x, y): x and y live in the same locality} Check reflexive Since x & x are the same person, they live in the same locality So, (x, x) ∈ R ∴ R is reflexive. Check symmetric If x & y live in the same locality then y & x live in the same locality ∴ If (x, y) ∈ R, (y, x) ∈ R. Hence, R is symmetric. Check transitive If x & y live in the same locality and y & z live in the same locality then x & z also live in the same locality If (x, y) ∈ R and (y, z) ∈ R, (x, z) ∈R ∴ R is transitive. Hence, R is reflexive, symmetric, and transitive Ex 1.1,1(v) (c) R = {(x, y): x is exactly 7 cm taller than y} R = {(x, y): x is exactly 7 cm taller than y} Check reflexive Since x & x are the same person, he cannot be taller than himself ⇒ (x, x) ∉ R ∴R is not reflexive. Check symmetric If x is exactly 7 cm taller than y. then, y is not taller than x. So, if (x, y) ∈ R , (y, x) ∉ R ∴ R is not symmetric. Check transitive If x is exactly 7 cm taller than y and y is exactly 7 cm taller than z. ⇒ x is exactly 14 cm taller than z . So, x is not 7 cm taller than z So, If (x, y) ∈ R & (y, z) ∈ R, then(x, z) ∉R ∴ R is not transitive. Hence, R is neither reflexive, nor symmetric, nor transitive. Ex 1.1,1(v) (d) R = {(x, y): x is wife of y} R = {(x, y): x is wife of y} Check reflexive Since x & x are the same person, she cannot be the wife of herself ⇒ (x, x) ∉ R ∴ R is not reflexive. Check symmetric If x is the wife of y. then, y cannot be the wife of x. So, if (x, y) ∈ R , (y, x) ∉ R ∴ R is not symmetric. Check transitive If x is the wife of y then y cannot be the wife of anybody else So, if (x, y) ∈ R & (y, z) ∉ R, ∴ R is not transitive. Hence, R is neither reflexive, nor symmetric, nor transitive. Ex 1.1, 1 (v) (e) R = {(x, y): x is father of y} R = {(x, y): x is father of y} Check reflexive Since x & x are the same person, he cannot be the father of herself ⇒ (x, x) ∉ R ∴ R is not reflexive. Check symmetric If x is the father of y. then, y cannot be the father of x. So, if (x, y) ∈ R , (y, x) ∉ R ∴ R is not symmetric. Check transitive If x is the father of y , & y is the father of z then x cannot be the father of z (he is the grandfather) So, if (x, y) ∈ R and (y, z) ∈ R. then (x, z) ∉ R ∴ R is not transitive. Hence, R is neither reflexive, nor symmetric, nor transitive.