Ex 6.2,19 (MCQ) - Chapter 6 Class 12 Application of Derivatives (Term 1)

Last updated at Aug. 9, 2021 by Teachoo

Ex 6.2, 19 - The interval in which y = x2 e-x is increasing

Ex 6.2,19 - Chapter 6 Class 12 Application of Derivatives - Part 2

Ex 6.2,19 - Chapter 6 Class 12 Application of Derivatives - Part 3

Transcript

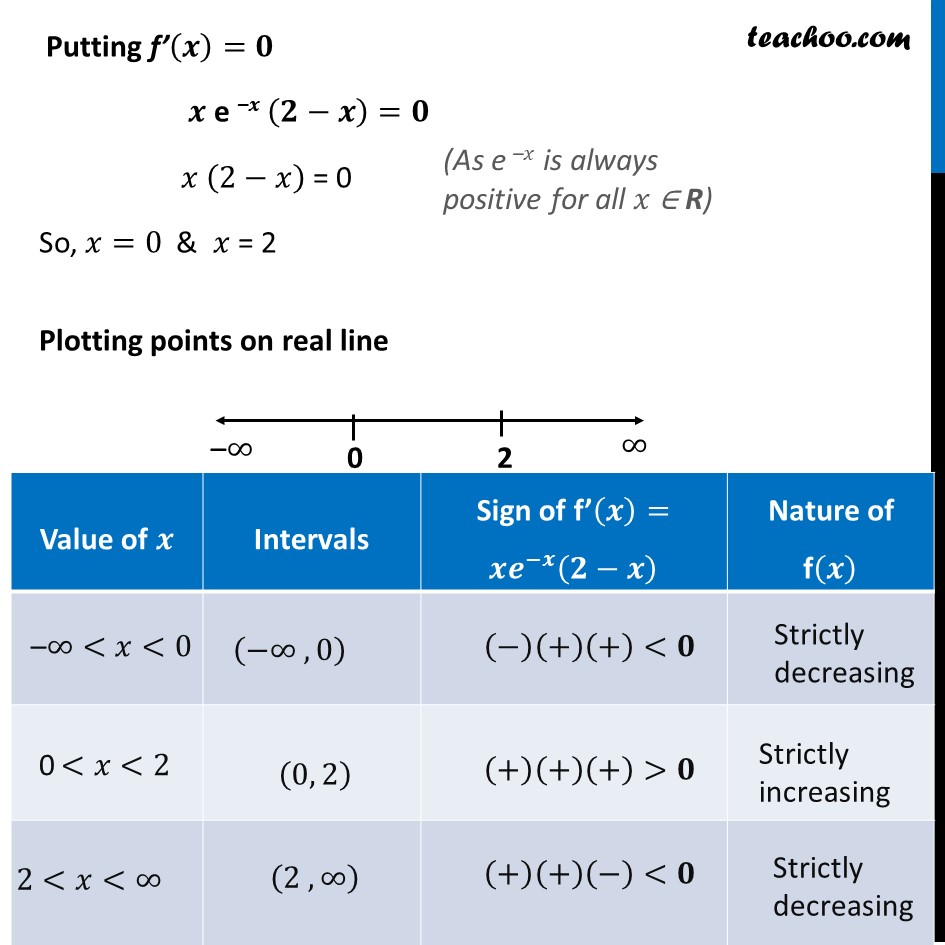
Ex 6.2, 19 The interval in which 𝑦 = 𝑥2 𝑒^(–𝑥) is increasing is (A) (– ∞, ∞) (B) (– 2, 0) (C) (2, ∞) (D) (0, 2)Let f(𝑥) = 𝑥^2 𝑒^(−𝑥) Finding f’(𝒙) f’(𝑥) = (𝑥^2 𝑒^(−𝑥) )′ Using product rule f’(𝑥) = (𝑥2)′ 𝑒^(−𝑥) + (𝑒^(−𝑥) )’ (𝑥2) f’(𝑥) = (2𝑥) 𝑒^(−𝑥) + (〖−𝑒〗^(−𝑥) ) (𝑥2) f’(𝑥) = 2𝑥 𝑒^(−𝑥)−𝑒^(−𝑥) 𝑥2 f’(𝒙) = 𝒙 e –𝒙 (𝟐−𝒙) Putting f’(𝒙)=𝟎 𝒙 e –𝒙 (𝟐−𝒙)=𝟎 𝑥 (2−𝑥) = 0 So, 𝑥=0 & 𝑥 = 2 Plotting points on real line (As e –𝑥 is always positive for all 𝑥 ∈ R) Hence, f(𝑥) is strictly increasing on (0 , 2) Therefore, correct answer is (𝐃)

Ex 6.2

Ex 6.2,19 (MCQ) Important You are here

Ex 6.3 →

Chapter 6 Class 12 Application of Derivatives (Term 1)

Serial order wise

About the Author

Davneet Singh

Davneet Singh is a graduate from Indian Institute of Technology, Kanpur. He has been teaching from the past 10 years. He provides courses for Maths and Science at Teachoo.