Ex 6.2,15 - Chapter 6 Class 12 Application of Derivatives

Last updated at Dec. 16, 2024 by

Ex 6.2, 15 - Let I be any interval disjoint from [–1, 1]. Prove

Ex 6.2,15 - Chapter 6 Class 12 Application of Derivatives - Part 2
Ex 6.2,15 - Chapter 6 Class 12 Application of Derivatives - Part 3

Remove Ads

Transcript

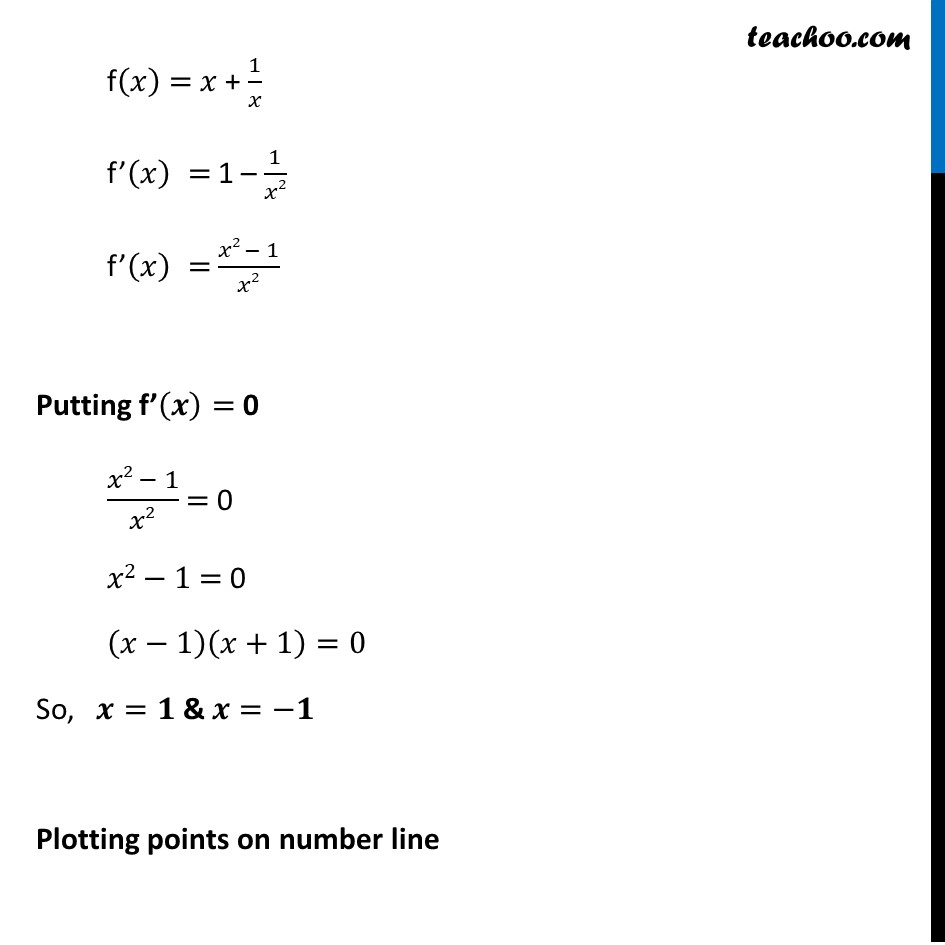
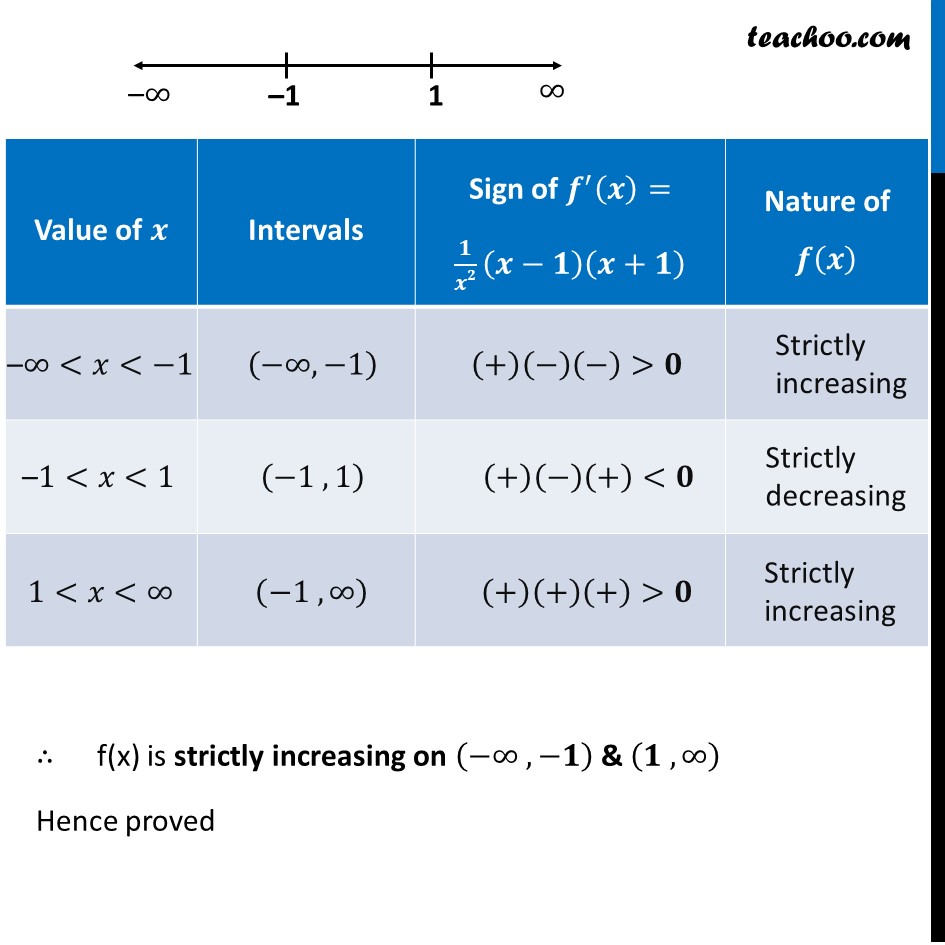
Ex 6.2, 15 Let I be any interval disjoint from [–1, 1]. Prove that the function f given by 𝑓(𝑥) = 𝑥 + 1/𝑥 is strictly increasing on I.I is any interval disjoint from [–1, 1] Let I = (−∞, −𝟏)∪(𝟏, ∞) Given f(𝑥) = 𝑥 + 1/𝑥 We need to show f(𝑥) is strictly increasing on I i.e. we need to show f’(𝒙) > 0 for 𝑥 ∈ (−∞, −𝟏)∪(𝟏, ∞) Finding f’(𝒙) f(𝑥) = 𝑥 + 1/𝑥 f’(𝑥) = 1 – 1/𝑥2 f’(𝑥) = (𝑥2 − 1)/𝑥2 Putting f’(𝒙) = 0 (𝑥2 − 1)/𝑥2 = 0 𝑥2−1 = 0 (𝑥−1)(𝑥+1)=0 So, 𝒙=𝟏 & 𝒙=−𝟏 Plotting points on number line The point 𝑥 = –1 , 1 into three disjoint intervals ∴ f(x) is strictly increasing on (−∞ , −𝟏) & (𝟏 , ∞) Hence proved

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo