Check sibling questions

Ex 6.2,14 - Chapter 6 Class 12 Application of Derivatives

Last updated at May 29, 2023 by

Ex 6.2, 14 - Find least value of a such that f(x) = x2+ax+1

Ex 6.2,14 - Chapter 6 Class 12 Application of Derivatives - Part 2

Learn in your speed, with individual attention - Teachoo Maths 1-on-1 Class

Book a free demo

Transcript

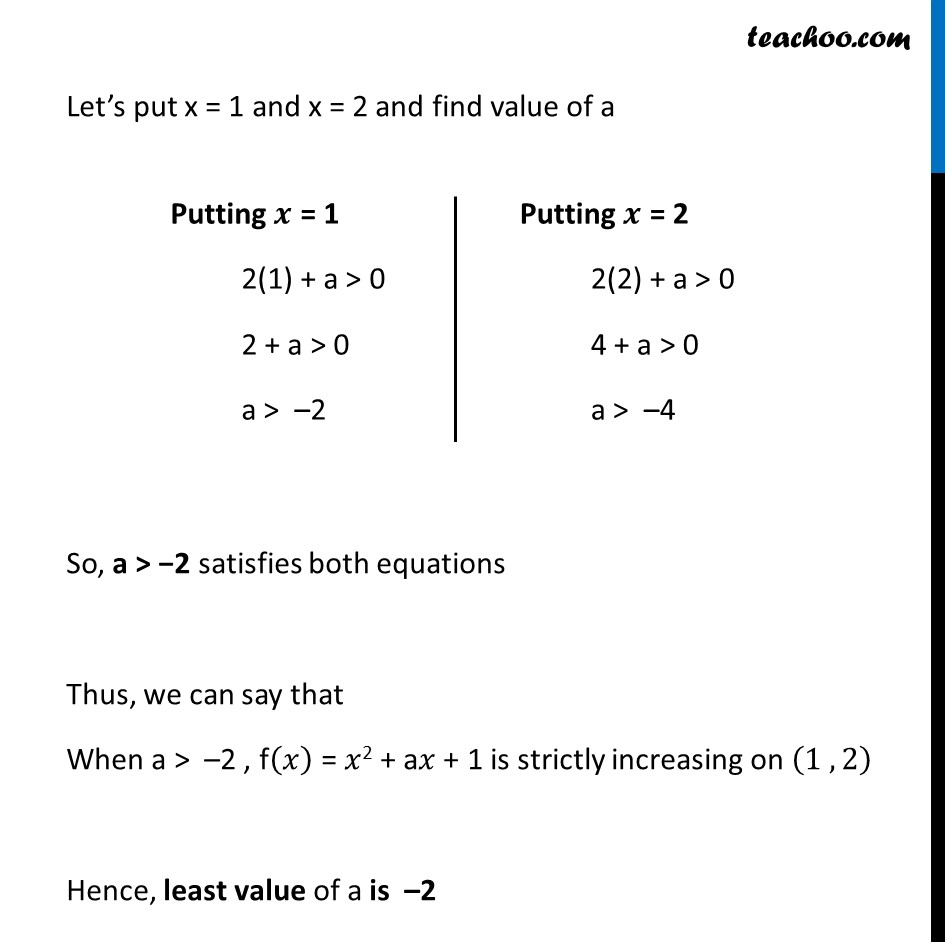
Ex 6.2, 14 Find the least value of a such that the function f given by 𝑓 (𝑥) = 𝑥2 + 𝑎𝑥 + 1 is strictly increasing on (1, 2).We have f(𝑥) = 𝑥2 + a𝑥 + 1 And, f’(𝑥) = 2𝑥 + a Given f is strictly increasing on (1 ,2) ∴ f’(𝑥) > 0 on (1 ,2) Putting value of f’(x) 2𝑥 + a > 0 on (1 ,2) Let’s put x = 1 and x = 2 and find value of a So, a > −2 satisfies both equations Thus, we can say that When a > –2 , f(𝑥) = 𝑥2 + a𝑥 + 1 is strictly increasing on (1 , 2) Hence, least value of a is –2 Putting 𝒙 = 1 2(1) + a > 0 2 + a > 0 a > –2 Putting 𝒙 = 2 2(2) + a > 0 4 + a > 0 a > –4

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 13 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.