Example 7 - Chapter 13 Class 12 Probability (Important Question)

Last updated at Dec. 16, 2024 by Teachoo

Next: Example 6 → Go Ad-free

Transcript

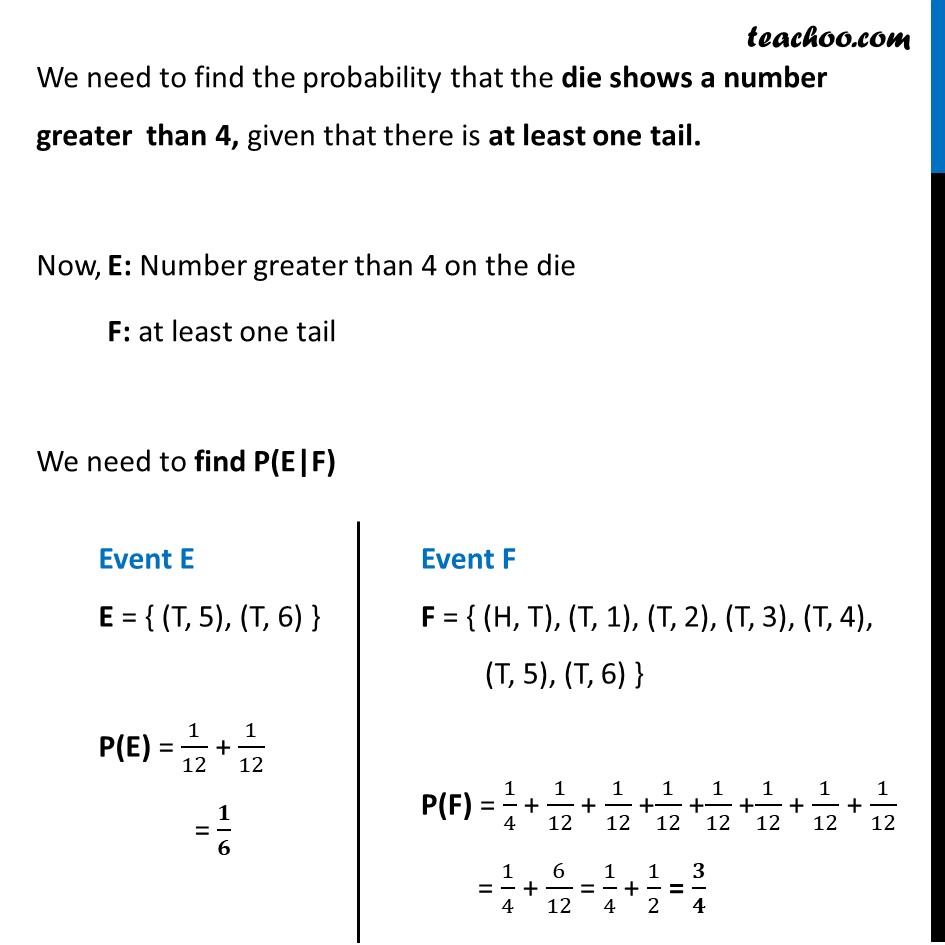
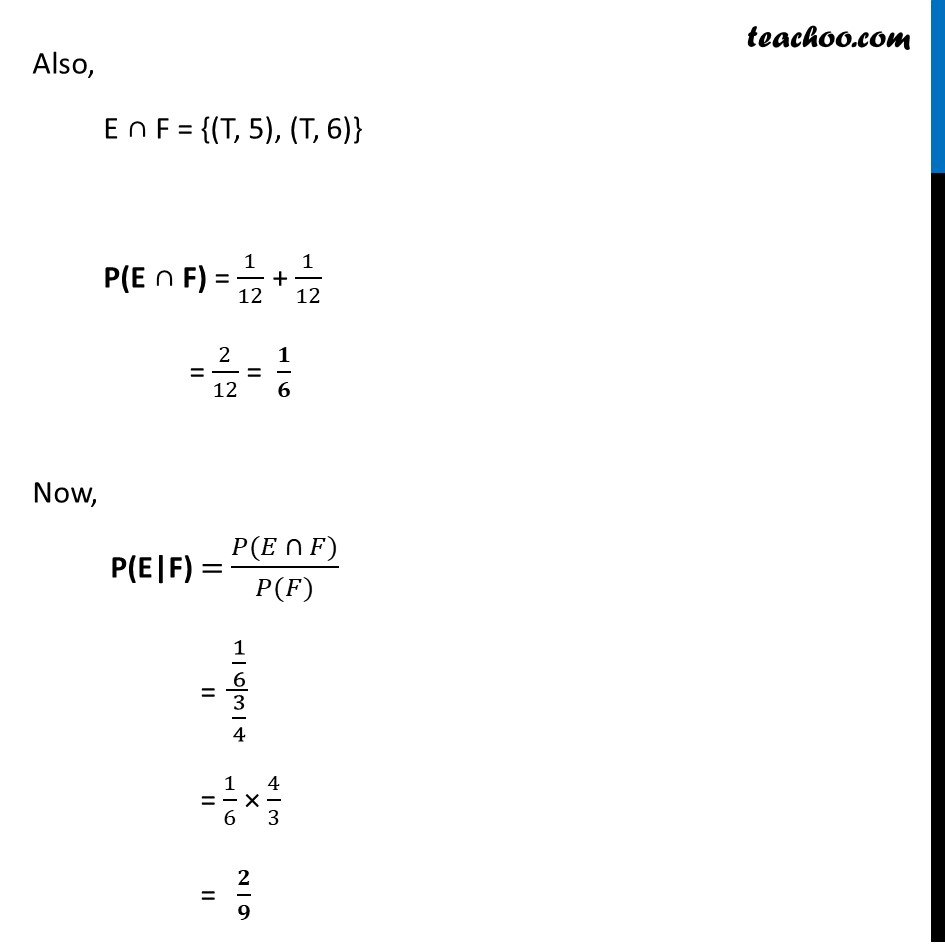
Example 7 Consider the experiment of tossing a coin. If the coin shows head, toss it again but if it shows tail, then throw a die. Find the conditional probability of the event that ‘the die shows a number greater than 4’ given that ‘there is at least one tail’.sLet’s Make a Tree Diagram We need to find the probability that the die shows a number greater than 4, given that there is at least one tail. Now, E: Number greater than 4 on the die F: at least one tail We need to find P(E|F) Event E E = { (T, 5), (T, 6) } P(E) = 1/12 + 1/12 = 𝟏/𝟔 Event F F = { (H, T), (T, 1), (T, 2), (T, 3), (T, 4), (T, 5), (T, 6) } P(F) = 1/4 + 1/12 + 1/12 +1/12 +1/12 +1/12 + 1/12 + 1/12 = 1/4 + 6/12 = 1/4 + 1/2 = 𝟑/𝟒 Also, E ∩ F = {(T, 5), (T, 6)} P(E ∩ F) = 1/12 + 1/12 = 2/12 = 𝟏/𝟔 Now, P(E|F) = (𝑃(𝐸 ∩ 𝐹))/(𝑃(𝐹)) = (1/6)/(3/4) = 1/6 × 4/3 = 𝟐/𝟗

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular