Question 6 (a) - Plane - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

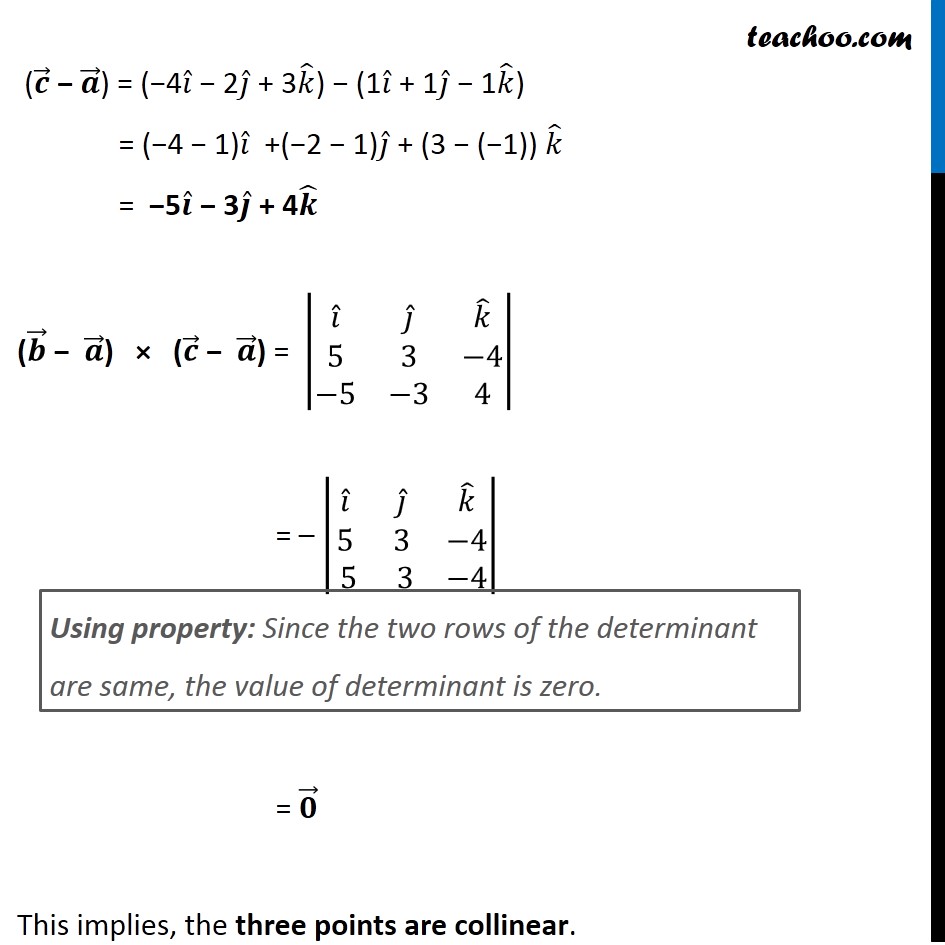
Question 6 (Introduction) Find the equations of the planes that passes through three points. (a) (1, 1, – 1), (6, 4, – 5), (– 4, – 2, 3) Vector equation of a plane passing through three points with position vectors 𝑎 ⃗, 𝑏 ⃗, 𝑐 ⃗ is ("r" ⃗ − 𝑎 ⃗) . [(𝑏 ⃗−𝑎 ⃗)×(𝑐 ⃗−𝑎 ⃗)] = 0 Question 6 Find the equations of the planes that passes through three points. (a) (1, 1, –1), (6, 4, –5), (–4, –2, 3) Vector equation of a plane passing through three points with position vectors 𝑎 ⃗, 𝑏 ⃗, 𝑐 ⃗ is ("r" ⃗ − 𝒂 ⃗) . [(𝒃 ⃗−𝒂 ⃗)×(𝒄 ⃗−𝒂 ⃗)] = 0 Now, the plane passes through the points (𝒃 ⃗ − 𝒂 ⃗) = (6𝑖 ̂ + 4𝑗 ̂ – 5𝑘 ̂) − (1𝑖 ̂ + 1𝑗 ̂ − 1𝑘 ̂) = (6 −1)𝑖 ̂ + (4 − 1)𝑗 ̂ + (−5 − (−1)) 𝑘 ̂ = 5𝒊 ̂ + 3𝒋 ̂ − 4𝒌 ̂ A (1, 1, −1) 𝑎 ⃗ = 1𝑖 ̂ + 1𝑗 ̂ − 1𝑘 ̂ B (6, 4, −5) 𝑏 ⃗ = 6𝑖 ̂ + 4𝑗 ̂ − 5𝑘 ̂ C ( −4, −2, 3) 𝑐 ⃗ = −4𝑖 ̂ − 2𝑗 ̂ + 3𝑘 ̂ (𝒄 ⃗ − 𝒂 ⃗) = (−4𝑖 ̂ − 2𝑗 ̂ + 3𝑘 ̂) − (1𝑖 ̂ + 1𝑗 ̂ − 1𝑘 ̂) = (−4 − 1)𝑖 ̂ +(−2 − 1)𝑗 ̂ + (3 − (−1)) 𝑘 ̂ = −5𝒊 ̂ − 3𝒋 ̂ + 4𝒌 ̂ (𝒃 ⃗ − 𝒂 ⃗) × (𝒄 ⃗ − 𝒂 ⃗) = |■8(𝑖 ̂&𝑗 ̂&𝑘 ̂@5&3&−4@−5&−3&4)| = – |■8(𝑖 ̂&𝑗 ̂&𝑘 ̂@5&3&−4@ 5& 3&−4)| = 𝟎 ⃗ This implies, the three points are collinear. Using property: Since the two rows of the determinant are same, the value of determinant is zero. ∴ Vector equation of plane is [𝑟 ⃗−(𝑖 ̂+𝑗 ̂ −𝑘 ̂ )] . 0 ⃗ = 0 Since, the above equation is satisfied for all values of 𝑟 ⃗, Therefore, there will be infinite planes passing through the given 3 collinear points.