Question 13 (c) - Plane - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

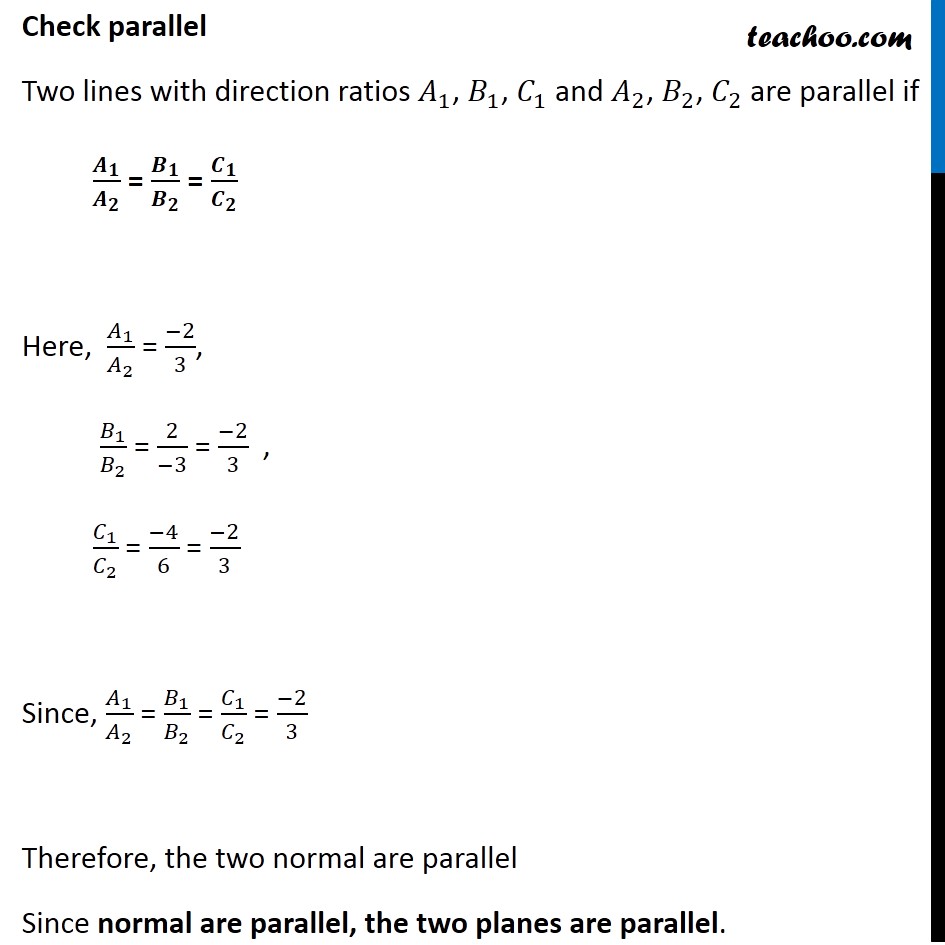
Question 13 In the following cases, determine whether the given planes are parallel or perpendicular, and in case they are neither, find the angles between them. (c) 2x – 2y + 4z + 5 = 0 and 3x – 3y + 6z – 1 = 0 2x − 2y + 4z + 5 = 0 2x − 2y + 4z = −5 −2x + 2y − 4z = 5 Comparing with A1x + B1y + C1z = d1 Direction ratios of normal = –2, 2, –4 A1 = –2 , B1 = 2 , C1 = –4 3x − 3y + 6z − 1 = 0 3x − 3y + 6z = 1 Comparing with A2x + B2y + C2z = d2 Direction ratios of normal = 3, –3, 6 A2 = 3 , B2 = –3 , C2 = 6 Check parallel Two lines with direction ratios 𝐴_1, 𝐵_1, 𝐶_1 and 𝐴_2, 𝐵_2, 𝐶_2 are parallel if 𝑨_𝟏/𝑨_𝟐 = 𝑩_𝟏/𝑩_𝟐 = 𝑪_𝟏/𝑪_𝟐 Here, 𝐴_1/𝐴_2 = (−2)/3, 𝐵_1/𝐵_2 = 2/(−3) = (−2)/3 , 𝐶_1/𝐶_2 = (−4)/6 = (−2)/3 Since, 𝐴_1/𝐴_2 = 𝐵_1/𝐵_2 = 𝐶_1/𝐶_2 = (−2)/3 Therefore, the two normal are parallel Since normal are parallel, the two planes are parallel.