Question 3 - Definite Integral as a limit of a sum - Chapter 7 Class 12 Integrals

Transcript

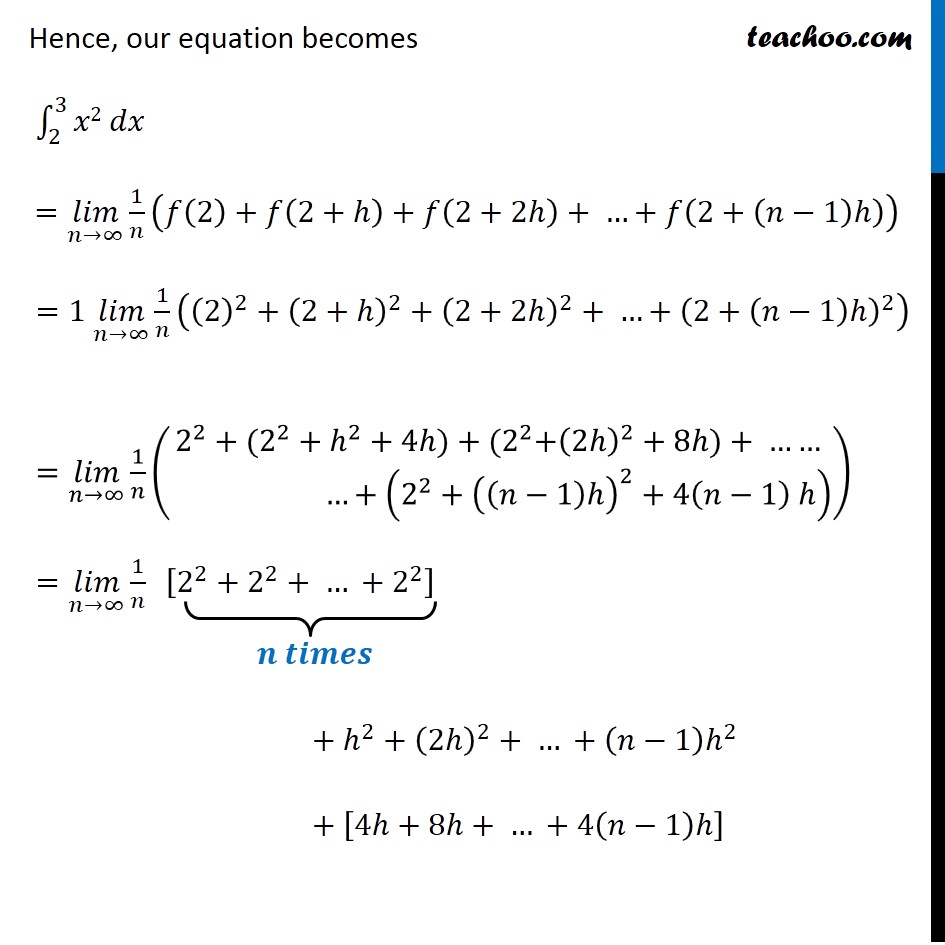
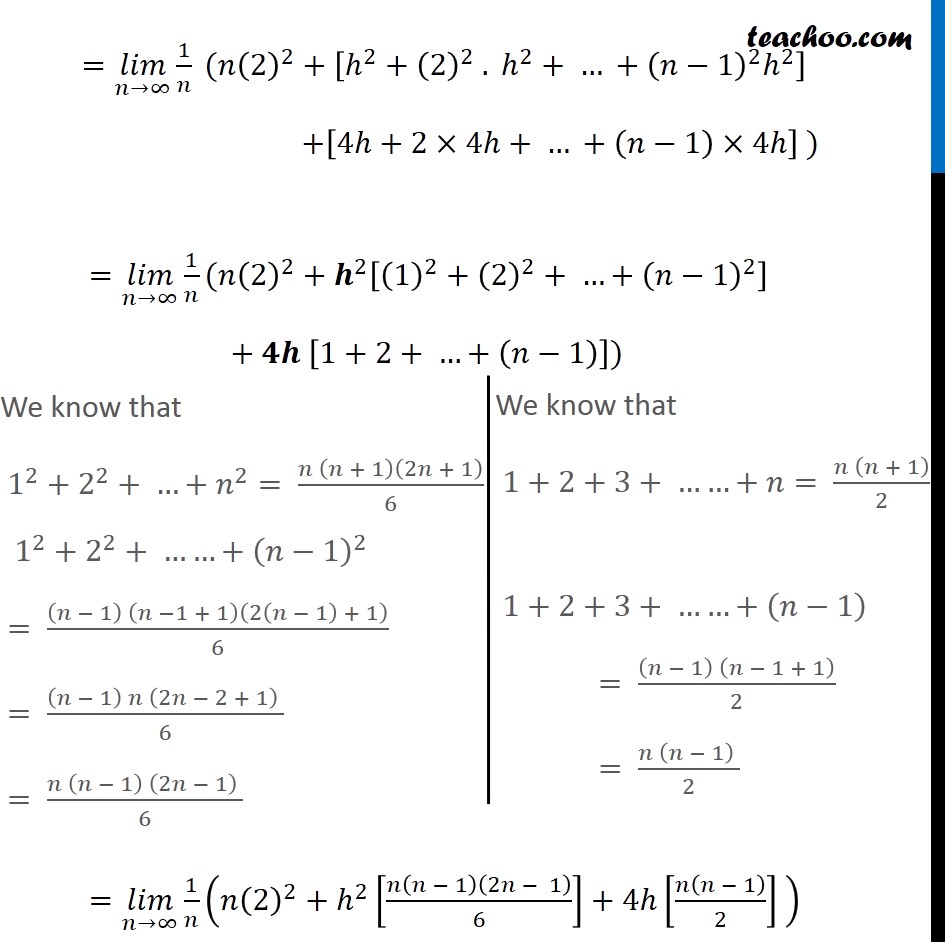
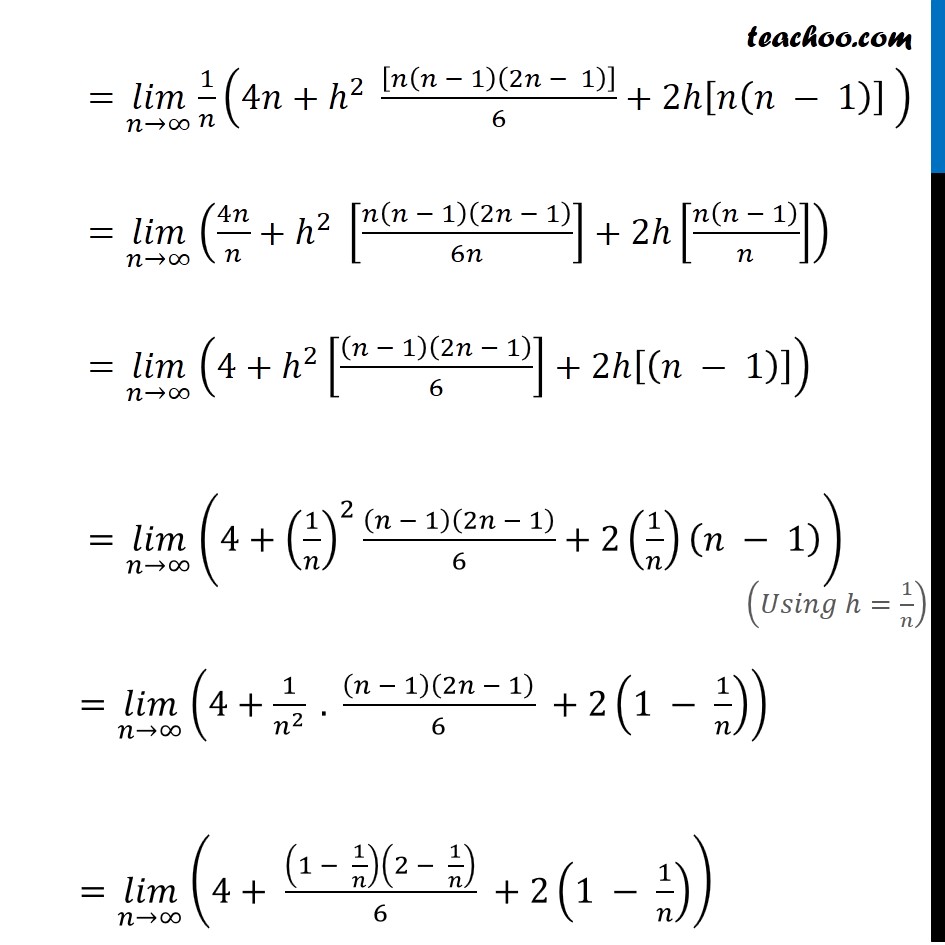
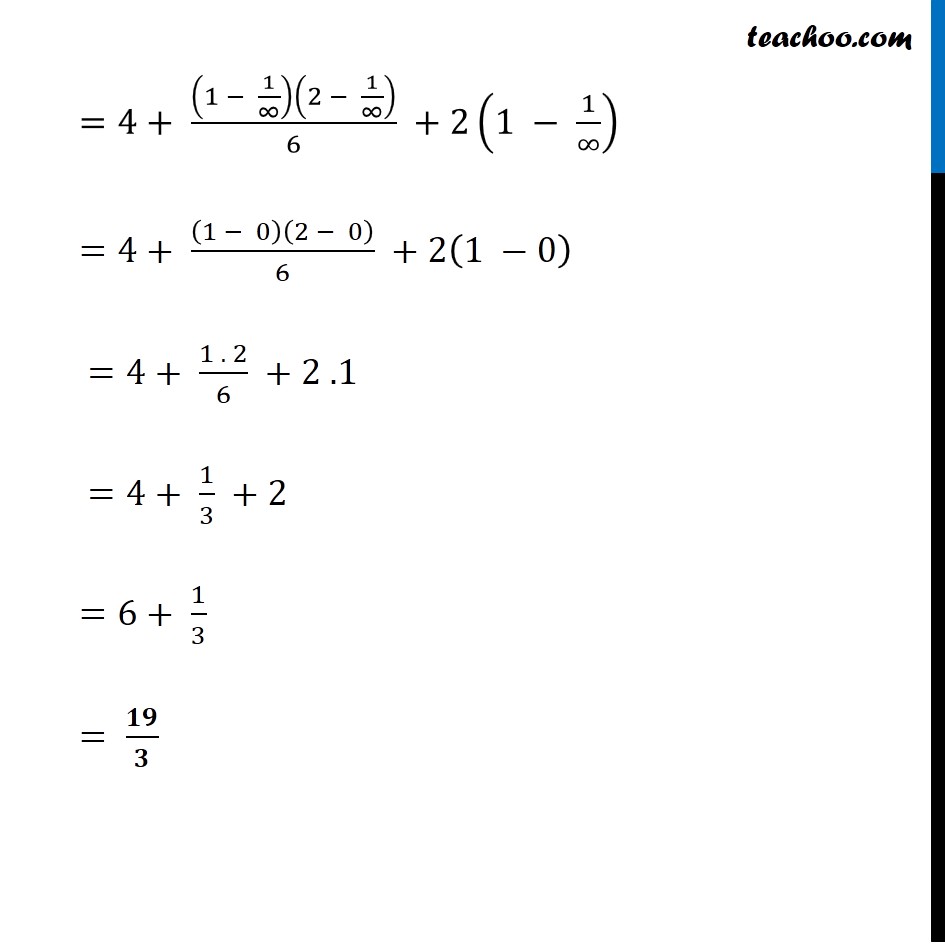
Question 3 ∫1_2^3▒〖𝑥2 𝑑𝑥〗 ∫1_2^3▒〖𝑥2 𝑑𝑥〗 Putting 𝑎 =2 𝑏 =3 ℎ=(𝑏 − 𝑎)/𝑛 =(3 − 2)/𝑛 =1/𝑛 𝑓(𝑥)=𝑥^2 We know that ∫1_𝑎^𝑏▒〖𝑥 𝑑𝑥〗 =(𝑏−𝑎) (𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 (𝑓(𝑎)+𝑓(𝑎+ℎ)+𝑓(𝑎+2ℎ)…+𝑓(𝑎+(𝑛−1)ℎ)) Hence we can write ∫1_2^3▒〖𝑥2 𝑑𝑥〗 =(3−2) (𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 (𝑓(2)+𝑓(2+ℎ)+𝑓(2+2ℎ)+ …+𝑓(2+(𝑛−1)ℎ)) =(𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 (𝑓(2)+𝑓(2+ℎ)+𝑓(2+2ℎ)+ …+𝑓(2+(𝑛−1)ℎ)) Here, 𝑓(𝑥)=𝑥^2 𝑓(2)=(2)^2=4 𝑓(2+ℎ)=(2+ℎ)^2 𝑓 (2+2ℎ)=(2+2ℎ)^2 … 𝑓(2+(𝑛−1)ℎ)=(2+(𝑛−1)ℎ)^2 Hence, our equation becomes ∫1_2^3▒〖𝑥2 𝑑𝑥〗 " " =(𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 (𝑓(2)+𝑓(2+ℎ)+𝑓(2+2ℎ)+ …+𝑓(2+(𝑛−1)ℎ)) =1 (𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 ((2)^2+(2+ℎ)^2+(2+2ℎ)^2+ …+(2+(𝑛−1)ℎ)^2 ) =(𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 (█(2^2+(2^2+ℎ^2+4ℎ)+〖(2〗^2+(2ℎ)^2+8ℎ)+ …… @ …+(2^2+((𝑛−1)ℎ)^2+4(𝑛−1) ℎ) )) =(𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 [2^2+2^2+ … +2^2 ] + ℎ^2+(2ℎ)^2+ … +(𝑛−1)ℎ^2 + [4ℎ+8ℎ+ … +4(𝑛−1)ℎ] =(𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 (〖𝑛(2)〗^2+[ℎ^2+(2)^2 . ℎ^2+ … +(𝑛−1)^2 ℎ^2 ] +[4ℎ+2×4ℎ+ … +(𝑛−1)×4ℎ] ) =(𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛(𝑛(2)^2+𝒉^2 [(1)^2+(2)^2+ …+(𝑛−1)^2 ] + 𝟒𝒉 [1+2+ …+(𝑛−1)]) =(𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 (𝑛(2)^2+ℎ^2 [𝑛(𝑛 − 1)(2𝑛 − 1)/6]+4ℎ[𝑛(𝑛 − 1)/2] ) We know that 1^2+2^2+ …+𝑛^2= (𝑛 (𝑛 + 1)(2𝑛 + 1))/6 1^2+2^2+ ……+(𝑛−1)^2 = ((𝑛 − 1) (𝑛 −1 + 1)(2(𝑛 − 1) + 1))/6 = ((𝑛 − 1) 𝑛 (2𝑛 − 2 + 1) )/6 = (𝑛 (𝑛 − 1) (2𝑛 − 1) )/6 We know that 1+2+3+ ……+𝑛= (𝑛 (𝑛 + 1))/2 1+2+3+ ……+(𝑛−1) = ((𝑛 − 1) (𝑛 − 1 + 1))/2 = (𝑛 (𝑛 − 1) )/2 =(𝑙𝑖𝑚)┬(𝑛→∞) 1/𝑛 (4𝑛+ℎ^2 [𝑛(𝑛 − 1)(2𝑛 − 1)]/6+2ℎ[𝑛(𝑛 − 1)] ) =(𝑙𝑖𝑚)┬(𝑛→∞) (4𝑛/𝑛+ℎ^2 [𝑛(𝑛 − 1)(2𝑛 − 1)/6𝑛]+2ℎ[𝑛(𝑛 − 1)/𝑛]) =(𝑙𝑖𝑚)┬(𝑛→∞) (4+ℎ^2 [(𝑛 − 1)(2𝑛 − 1)/6]+2ℎ[(𝑛 − 1)]) =(𝑙𝑖𝑚)┬(𝑛→∞) (4+(1/𝑛)^2 (𝑛 − 1)(2𝑛 − 1)/6+2(1/𝑛)(𝑛 − 1)) =(𝑙𝑖𝑚)┬(𝑛→∞) (4+1/𝑛^2 . (𝑛 − 1)(2𝑛 − 1)/6 +2(1 − 1/𝑛)) =(𝑙𝑖𝑚)┬(𝑛→∞) (4+ (1 − 1/𝑛)(2 − 1/𝑛)/6 +2(1 − 1/𝑛)) =4+ (1 − 1/∞)(2 − 1/∞)/6 +2(1 − 1/∞) =4+ (1 − 0)(2 − 0)/6 +2(1 −0) =4+ (1 . 2)/6 +2 .1 =4+ 1/3 +2 =6+ 1/3 = 𝟏𝟗/𝟑