Misc 22 - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

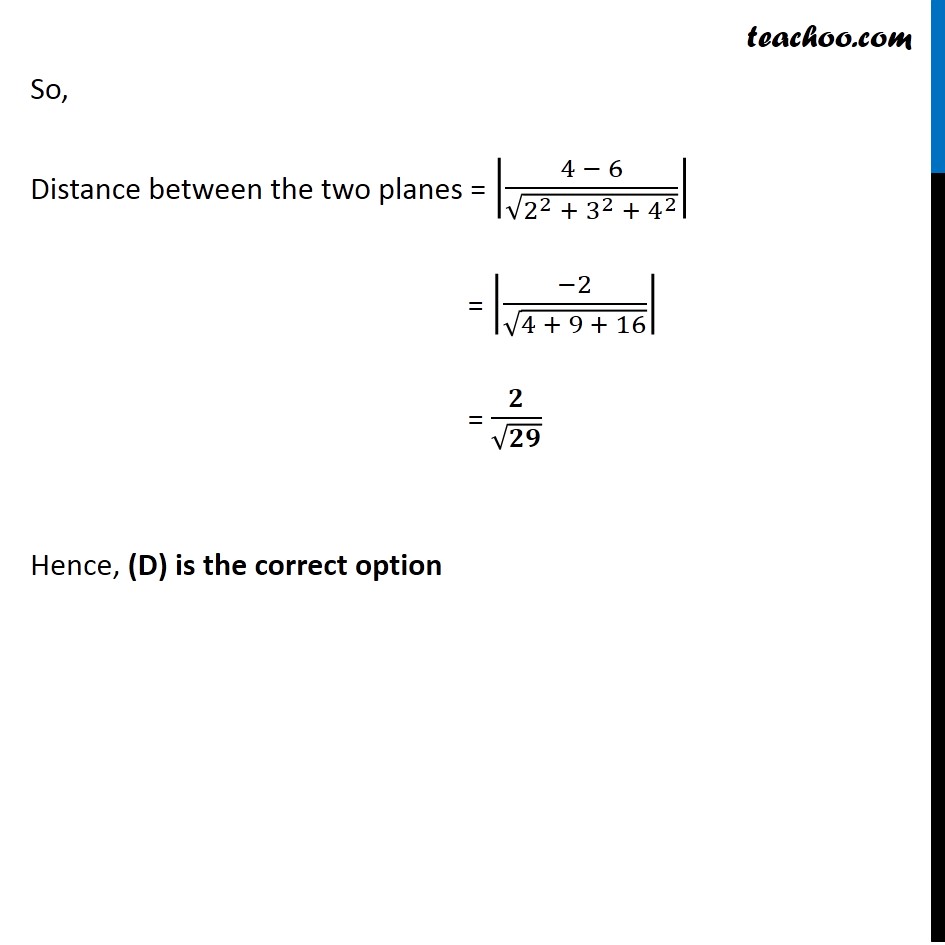
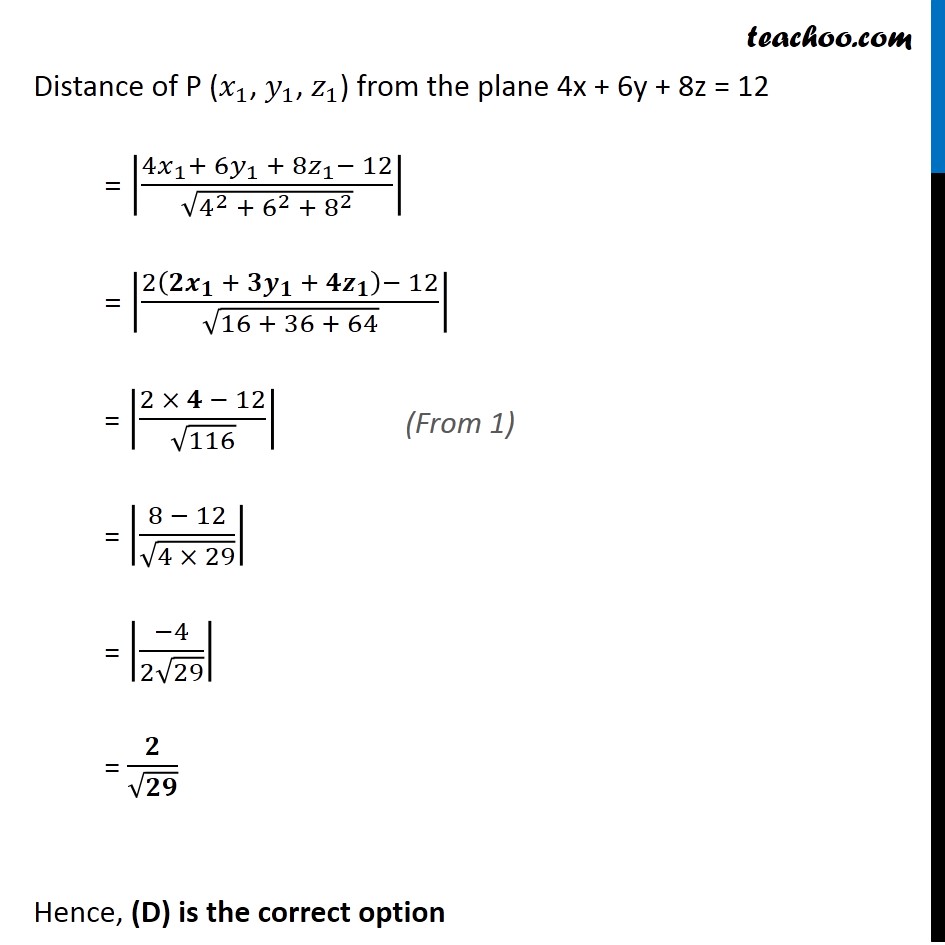
Misc 22 (Method 1) Distance between two planes: 2x + 3y + 4z = 4 and 4x + 6y + 8z = 12 is (A) 2 units (B) 4 units (C) 8 units (D) 2/√29 units Distance between two parallel planes Ax + By + Cz = 𝑑_1 and Ax + By + Cz = 𝑑_2 is |(𝒅_𝟏 − 𝒅_𝟐)/(√(𝑨^𝟐 + 𝑩^𝟐 + 𝑪^𝟐 ) )| 2x + 3y + 4z = 4 Comparing with Ax + By + Cz = d1 A = 2, B = 3, C = 4, d1 = 4 4x + 6y + 8z = 12 2 (2x + 3y + 4z) = 12 Dividing by 2 2x + 3y + 4z = 6 Comparing with Ax + By + Cz = d2 A = 2, B = 3, C = 4 , d2 = 6 So, Distance between the two planes = |(4 − 6)/√(2^2 + 3^2 + 4^2 )| = |(−2)/√(4 + 9 + 16)| = 𝟐/√𝟐𝟗 Hence, (D) is the correct option Misc 22 (Method 2) Distance between the two planes : 2x + 3y + 4z = 4 and 4x + 6y + 8z = 12 is (A) 2 units (B) 4 units (C) 8 units (D)2/√29 units Distance of a point (𝑥_1, 𝑦_1, 𝑧_1) from the plane Ax + By + Cz = D is |(𝑨𝒙_𝟏 + 𝑩𝒚_𝟏 + 𝑪𝒛_𝟏− 𝑫)/√(𝑨^𝟐 + 𝑩^𝟐 + 𝑪^𝟐 )| Let us take a point P (𝑥_1, 𝑦_1, 𝑧_1) on the plane 2x + 3y + 4z = 4 2𝑥_1 + 3𝑦_1 + 4𝑧_1 = 4 Now, to find the distance of point P form plane 4x + 6y + 8z = 12, Comparing with Ax + By + Cz = D, A = 4, B = 6, C = 8, D = 12 Distance of P (𝑥_1, 𝑦_1, 𝑧_1) from the plane 4x + 6y + 8z = 12 = |(4𝑥_1+ 〖6𝑦〗_1 + 8𝑧_1− 12)/√(4^2 + 6^2 + 8^2 )| = |(2(𝟐𝒙_𝟏 + 〖𝟑𝒚〗_𝟏 + 𝟒𝒛_𝟏 )− 12)/√(16 + 36 + 64)| = |(2 × 𝟒 − 12)/√116| = |(8 − 12)/√(4 × 29)| = |(−4)/(2√29)| = 𝟐/√𝟐𝟗 Hence, (D) is the correct option