Question 7 - Miscellaneous - Chapter 11 Class 12 Three Dimensional Geometry

Transcript

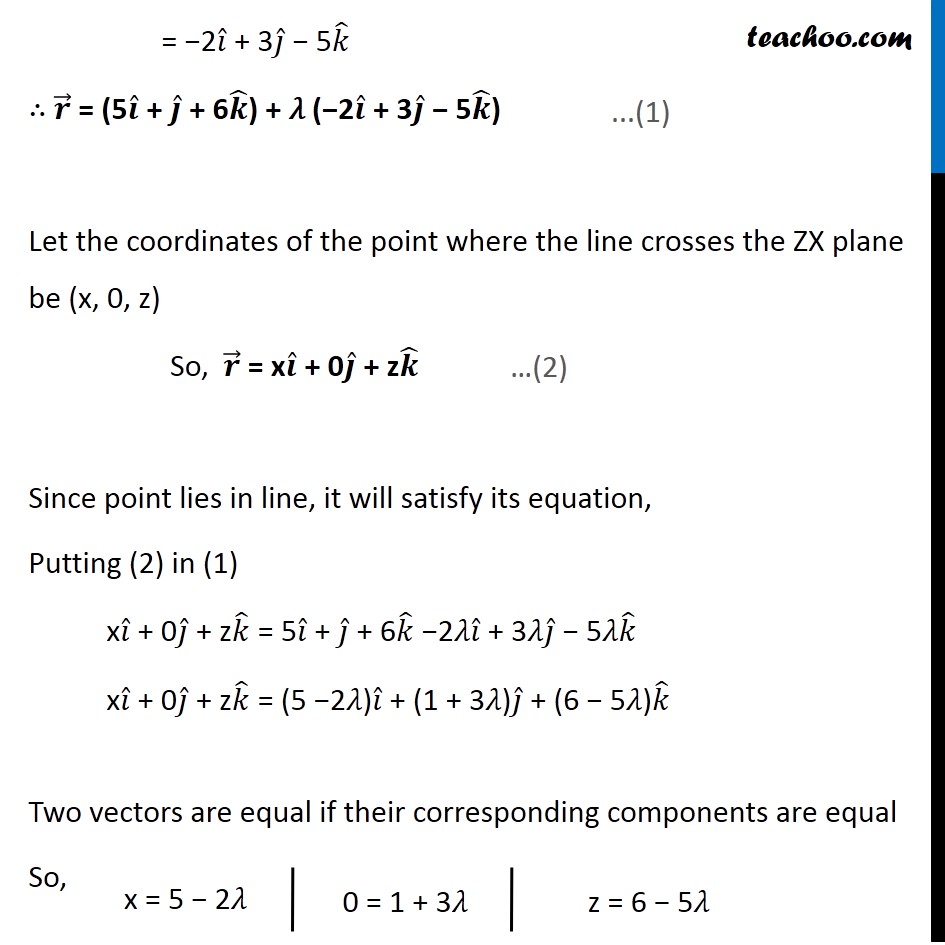
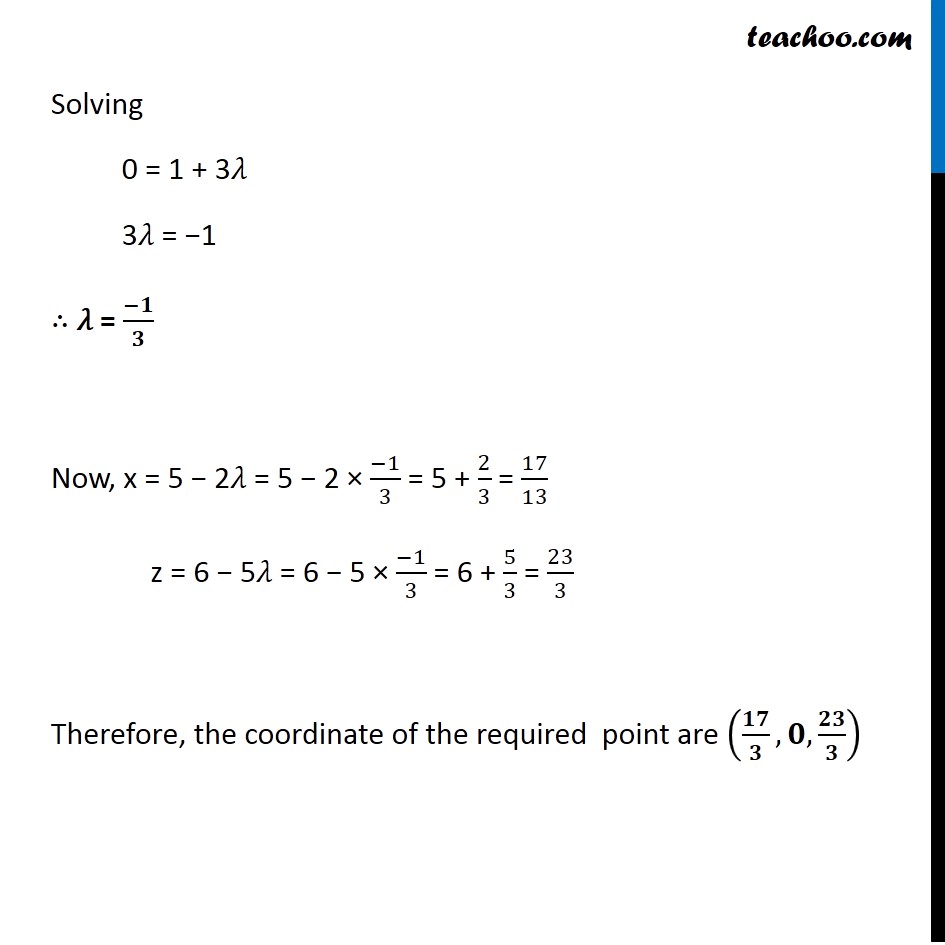
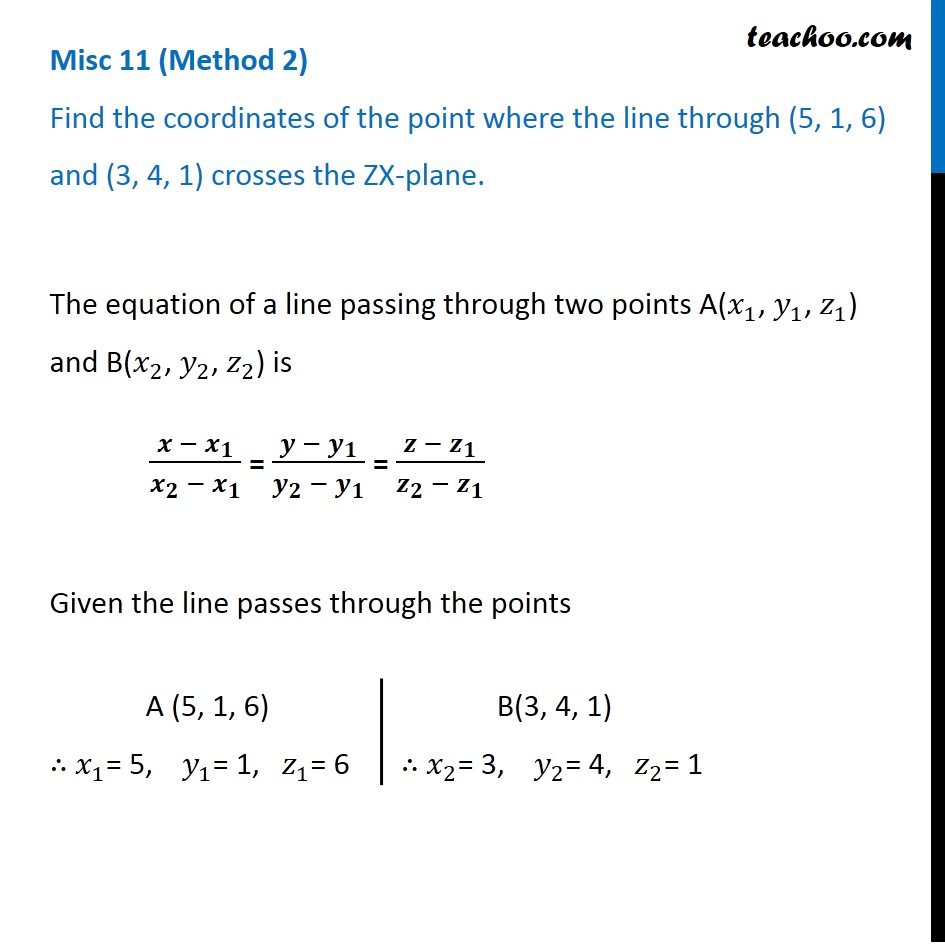
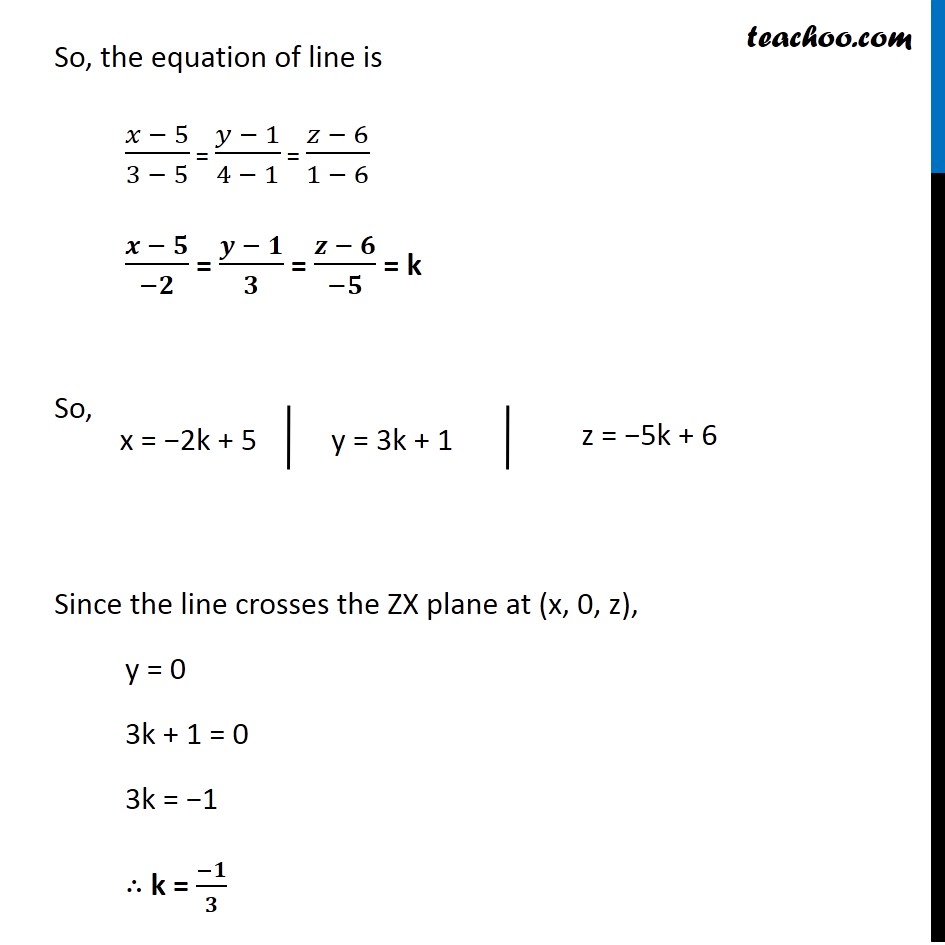
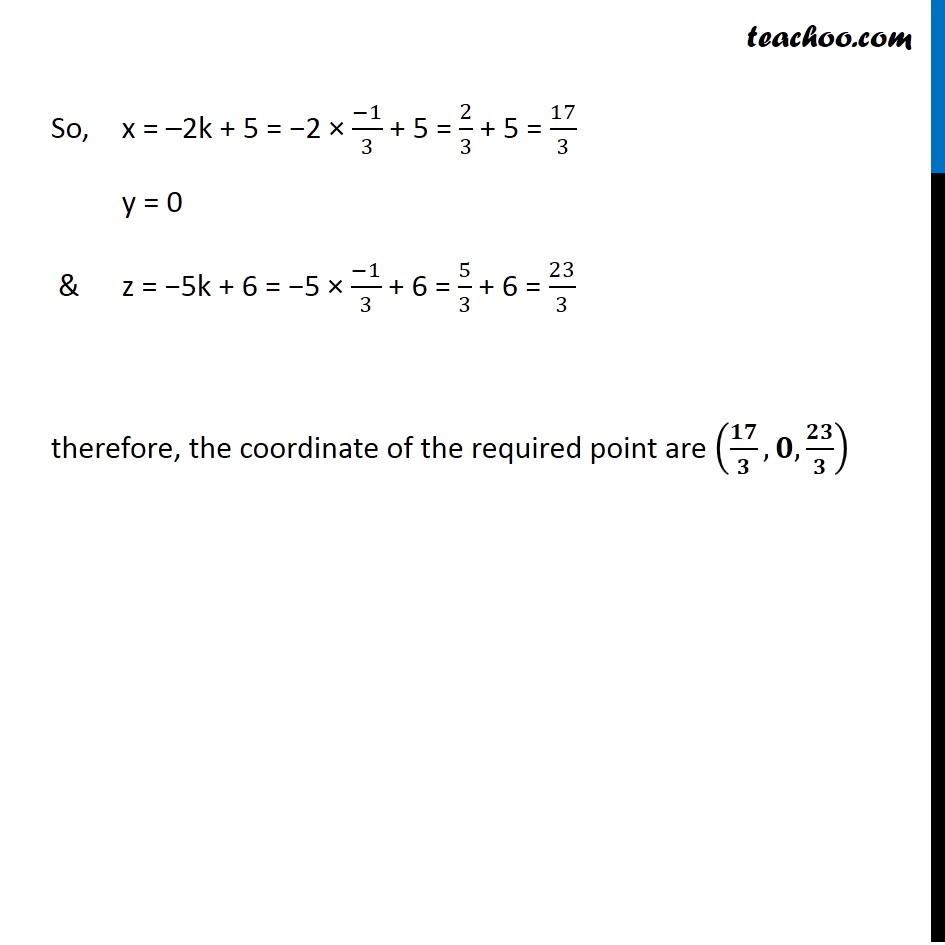
Question 7 (Method 1) Find the coordinates of the point where the line through (5, 1, 6) and (3, 4, 1) crosses the ZX-plane.The equation of a line passing through two points with position vectors 𝑎 ⃗ & 𝑏 ⃗ is 𝒓 ⃗ = 𝒂 ⃗ + 𝜆(𝒃 ⃗ − 𝒂 ⃗) Given, the line passes through (𝑏 ⃗ − 𝑎 ⃗) = (3𝑖 ̂ + 4𝑗 ̂ + 1𝑘 ̂) − (5𝑖 ̂ + 1𝑗 ̂ + 6𝑘 ̂) = (3 −5)𝑖 ̂ + (4 − 1)𝑗 ̂ + (1 − 6)𝑘 ̂ A (5, 1, 6) 𝑎 ⃗ = 5𝑖 ̂ + 1𝑗 ̂ + 6𝑘 ̂ B(3, 4, 1) 𝑏 ⃗ = 3𝑖 ̂ + 4𝑗 ̂ + 1𝑘 ̂ = −2𝑖 ̂ + 3𝑗 ̂ − 5𝑘 ̂ ∴ 𝒓 ⃗ = (5𝒊 ̂ + 𝒋 ̂ + 6𝒌 ̂) + 𝜆 (−2𝒊 ̂ + 3𝒋 ̂ − 5𝒌 ̂) Let the coordinates of the point where the line crosses the ZX plane be (x, 0, z) So, 𝒓 ⃗ = x𝒊 ̂ + 0𝒋 ̂ + z𝒌 ̂ Since point lies in line, it will satisfy its equation, Putting (2) in (1) x𝑖 ̂ + 0𝑗 ̂ + z𝑘 ̂ = 5𝑖 ̂ + 𝑗 ̂ + 6𝑘 ̂ −2𝜆𝑖 ̂ + 3𝜆𝑗 ̂ − 5𝜆𝑘 ̂ x𝑖 ̂ + 0𝑗 ̂ + z𝑘 ̂ = (5 −2𝜆)𝑖 ̂ + (1 + 3𝜆)𝑗 ̂ + (6 − 5𝜆)𝑘 ̂ Two vectors are equal if their corresponding components are equal So, Solving 0 = 1 + 3𝜆 3𝜆 = −1 ∴ 𝜆 = (−𝟏)/𝟑 Now, x = 5 − 2𝜆 = 5 − 2 × (−1)/3 = 5 + 2/3 = 17/13 z = 6 − 5𝜆 = 6 − 5 × (−1)/3 = 6 + 5/3 = 23/3 Therefore, the coordinate of the required point are (𝟏𝟕/𝟑,𝟎,𝟐𝟑/𝟑) Question 7 (Method 2) Find the coordinates of the point where the line through (5, 1, 6) and (3, 4, 1) crosses the ZX-plane.The equation of a line passing through two points A(𝑥_1, 𝑦_1, 𝑧_1) and B(𝑥_2, 𝑦_2, 𝑧_2) is (𝒙 − 𝒙_𝟏)/(𝒙_𝟐 − 𝒙_𝟏 ) = (𝒚 − 𝒚_𝟏)/(𝒚_𝟐 − 𝒚_𝟏 ) = (𝒛 − 𝒛_𝟏)/(𝒛_𝟐 − 𝒛_𝟏 ) Given the line passes through the points A (5, 1, 6) ∴ 𝑥_1= 5, 𝑦_1= 1, 𝑧_1= 6 B(3, 4, 1) ∴ 𝑥_2= 3, 𝑦_2= 4, 𝑧_2= 1 So, the equation of line is (𝑥 − 5)/(3 − 5) = (𝑦 − 1)/(4 − 1) = (𝑧 − 6)/(1 − 6) (𝒙 − 𝟓)/(−𝟐) = (𝒚 − 𝟏)/𝟑 = (𝒛 − 𝟔)/(−𝟓) = k So, Since the line crosses the ZX plane at (x, 0, z), y = 0 3k + 1 = 0 3k = −1 ∴ k = (−𝟏)/𝟑 So, x = –2k + 5 = −2 × (−1)/3 + 5 = 2/3 + 5 = 17/3 y = 0 & z = −5k + 6 = −5 × (−1)/3 + 6 = 5/3 + 6 = 23/3 therefore, the coordinate of the required point are (𝟏𝟕/𝟑,𝟎,𝟐𝟑/𝟑)