Question 5 - Examples - Chapter 4 Class 12 Determinants

Last updated at Dec. 16, 2024 by

Example 10 - Show |a b c a+2x b+2y c+2z x y z| = 0 - Examples

Example 10 - Chapter 4 Class 12 Determinants - Part 2
Example 10 - Chapter 4 Class 12 Determinants - Part 3

Go Ad-free

Transcript

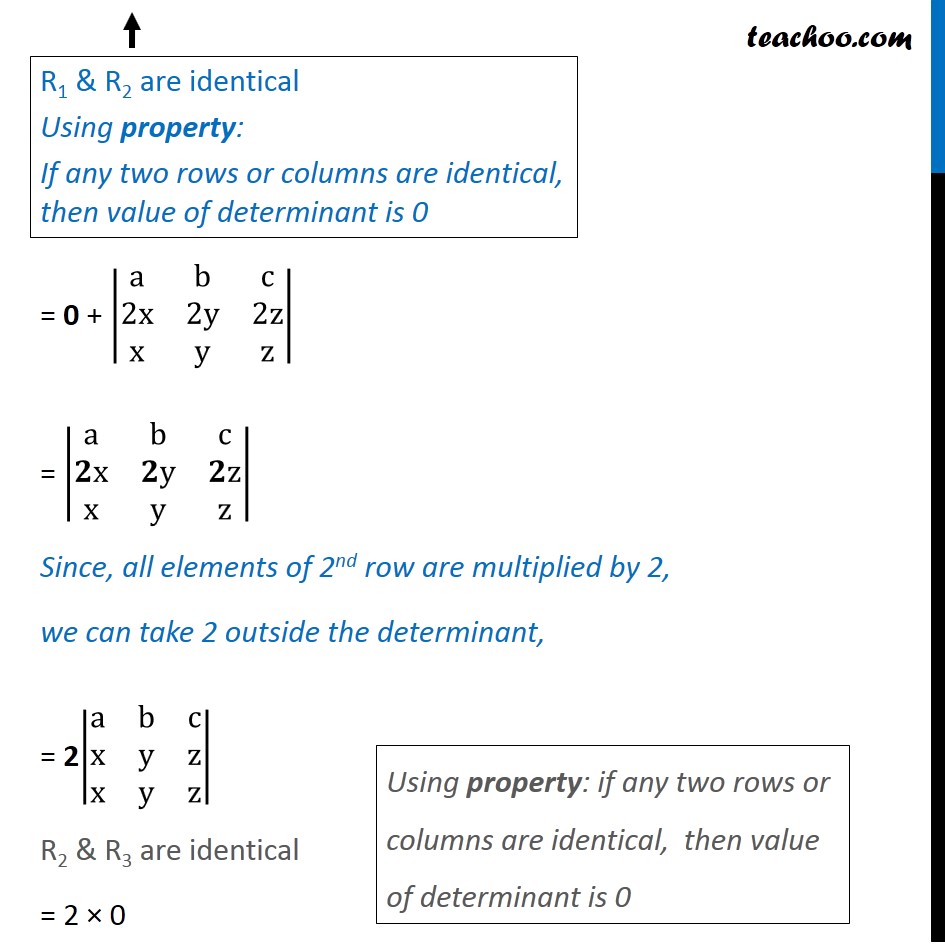
Question 5 Show that |■8(a&b&c@a+2x&b+2y&c+2z@x&y&z)| = 0 Solving L.H.S |■8(a&b&c@a+2x&b+2y&c+2z@x&y&z)| Expressing elements of 2nd row as sum of two elements = |■8(𝐚&𝐛&𝐜@𝐚&𝐛&𝐜@x&y&z)| + |■8(a&b&c@2x&2y&2z@x&y&z)| By Property 5: If some or all elements of a row or column of a determinant are expressed as sum of two (or more) terms ,then the determinant is expressed as a sum of two (or more) determinants. R1 & R2 are identical Using property: If any two rows or columns are identical, then value of determinant is 0 = 0 + |■8(a&b&c@2x&2y&2z@x&y&z)| = |■8(a&b&c@𝟐x&𝟐y&𝟐z@x&y&z)| Since, all elements of 2nd row are multiplied by 2, we can take 2 outside the determinant, = 2|■8(a&b&c@x&y&z@x&y&z)| R2 & R3 are identical = 2 × 0 Using property: if any two rows or columns are identical, then value of determinant is 0 = 0 = R.H.S Thus L.H.S = R.H.S Hence proved

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo