Get live Maths 1-on-1 Classs - Class 6 to 12
Example 14 - Chapter 6 Class 10 Triangles (Term 1)
Last updated at March 28, 2023 by Teachoo
Examples
Example 1
Example 2 Important
Example 3
Example 4
Example 5 Important
Example 6
Example 7 Important
Example 8 Important
Example 9 Important Deleted for CBSE Board 2023 Exams
Example 10 Important Deleted for CBSE Board 2023 Exams
Example 11 Deleted for CBSE Board 2023 Exams
Example 12 Deleted for CBSE Board 2023 Exams
Example 13 Important Deleted for CBSE Board 2023 Exams
Example 14 Important Deleted for CBSE Board 2023 Exams You are here
Chapter 6 Class 10 Triangles
Serial order wise
Transcript
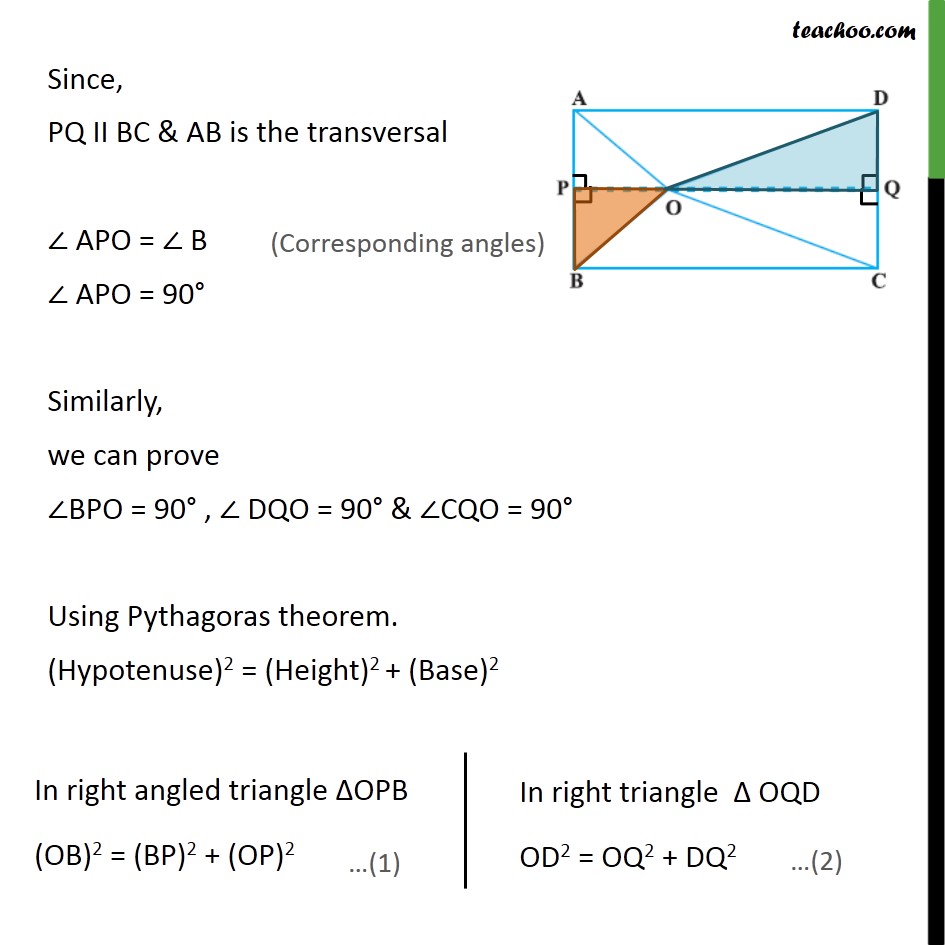
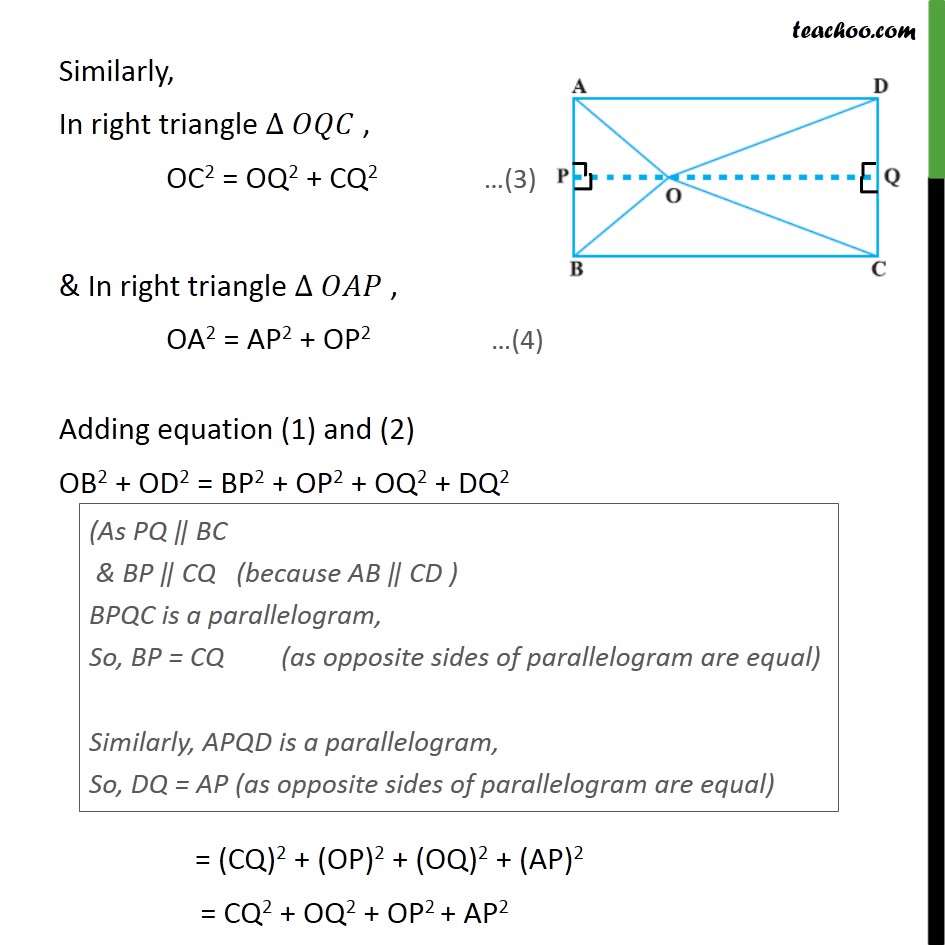
Example 14 O is any point inside a rectangle ABCD (see Fig. 6.52). Prove that OB2 + OD2 = OA2 + OC2. Given : Rectangle ABCD , and a point O inside rectangle . To prove :- OB2 + OD2 = OA2 + OC2 Proof :- Let us draw a line PQ, through O which is parallel to BC. Hence, PQ II BC ⇒ PQ II AD All angles of a rectangle are 90° , So, ∠ A = ∠ B = ∠ C = ∠ D = 90° Since, PQ II BC & AB is the transversal ∠ APO = ∠ B ∠ APO = 90° Similarly, we can prove ∠BPO = 90° , ∠ DQO = 90° & ∠CQO = 90° Using Pythagoras theorem. (Hypotenuse)2 = (Height)2 + (Base)2 Similarly, In right triangle ∆ 𝑂𝑄𝐶 , OC2 = OQ2 + CQ2 …(3) & In right triangle ∆ 𝑂𝐴𝑃 , OA2 = AP2 + OP2 …(4) Adding equation (1) and (2) OB2 + OD2 = BP2 + OP2 + OQ2 + DQ2 = (CQ)2 + (OP)2 + (OQ)2 + (AP)2 = CQ2 + OQ2 + OP2 + AP2 = OC2 + OA2 Thus, OB2 + OD2 = OC2 + OA2 Hence proved
