Question 1 - Examples - Chapter 6 Class 10 Triangles

Last updated at May 29, 2023 by

Example 9 - Line segment XY is parallel to side AC of ABC - Area of similar triangles

Example 9 - Chapter 6 Class 10 Triangles - Part 2
Example 9 - Chapter 6 Class 10 Triangles - Part 3

Learn in your speed, with individual attention - Teachoo Maths 1-on-1 Class

Book a free demo

Transcript

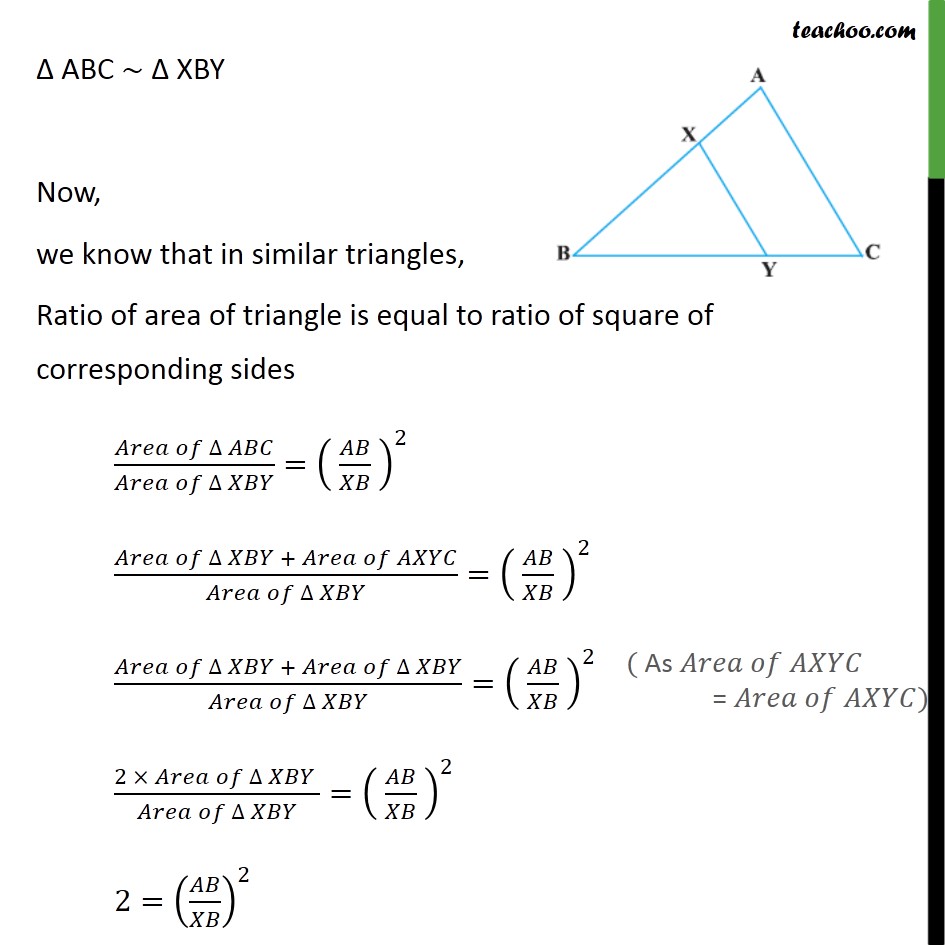
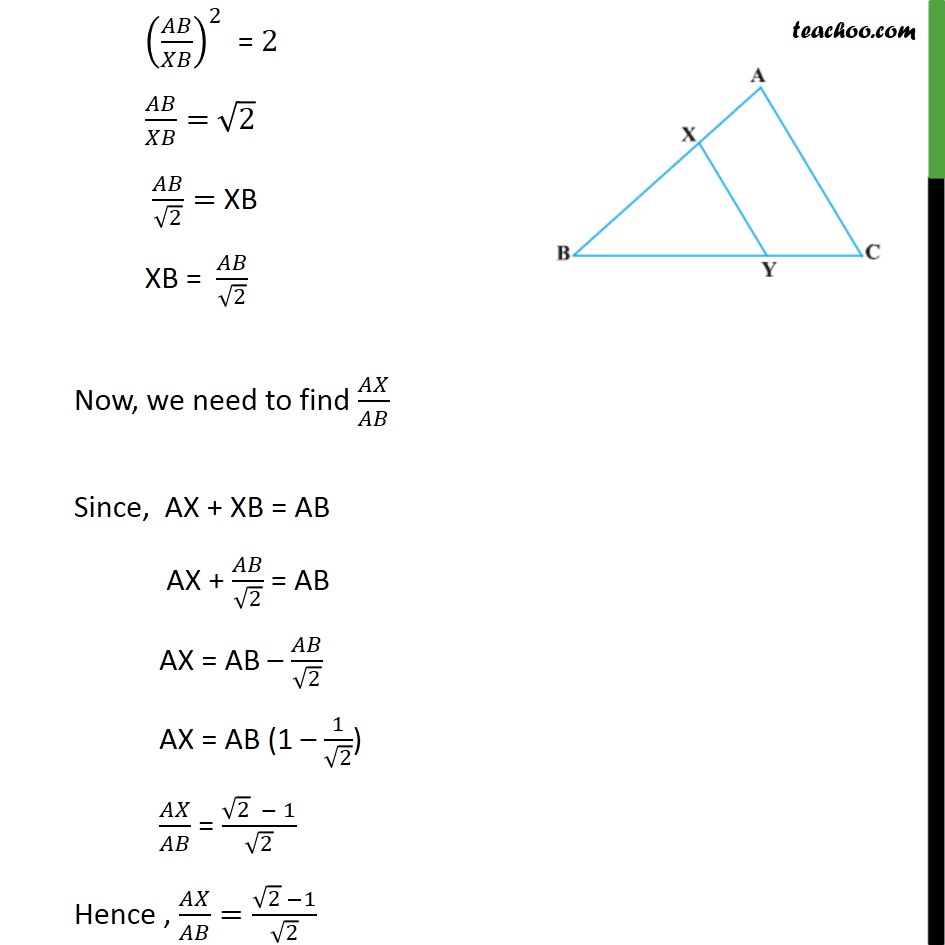
Question 1 In figure, the line segment XY is parallel to side AC of ABC and it divides the triangle into two parts of equal areas. Find the ratio / Given: ABC XY is parallel to AC i.e. XY II AC ar( BXY ) = ar(AXYC) To find : / Proof: In ABC & XBY, ABC = XBY ACB = XYB ABC ~ XBY ABC ~ XBY Now, we know that in similar triangles, Ratio of area of triangle is equal to ratio of square of corresponding sides ( )/( )=( / )^2 ( + )/( )=( / )^2 ( + )/( )=( / )^2 (2 )/( )=( / )^2 2=( / )^2 ( / )^2 = 2 / = 2 / 2= XB XB = / 2 Now, we need to find / Since, AX + XB = AB AX + / 2 = AB AX = AB / 2 AX = AB (1 1/ 2) / = ( 2 1)/ 2 Hence , / =( 2 1)/ 2

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 13 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.