Question 27 - CBSE Class 12 Sample Paper for 2021 Boards - Solutions of Sample Papers and Past Year Papers - for Class 12 Boards

Last updated at April 16, 2024 by Teachoo

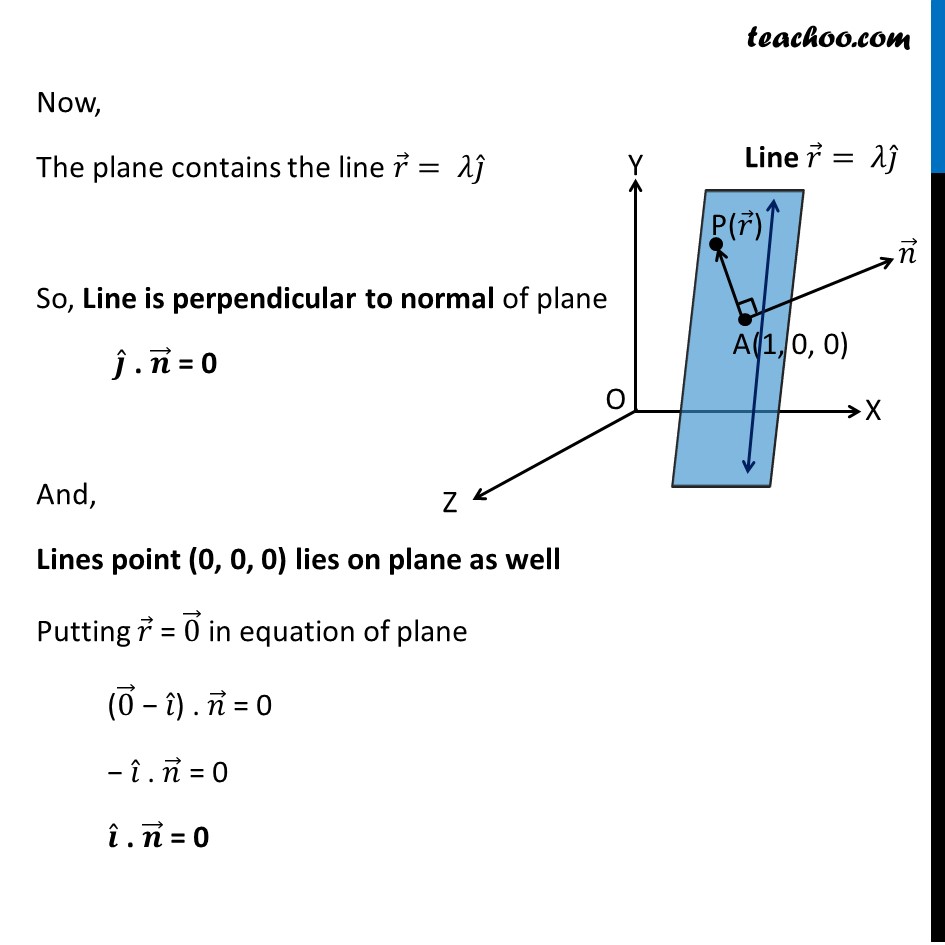
Find the vector equation of the plane that passes through the point (1, 0, 0) and contains the line r ^→ = ⋏i ̂ j ̂

Find vector equation of plane that passes through the point (1,0,0)

Question 27 - CBSE Class 12 Sample Paper for 2021 Boards - Part 2

Question 27 - CBSE Class 12 Sample Paper for 2021 Boards - Part 3

Transcript

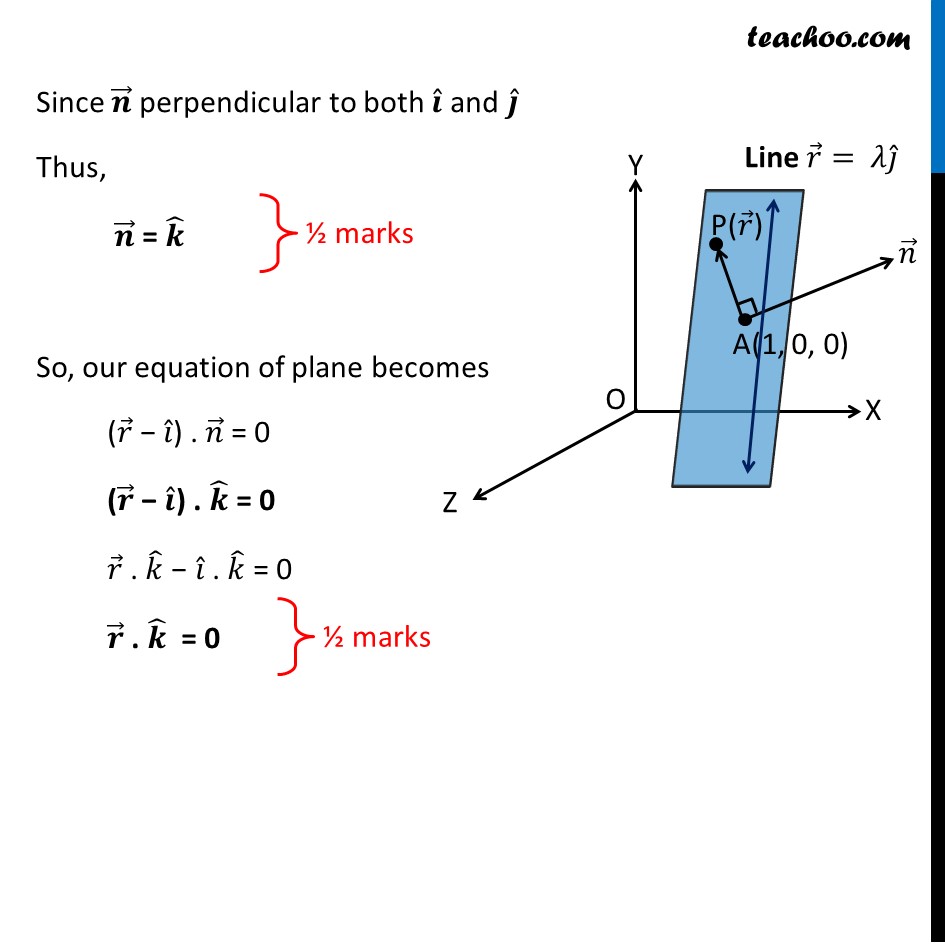
Equation of plane passing through point A whose position vector is 𝒂 ⃗ & perpendicular to 𝒏 ⃗ is (𝒓 ⃗ − 𝒂 ⃗) . 𝒏 ⃗ = 0 Given Plane passes through (1, 0, 0) So 𝒂 ⃗ = 1𝑖 ̂ + 0𝑗 ̂ − 0𝑘 ̂ 𝒂 ⃗ = 𝑖 ̂ Thus, equation of plane is (𝑟 ⃗ − 𝑎 ⃗) . 𝑛 ⃗ = 0 (𝒓 ⃗ − 𝒊 ̂) . 𝒏 ⃗ = 0 Now, The plane contains the line 𝑟 ⃗= 𝜆𝑗 ̂ So, Line is perpendicular to normal of plane 𝒋 ̂ . 𝒏 ⃗ = 0 And, Lines point (0, 0, 0) lies on plane as well Putting 𝑟 ⃗ = 0 ⃗ in equation of plane (0 ⃗ − 𝑖 ̂) . 𝑛 ⃗ = 0 − 𝑖 ̂ . 𝑛 ⃗ = 0 𝒊 ̂ . 𝒏 ⃗ = 0 Since 𝒏 ⃗ perpendicular to both 𝒊 ̂ and 𝒋 ̂ Thus, 𝒏 ⃗ = 𝒌 ̂ So, our equation of plane becomes (𝑟 ⃗ − 𝑖 ̂) . 𝑛 ⃗ = 0 (𝒓 ⃗ − 𝒊 ̂) . 𝒌 ̂ = 0 𝑟 ⃗ . 𝑘 ̂ − 𝑖 ̂ . 𝑘 ̂ = 0 𝒓 ⃗ . 𝒌 ̂ = 0

Next: Question 28 (Choice 1) → Go Ad-free

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular