641 students have Teachoo Black. What are you waiting for?
Ex 11.3, 16 - Chapter 11 Class 11 Conic Sections (Term 2)
Last updated at Feb. 6, 2020 by Teachoo
Ex 11.3
Ex 11.3, 1
Ex 11.3, 2 Important
Ex 11.3, 3
Ex 11.3, 4
Ex 11.3, 5 Important
Ex 11.3, 6
Ex 11.3, 7 Important
Ex 11.3, 8
Ex 11.3, 9
Ex 11.3, 10
Ex 11.3, 11 Important
Ex 11.3, 12 Important
Ex 11.3, 13
Ex 11.3, 14 Important
Ex 11.3, 15
Ex 11.3, 16 Important You are here
Ex 11.3, 17
Ex 11.3, 18 Important
Ex 11.3, 19 Important
Ex 11.3, 20
Chapter 11 Class 11 Conic Sections (Term 2)
Serial order wise
- Ex 11.1
- Ex 11.2
-
Ex 11.3
- Ex 11.4
- Examples
- Miscellaneous
Transcript
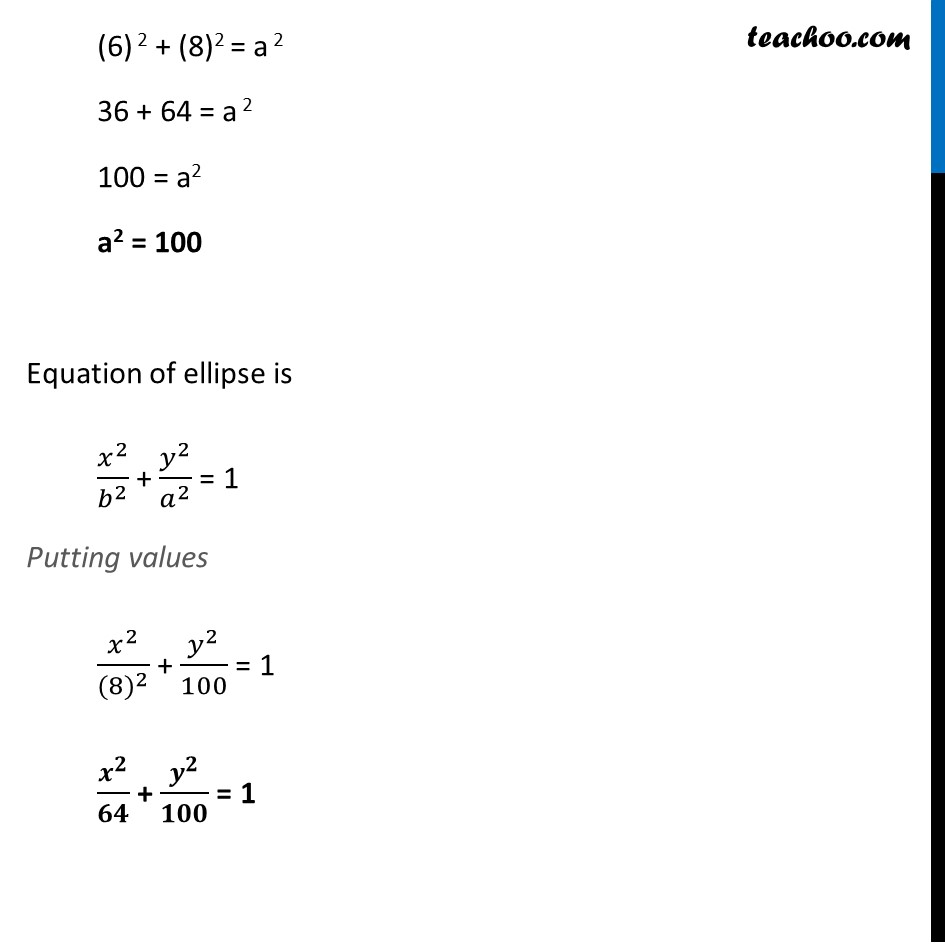
Ex 11.3, 16 Find the equation for the ellipse that satisfies the given conditions: Length of minor axis 16, foci (0, ±6) We need to find equation of ellipse whose length of minor axis = 16 & Foci = (0, ±6) Since foci is of the type (0, ±c) The major axis is along the y-axis. & required Equation of Ellipse is 𝒙^𝟐/𝒃^𝟐 + 𝒚^𝟐/𝒂^𝟐 = 1 From (1) & (2) c = 6 Given length of major axis = 16 & we know that Length of manor axis = 2b 16 = 2b 2b = 16 b = 16/2 b = 8 Also, We know that c2 = a2 − b2 (6) 2 = a 2 − (8)2 (6) 2 + (8)2 = a 2 36 + 64 = a 2 100 = a2 a2 = 100 Equation of ellipse is 𝑥^2/𝑏^2 + 𝑦^2/𝑎^2 = 1 Putting values 𝑥^2/〖(8)〗^2 + 𝑦^2/100 = 1 𝒙^𝟐/𝟔𝟒 + 𝒚^𝟐/𝟏𝟎𝟎 = 1
