Ex 10.3, 14 - Chapter 10 Class 11 Conic Sections

Last updated at May 29, 2023 by

Ex 11.3, 14 - Find ellipse: ends of major axis (0, 5), minor

Ex 11.3, 14 - Chapter 11 Class 11 Conic Sections - Part 2
Ex 11.3, 14 - Chapter 11 Class 11 Conic Sections - Part 3

Learn in your speed, with individual attention - Teachoo Maths 1-on-1 Class

Book a free demo

Transcript

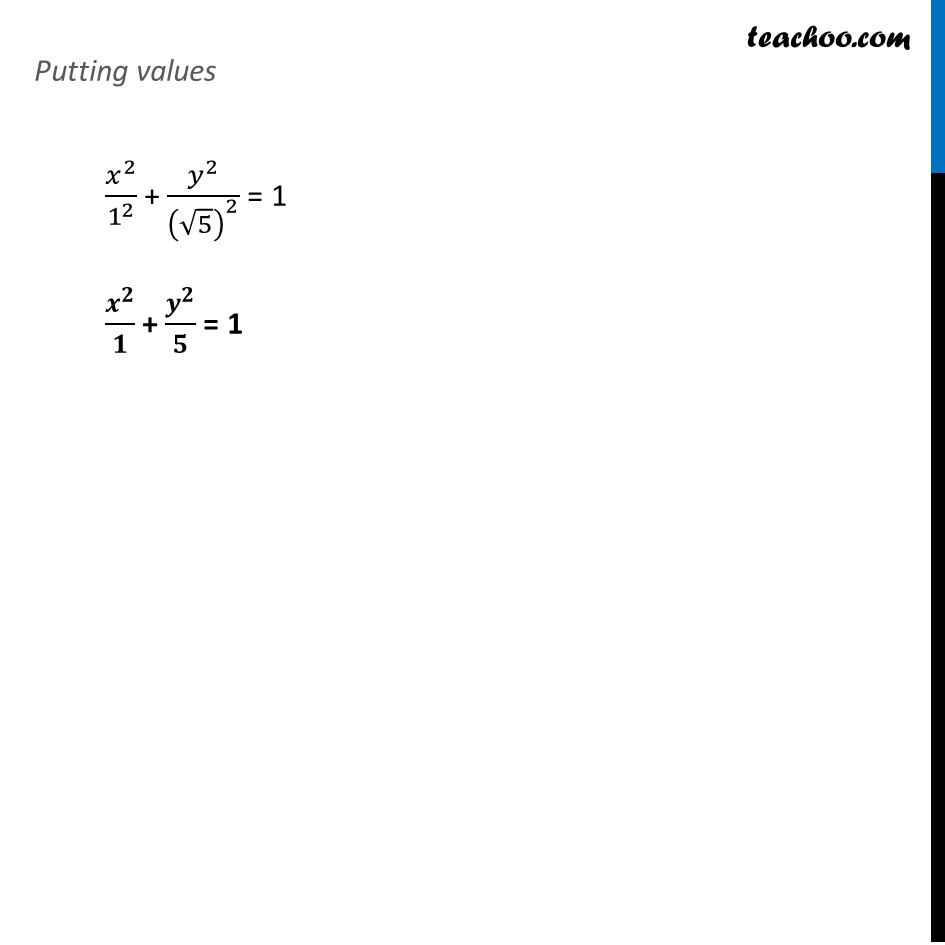
Ex 10.3, 14 Find the equation for the ellipse that satisfies the given conditions: Ends of major axis (0, ± √5) , ends of minor axis (±1, 0) Given ends of Major Axis (0, ± √5), & ends of Minor Axis (±1, 0) Major axis is along the y-axis So, our required equation of ellipse is 𝒙^𝟐/𝒃^𝟐 + 𝒚^𝟐/𝒂^𝟐 = 1 We know that End of major axis is the vertices of the ellipse So vertices of ellipse = (0, ± √5) Also, Vertices of the ellipse is (0, ± a) Comparing (0, ± a) = (0, ± √5) a = √𝟓 We know that End of minor axis = (± b, 0) So, (±1, 0) = (± b, 0) So, b = 1 Required equation of ellipse is 𝑥^2/𝑏^2 + 𝑦^2/𝑎^2 = 1 Putting values 𝑥^2/1^2 + 𝑦^2/(√5)^2 = 1 𝒙^𝟐/𝟏 + 𝒚^𝟐/𝟓 = 1

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 13 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.