











Examples
Examples
Last updated at Dec. 16, 2024 by Teachoo














Transcript
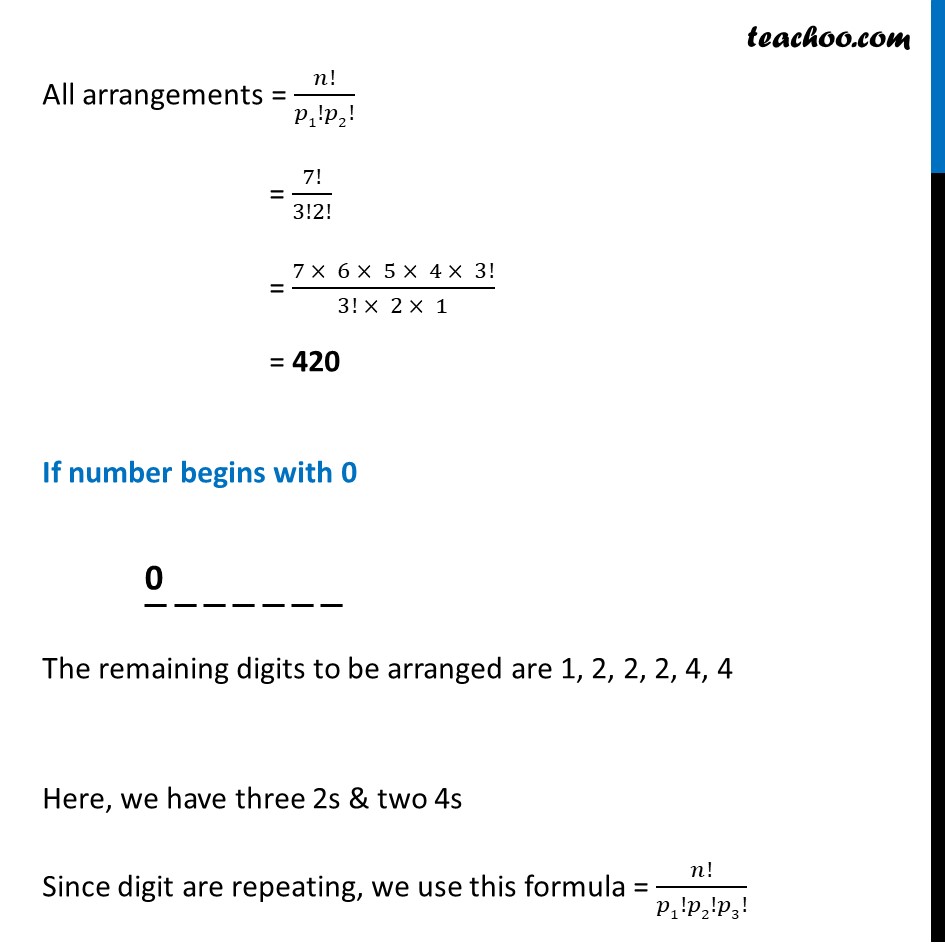
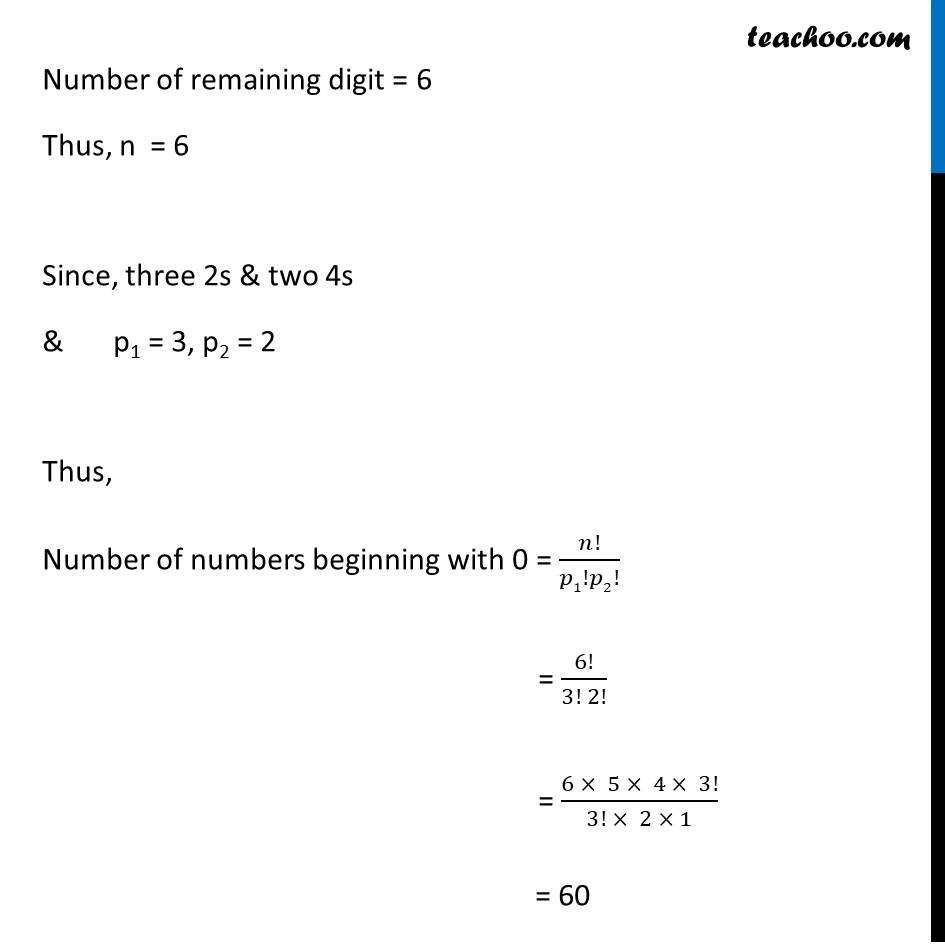
Example 23 (Method 1) How many numbers greater than 1000000 can be formed by using the digits 1, 2, 0, 2, 4, 2, 4? There are total 7 digits in 1000000 We need to form a 7 digit number using the digits 1, 2, 0, 2, 4, 2, 4 But, these include numbers starting with ‘0’ like 0412224, …etc which are actually 6 digit numbers Hence, we can’t have number beginning with 0 Thus, Required numbers = All arrangements – Numbers starting with 0 All arrangements The digits to be arranged are 1, 0, 2, 2, 2, 4, 4 Here, three 2s & two 4s Since digit are repeating, we use this formula = 𝑛!/(𝑝1! 𝑝2! 𝑝3!) Number of digits = 7 Thus, n = 7 Since, three 2s & two 4s & p1 = 3, p2 = 2 All arrangements = 𝑛!/𝑝1!𝑝2! = 7!/3!2! = (7 × 6 × 5 × 4 × 3!)/(3! × 2 × 1) = 420 If number begins with 0 The remaining digits to be arranged are 1, 2, 2, 2, 4, 4 Here, we have three 2s & two 4s Since digit are repeating, we use this formula = 𝑛!/𝑝1!𝑝2!𝑝3! Number of remaining digit = 6 Thus, n = 6 Since, three 2s & two 4s & p1 = 3, p2 = 2 Thus, Number of numbers beginning with 0 = 𝑛!/𝑝1!𝑝2! = 6!/(3! 2!) = (6 × 5 × 4 × 3!)/(3! × 2 × 1) = 60 Now, Required numbers = All arrangements – Numbers starting with 0 = 420 – 60 = 360 Example 23 (Method 2) How many numbers greater than 1000000 can be formed by using the digits 1, 2, 0, 2, 4, 2, 4? There are total 7 digits in 1000000 We need to form a 7 digit number using the digits 1, 2, 0, 2, 4,2, 4 But, these include numbers starting with ‘0’ like 0412224, …etc. which are actually 6 digit numbers Hence, we cant have number beginning with 0 So the number can begin either with 1, 2 or 4 Thus, Required numbers = Numbers starting with 1 + Numbers starting with 2 + Numbers starting with 4 Thus, Required numbers = Numbers starting with 1 + Numbers starting with 2 + Numbers starting with 4 If number begins with 1 The remaining digits to be arranged are 0, 2, 2, 2, 4, 4 Here, we have three 2s & two 4s Since digit are repeating, we use this formula = 𝑛!/𝑝1!𝑝2!𝑝3! Number of remaining digit = 6 Thus, n = 6 Since, three 2s & two 4s & p1 = 3, p2 = 2 Thus, Number of numbers beginning with 0 = 𝑛!/𝑝1!𝑝2! = 6!/(3! 2!) = (6 × 5 × 4 × 3!)/(3! × 2 × 1) = 60 If number begins with 2 The remaining digits to be arranged are 0, 1, 2, 2, 4, 4 Here, we have two 2s & two 4s Since digit are repeating, we use this formula = 𝑛!/𝑝1!𝑝2!𝑝3! Number of remaining digit = 6 Thus, n = 6 Since, two 2s & two 4s & p1 = 2, p2 = 2 Thus, Number of numbers beginning with 0 = 𝑛!/𝑝1!𝑝2! = 6!/(2! 2!) = (6 × 5 × 4 × 3 × 2!)/(2! × 2 × 1) = 180 If number begins with 4 The remaining digits to be arranged are 0, 1, 2, 2, 2, 4 Here, we have three 2s Since digit are repeating, we use this formula = 𝑛!/𝑝1!𝑝2!𝑝3! Number of remaining digit = 6 Thus, n = 6 Since, three 2s & p1 = 3, Thus, Number of numbers beginning with 0 = 𝑛!/𝑝1!𝑝2! = 6!/3! = (6 × 5 × 4 × 3!)/3! = 120 Thus, Required numbers = Numbers starting with 1 + Numbers starting with 2 + Numbers starting with 4 = 60 + 180 +120 = 360