Question 1 (iii) - Approximations (using Differentiation) - Chapter 6 Class 12 Application of Derivatives

Last updated at Dec. 16, 2024 by

Ex 6.4, 1 (iii) - Find approximate value upto 3 decimal places √0.6

Ex 6.4, 1 (iii) - Chapter 6 Class 12 Application of Derivatives - Part 2
Ex 6.4, 1 (iii) - Chapter 6 Class 12 Application of Derivatives - Part 3

Share on WhatsApp

Transcript

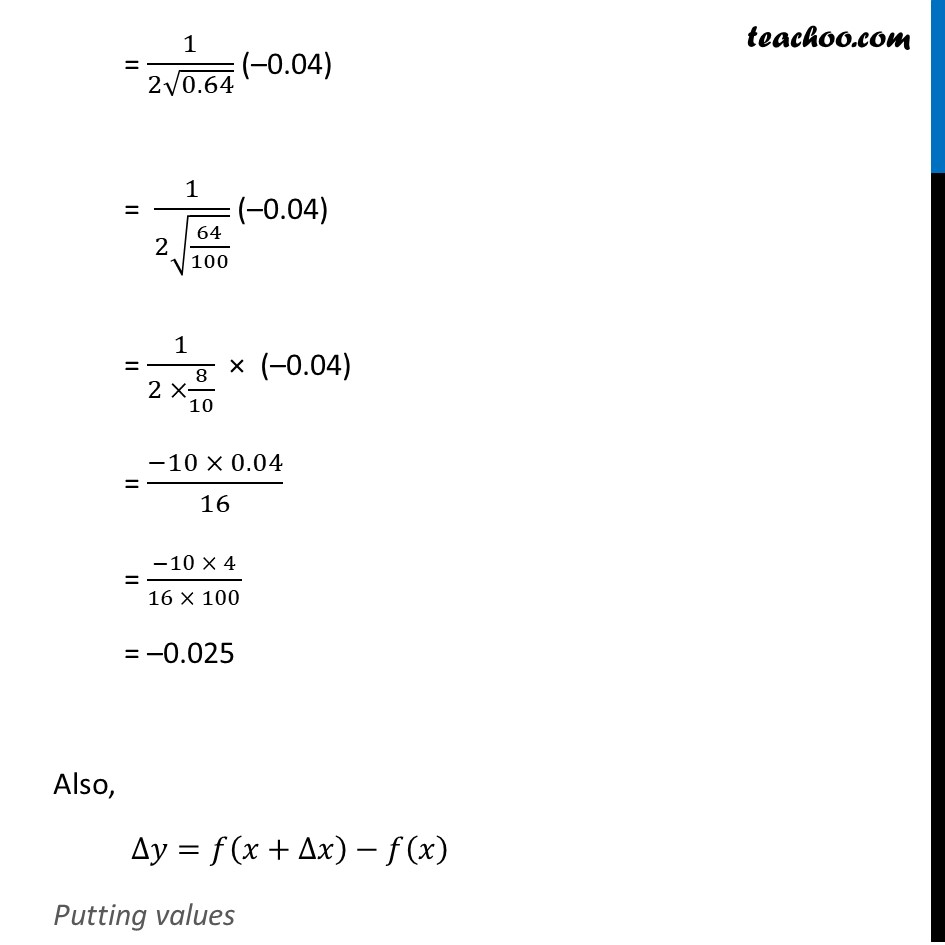
Question 1 Using differentials, find the approximate value of each of the following up to 3 places of decimal. (iii) √0.6 Let y = √𝑥 where x = 0.64 & △x = –0.04 Since y = √𝑥 𝑑𝑦/𝑑𝑥 = (𝑑(√𝑥))/𝑑𝑥 = 1/(2√𝑥) Now, ∆𝑦 = 𝑑𝑦/𝑑𝑥 △x = 1/(2√0.64) (–0.04) = 1/(2√(64/100)) (–0.04) = 1/(2 ×8/10) × (–0.04) = (−10 × 0.04)/16 = (−10 × 4)/(16 × 100) = –0.025 Also, ∆𝑦=𝑓(𝑥+∆𝑥)−𝑓(𝑥) Putting values ∆𝑦=√(𝑥+∆𝑥)−√𝑥 −0. 025=√(0.64−0.04)−√0.64 −0.025=√0.60−0.8 0.8 – 0. 025=√0.60 √0.60=0.775 Hence, approximate value of √0.60 is 0.775

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo