Learn in your speed, with individual attention - Teachoo Maths 1-on-1 Class
Ex 13.1, 12 - Chapter 13 Class 12 Probability
Last updated at Aug. 16, 2023 by Teachoo
Ex 13.1
Ex 13.1, 1
Ex 13.1, 2
Ex 13.1, 3 Important
Ex 13.1, 4
Ex 13.1, 5
Ex 13.1, 6 (i)
Ex 13.1, 6 (ii) Important
Ex 13.1, 6 (iii)
Ex 13.1, 7 (i)
Ex 13.1, 7 (ii)
Ex 13.1, 8
Ex 13.1, 9
Ex 13.1, 10 (a) Important
Ex 13.1, 10 (b) Important
Ex 13.1, 11
Ex 13.1, 12 Important You are here
Ex 13.1, 13 Important
Ex 13.1, 14
Ex 13.1, 15
Ex 13.1, 16 (MCQ) Important
Ex 13.1, 17 (MCQ) Important
Chapter 13 Class 12 Probability
Serial order wise
Transcript
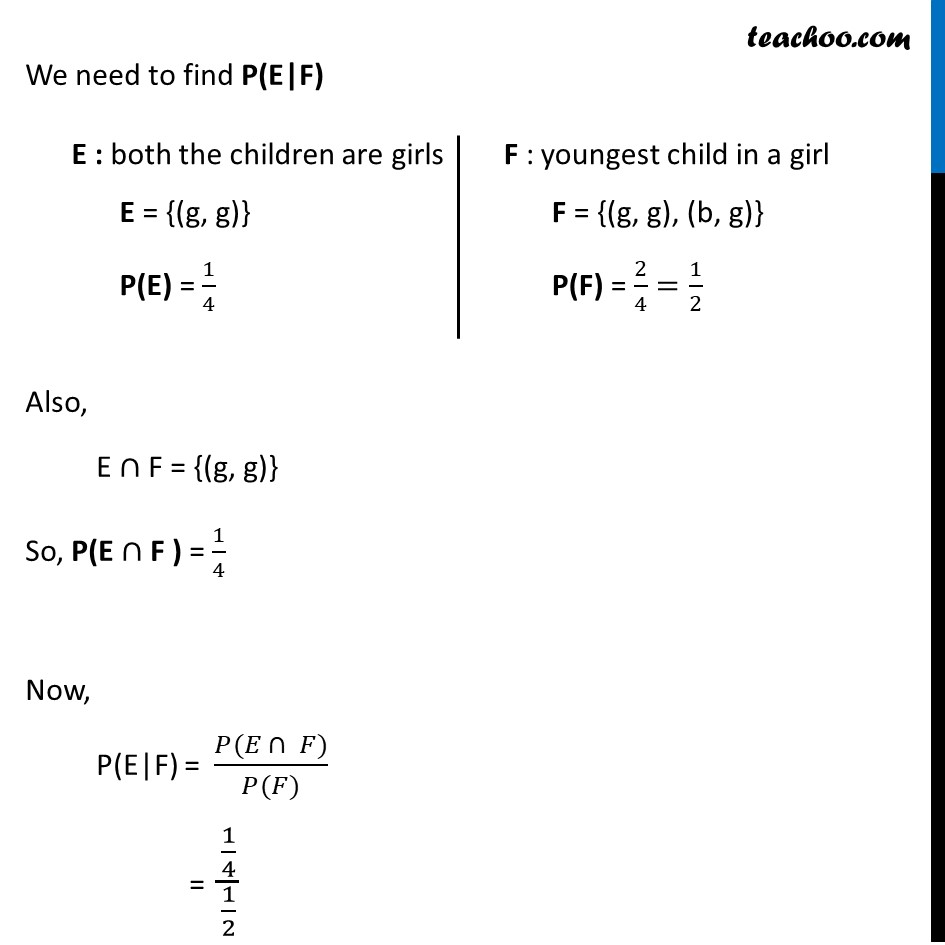
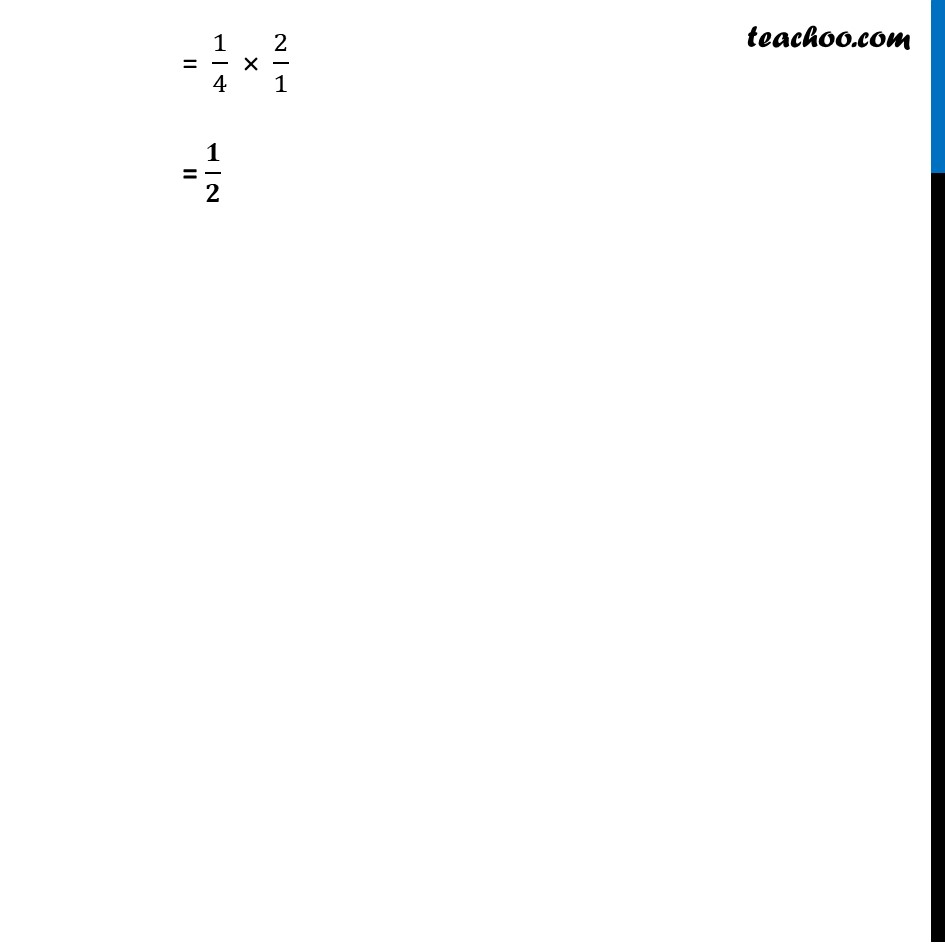
Ex 13.1, 12 Assume that each born child is equally likely to be a boy or a girl. If a family has two children, what is the conditional probability that both are girls given that (ii) at least one is a girl? S = {(g, g), (g, b), (b, g), (b, b)} We need to find the probability that the children are girls, given that at least one is a girl. E : both the children are girls F : at least one child is a girl E = {(g, g)} P(E) = 1/4 F = {(g, g), (g, b), (b, g)} P(F) = 3/4 Also, E ∩ F = {(g, g)} So, P(E ∩ F) = 1/4 Now, P(E|F) = (𝑃(𝐸 ∩ 𝐹))/(𝑃(𝐹)) = (1/4)/(3/4) = 1/4 × 4/3 = 𝟏/𝟑
