Example 7 - Chapter 2 Class 9 Polynomials (Important Question)

Last updated at December 16, 2024 by Teachoo

Example 7 - Find the value of k, if x – 1 is a factor - Examples - Examples

part 2 - Example 7 - Examples - Serial order wise - Chapter 2 Class 9 Polynomials

Next: Example 10 Important → Remove Ads Share on WhatsApp

Transcript

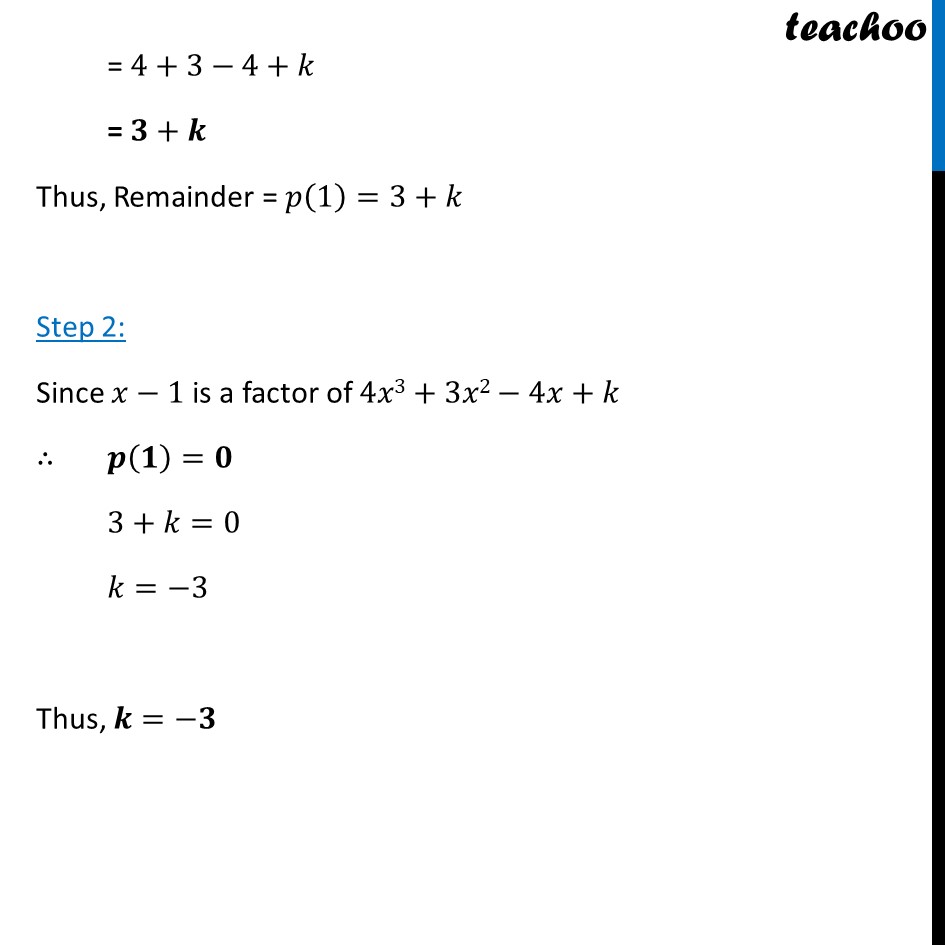
Example 7 Find the value of k, if 𝑥−1 is a factor of 4𝑥^3+3𝑥^2−4𝑥+𝑘 Finding remainder when 𝟒𝒙^𝟑+𝟑𝒙^𝟐−𝟒𝒙+𝒌 is divided by x – 1 Step 1: Putting Divisor = 0 x – 1 = 0 x = 1 Step 2: Let 𝑝(𝑥) = 4𝑥^3+3𝑥^2−4𝑥+𝑘 Putting x = 1 𝑝(𝟏) = 4〖(𝟏)〗^3+3〖(𝟏)〗^2− 4(𝟏)+𝑘 Dividend Divisor = 4+3− 4+𝑘 = 𝟑+𝒌 Thus, Remainder = 𝑝(1) = 3+𝑘 Step 3: Since 𝑥 – 1 is a factor of 4𝑥^3+3𝑥^2−4𝑥+𝑘 ∴ 𝒑(𝟏) = 0 3+𝑘 = 0 k = −3 Thus, k = −𝟑

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular