Example 4 - Chapter 8 Class 10 Introduction to Trignometry

Transcript

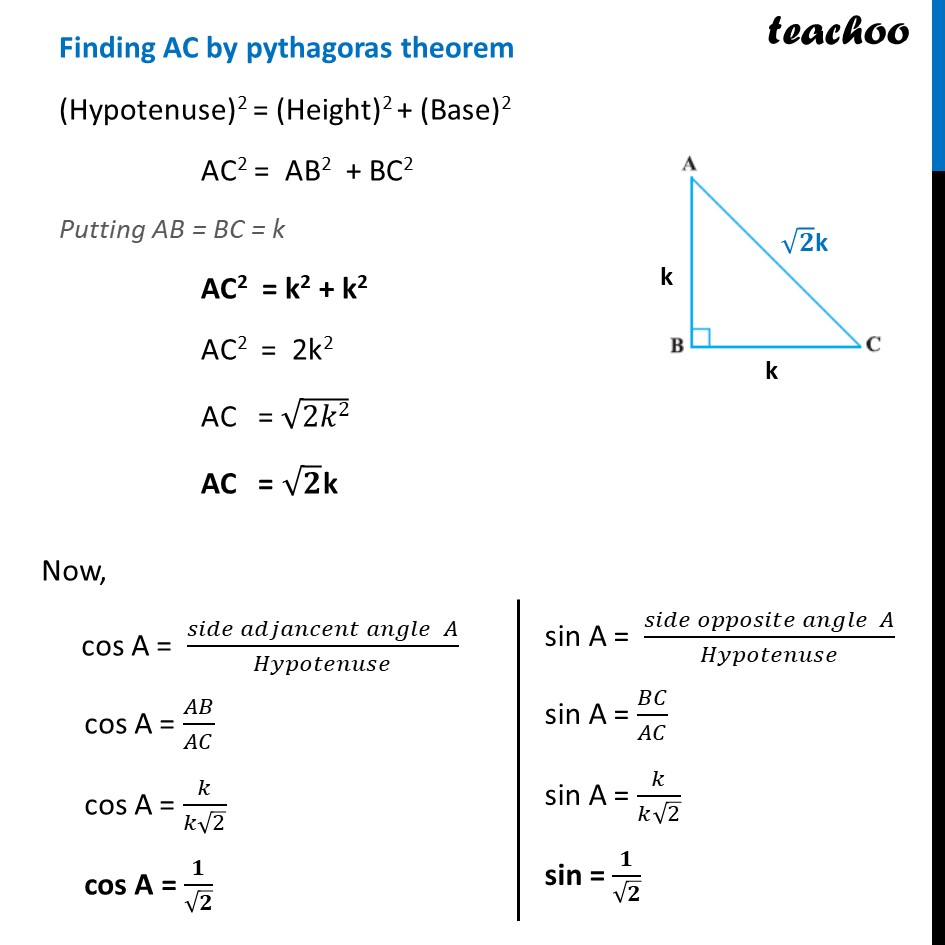
Example 4 In a right triangle ABC, right-angled at B, if tan A = 1, then verify that 2 sin A cos A = 1. In a right angle triangle ABC tan A = 1 (𝑠𝑖𝑑𝑒 𝑜𝑝𝑝𝑜𝑠𝑖𝑡𝑒 𝑡𝑜 𝑎𝑛𝑔𝑙𝑒 𝐴)/(𝑆𝑖𝑑𝑒 𝑎𝑑𝑗𝑎𝑐𝑒𝑛𝑡 𝑡𝑜 𝑎𝑛𝑔𝑙𝑒 𝐴) = 1 𝐵𝐶/𝐴𝐵 = 1 AB = BC Let AB = BC = k Where k is a positive number. Finding AC by pythagoras theorem (Hypotenuse)2 = (Height)2 + (Base)2 AC2 = AB2 + BC2 Putting AB = BC = k AC2 = k2 + k2 AC2 = 2k2 AC = √2𝑘2 AC = √𝟐 "k" Now, cos A = (𝑠𝑖𝑑𝑒 𝑎𝑑𝑗𝑎𝑛𝑐𝑒𝑛𝑡 𝑎𝑛𝑔𝑙𝑒 𝐴)/𝐻𝑦𝑝𝑜𝑡𝑒𝑛𝑢𝑠𝑒 cos A = 𝐴𝐵/𝐴𝐶 cos A = 𝑘/(𝑘√2) cos A = 𝟏/√𝟐 sin A = (𝑠𝑖𝑑𝑒 𝑜𝑝𝑝𝑜𝑠𝑖𝑡𝑒 𝑎𝑛𝑔𝑙𝑒 𝐴)/𝐻𝑦𝑝𝑜𝑡𝑒𝑛𝑢𝑠𝑒 sin A = 𝐵𝐶/𝐴𝐶 sin A = 𝑘/(𝑘√2) sin = 𝟏/√𝟐 We have to find 2 sin A cos A Substituting the value of sin A and cos A = 2 ×1/√2×1/√2 = 𝟐/(√𝟐 × √𝟐) = 2/(√2 )^2 = 2/2 = 1