Ex 8.3, 4 (ix) - Chapter 8 Class 10 Introduction to Trignometry

Transcript

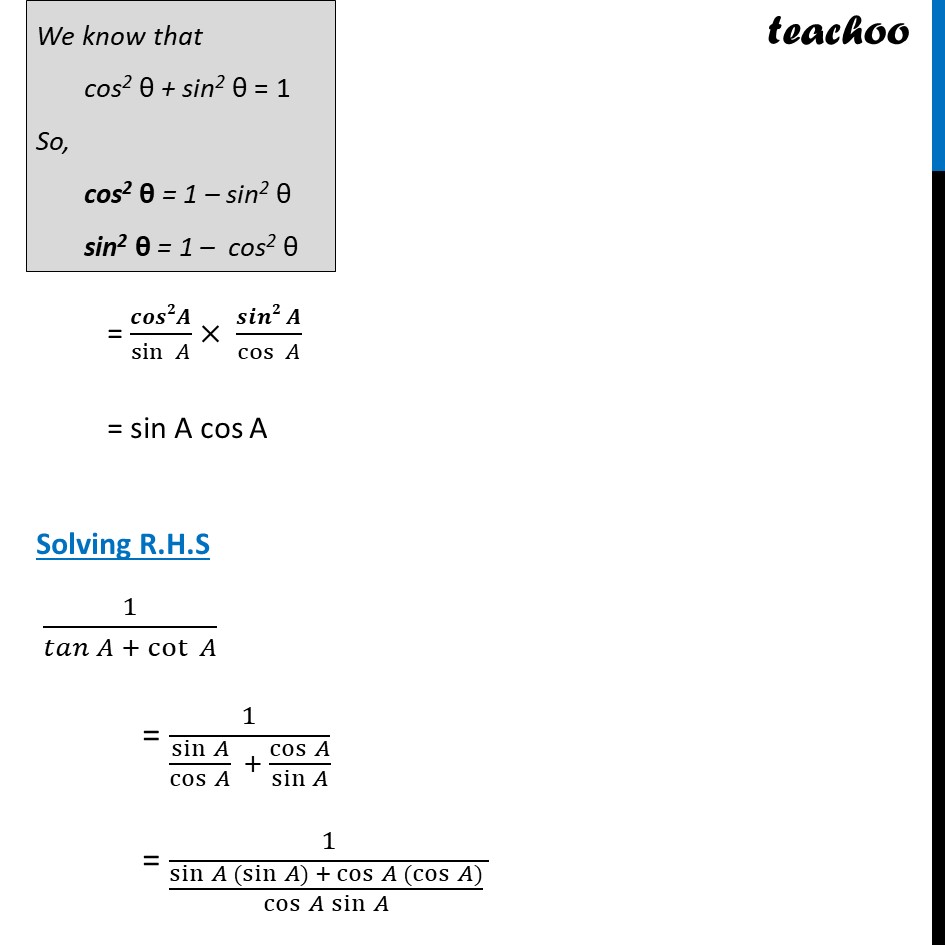
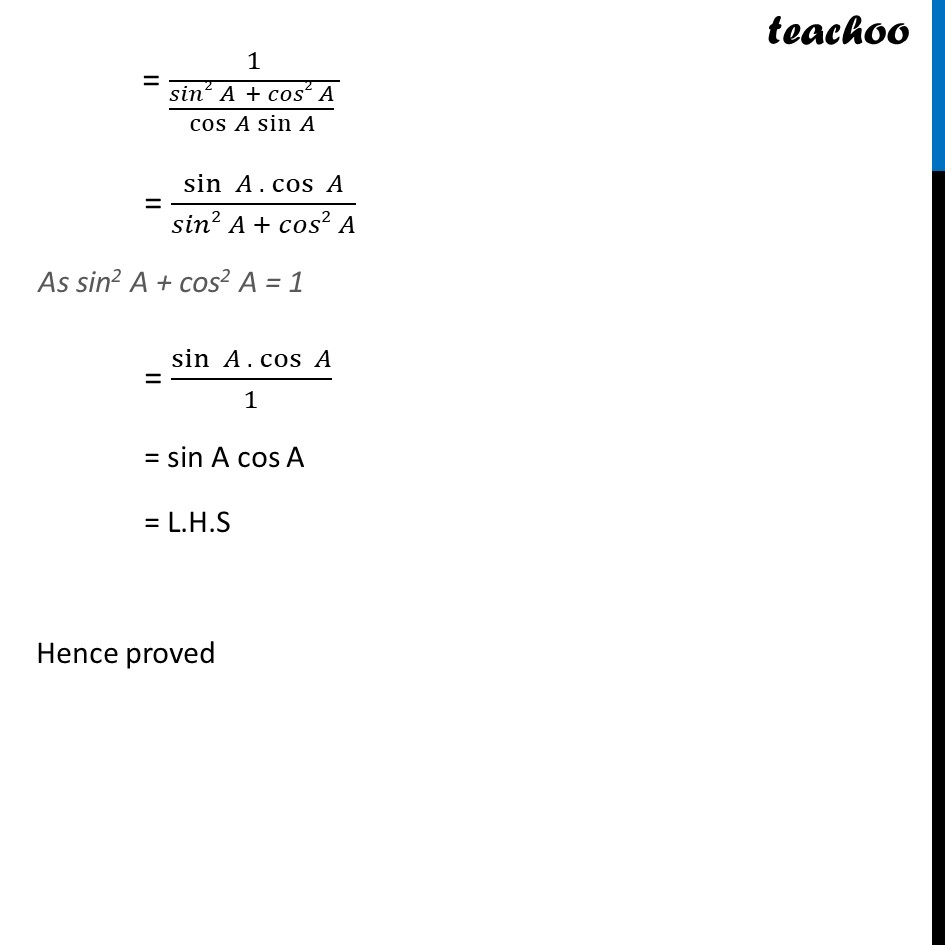
Ex 8.3, 4 Prove the following identities, where the angles involved are acute angles for which the expressions are defined. (ix) (cosec A – sin A)(sec A – cos A) = 1/(𝑡𝑎𝑛 𝐴 +cot⁡ 𝐴) [Hint : Simplify LHS and RHS separately] Solving L.H.S (cosec A – sin A) (sec A – cos A) = (1/sin⁡〖 𝐴〗 − sin⁡𝐴 )(1/cos⁡〖 𝐴〗 − cos⁡ 𝐴) = ((𝟏 − 𝒔𝒊𝒏𝟐 𝑨))/sin⁡〖 𝐴〗 × ((𝟏 − 𝒄𝒐𝒔𝟐 𝑨))/cos⁡〖 𝐴〗 We know that cos2 θ + sin2 θ = 1 So, cos2 θ = 1 – sin2 θ sin2 θ = 1 – cos2 θ = 𝒄𝒐𝒔𝟐𝑨/sin⁡〖 𝐴〗 × (𝒔𝒊𝒏𝟐 𝑨)/cos⁡〖 𝐴〗 = sin A cos A Solving R.H.S 1/(𝑡𝑎𝑛 𝐴 + cot⁡ 𝐴) = 1/(sin⁡𝐴/cos⁡𝐴 + cos⁡𝐴/sin⁡𝐴 ) = 1/(sin⁡〖𝐴 (sin⁡〖𝐴) + cos⁡〖𝐴 (cos⁡〖𝐴)〗 〗 〗 〗/cos⁡〖𝐴 sin⁡𝐴 〗 ) = 1/((𝑠𝑖𝑛2 𝐴 + 𝑐𝑜𝑠2 𝐴)/cos⁡〖𝐴 sin⁡𝐴 〗 ) = 1/((𝑠𝑖𝑛2 𝐴 + 𝑐𝑜𝑠2 𝐴)/cos⁡〖𝐴 sin⁡𝐴 〗 ) = sin⁡〖 𝐴 . cos⁡ 𝐴〗/(𝑠𝑖𝑛2 𝐴 + 𝑐𝑜𝑠2 𝐴) As sin2 A + cos2 A = 1 = sin⁡〖 𝐴 . 〖 cos〗⁡ 𝐴〗/1 = sin A cos A = L.H.S Hence proved