Ex 8.3, 4 (vii) - Chapter 8 Class 10 Introduction to Trignometry

Transcript

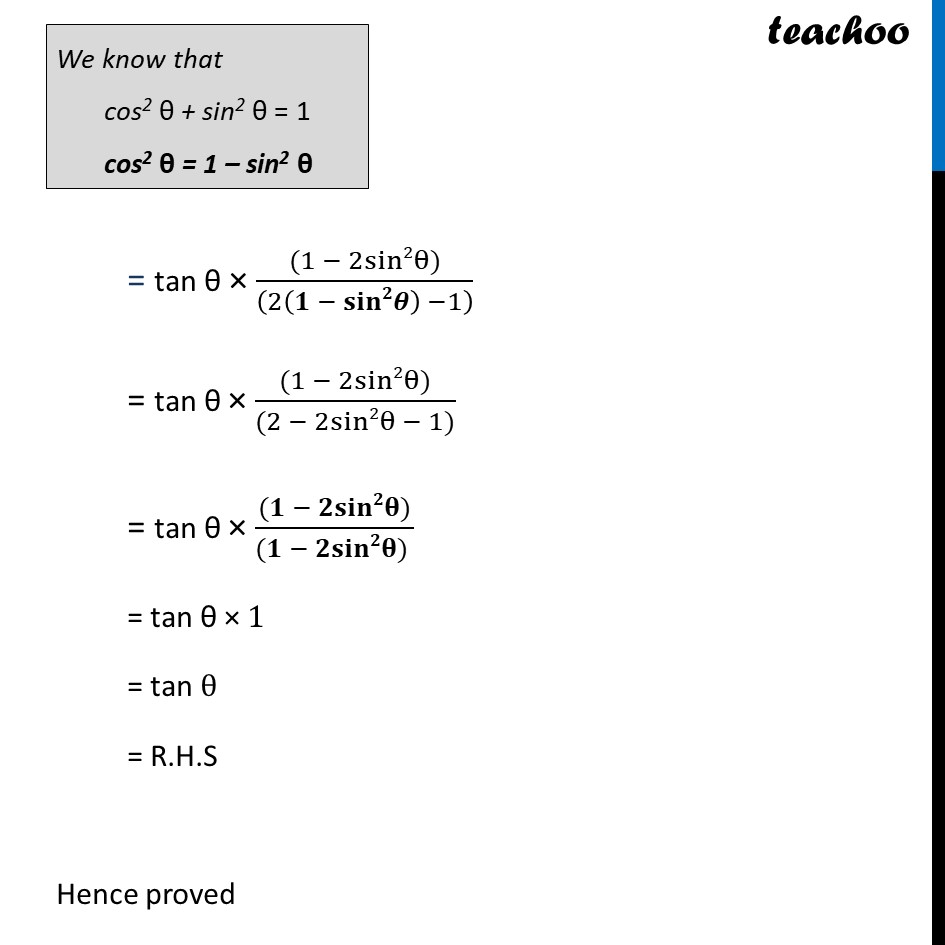
Ex 8.3, 4 Prove the following identities, where the angles involved are acute angles for which the expressions are defined. (vii) (sin θ − 2 sin3 θ)/(2 cos3 θ − cos θ)=tan θ Solving L.H.S (sin θ − 2 sin3θ)/(2 cos3 θ − cosθ) = (𝒔𝒊𝒏⁡𝜽 (1 − 2 sin2 θ))/(𝐜𝐨𝐬 𝛉(2cos2 θ − 1)) = sin⁡θ/(cos θ) × ( (1 − 2sin2θ))/((2cos2θ − 1)) = tan θ × ( (1 − 2sin2θ))/((2𝐜𝐨𝐬𝟐𝛉 − 1)) We know that cos2 θ + sin2 θ = 1 cos2 θ = 1 – sin2 θ = tan θ × ((1 − 2sin2θ))/((2(𝟏 − 𝐬𝐢𝐧𝟐𝜽) −1) ) = tan θ × ((1 − 2sin2θ))/((2 − 2sin2θ − 1)) = tan θ × ((𝟏 − 𝟐𝐬𝐢𝐧𝟐𝛉))/((𝟏 − 𝟐𝐬𝐢𝐧𝟐𝛉) ) = tan θ × 1 = tan θ = R.H.S Hence proved