Ex 5.4, 7 - Chapter 5 Class 8 Squares and Square Roots

Last updated at July 14, 2026 by

Ex 5.4, 7 - In a right triangle ABC, angle B - 90 (a) If AB = 6 cm - Ex 5.4

part 2 - Ex 5.4, 7 - Ex 5.4 - Serial order wise - Chapter 5 Class 8 Squares and Square Roots

 

part 3 - Ex 5.4, 7 - Ex 5.4 - Serial order wise - Chapter 5 Class 8 Squares and Square Roots part 4 - Ex 5.4, 7 - Ex 5.4 - Serial order wise - Chapter 5 Class 8 Squares and Square Roots

 

Remove Ads Share on WhatsApp

Transcript

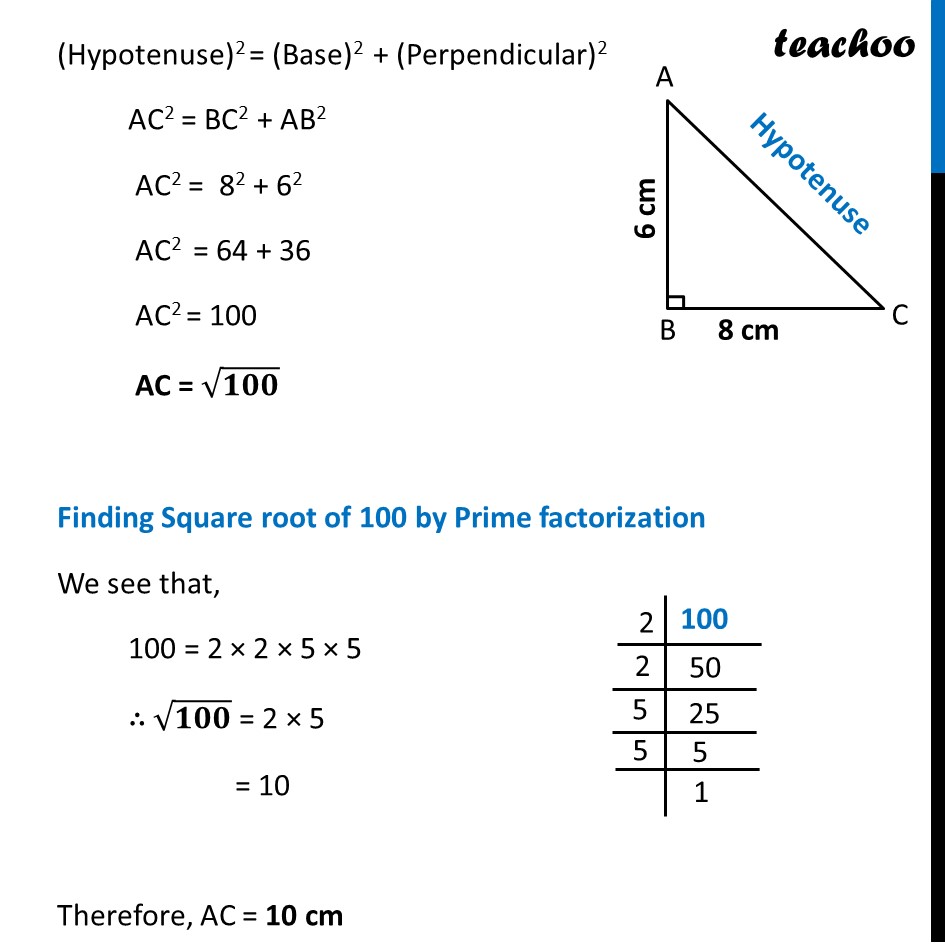
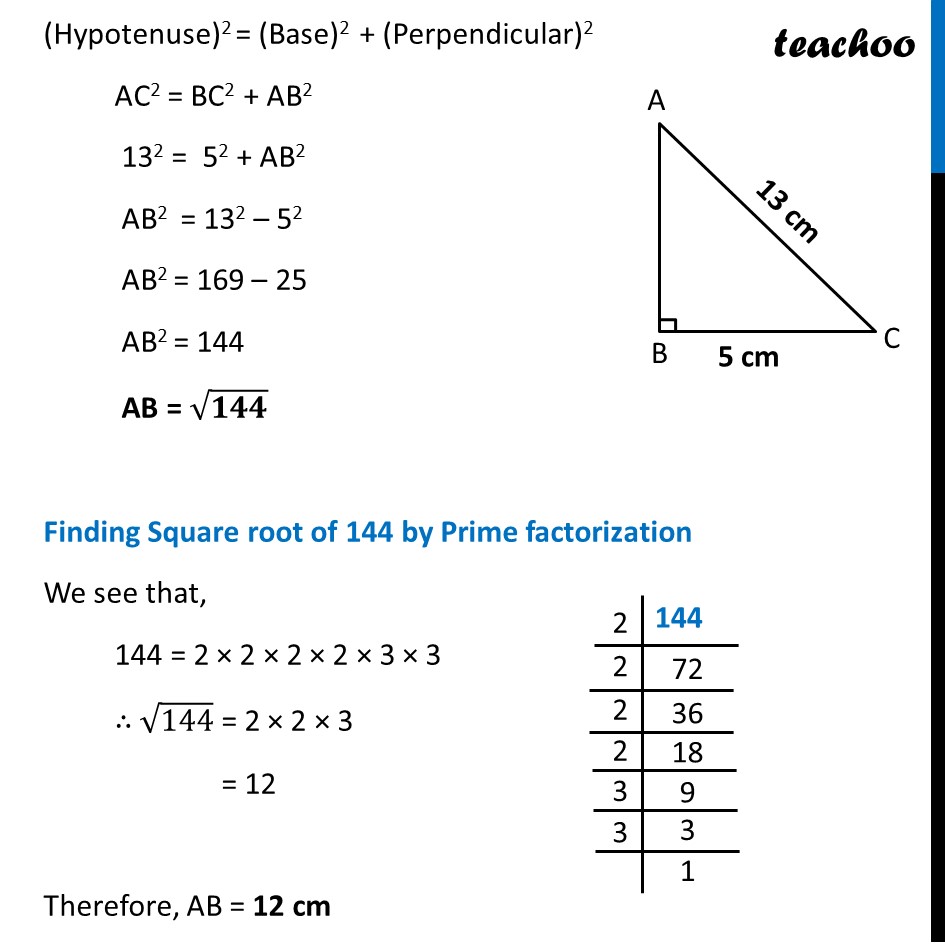
Ex 5.4, 7 In a right triangle ABC, ∠B = 90°. (a) If AB = 6 cm, BC = 8 cm, find AC Given, ∠B = 90° AB = 6 cm BC = 8 cm In right angled triangle ∆ABC, Using Pythagoras theorem, Hypotenuse is the side opposite to right angle. (Hypotenuse)2 = (Base)2 + (Perpendicular)2 AC2 = BC2 + AB2 AC2 = 82 + 62 AC2 = 64 + 36 AC2 = 100 AC = √𝟏𝟎𝟎 Finding Square root of 100 by Prime factorization We see that, 100 = 2 × 2 × 5 × 5 ∴ √𝟏𝟎𝟎 = 2 × 5 = 10 Therefore, AC = 10 cm Ex 5.4, 7 In a right triangle ABC, ∠B = 90°. (b) If AC = 13 cm, BC = 5 cm, find AB Given, ∠B = 90° AC = 13 cm BC = 5 cm In right angled triangle ∆ABC, Using Pythagoras theorem, (Hypotenuse)2 = (Base)2 + (Perpendicular)2 AC2 = BC2 + AB2 132 = 52 + AB2 AB2 = 132 – 52 AB2 = 169 – 25 AB2 = 144 AB = √𝟏𝟒𝟒 Finding Square root of 144 by Prime factorization We see that, 144 = 2 × 2 × 2 × 2 × 3 × 3 ∴ √144 = 2 × 2 × 3 = 12 Therefore, AB = 12 cm

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.