Question 10 (MCQ) - Miscellaneous - Chapter 4 Class 12 Determinants

Transcript

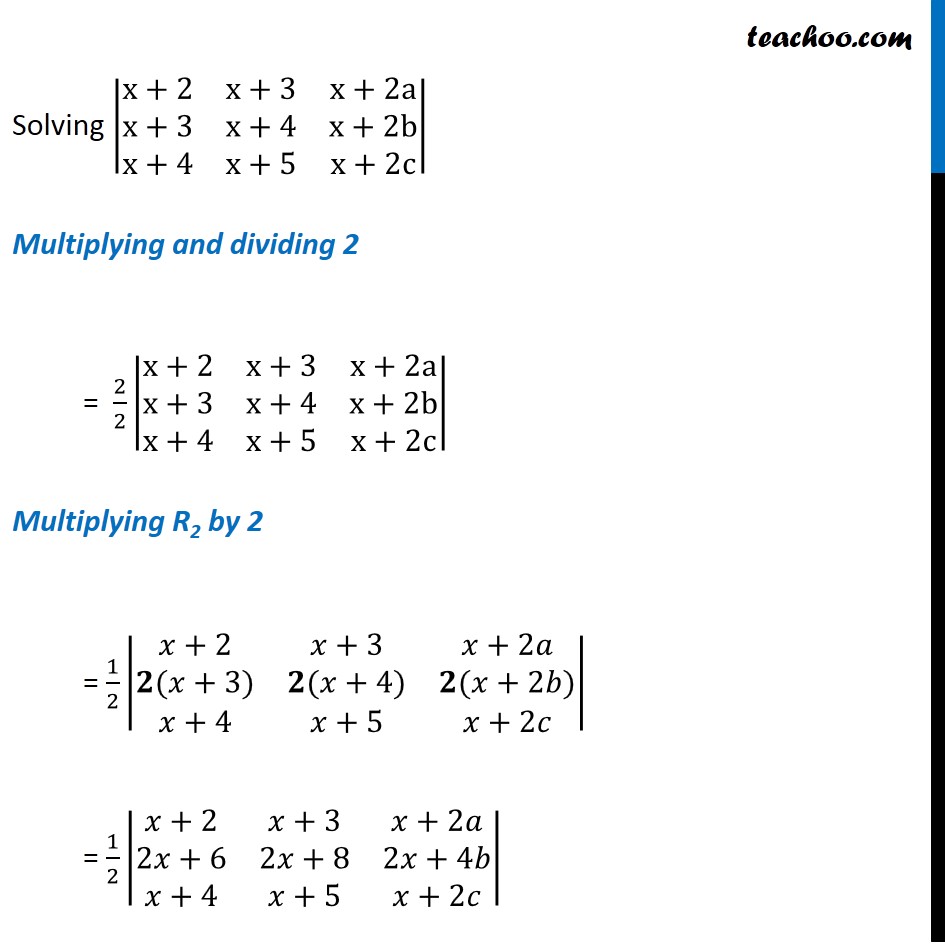
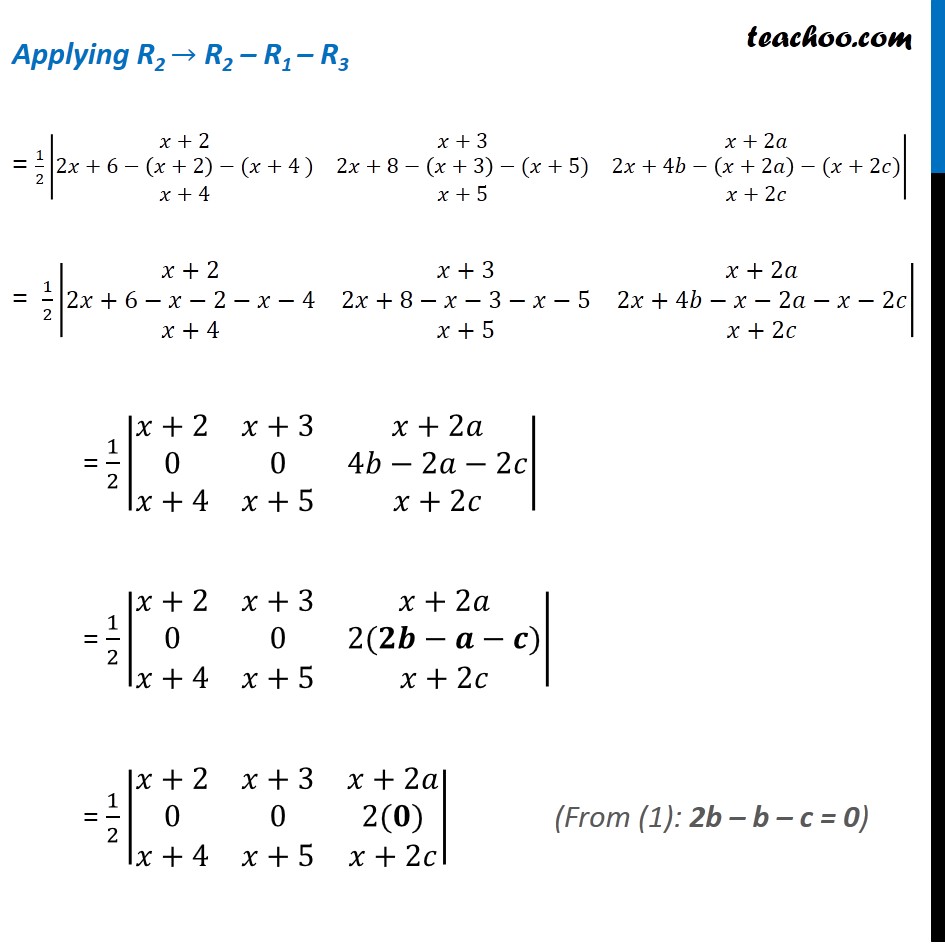
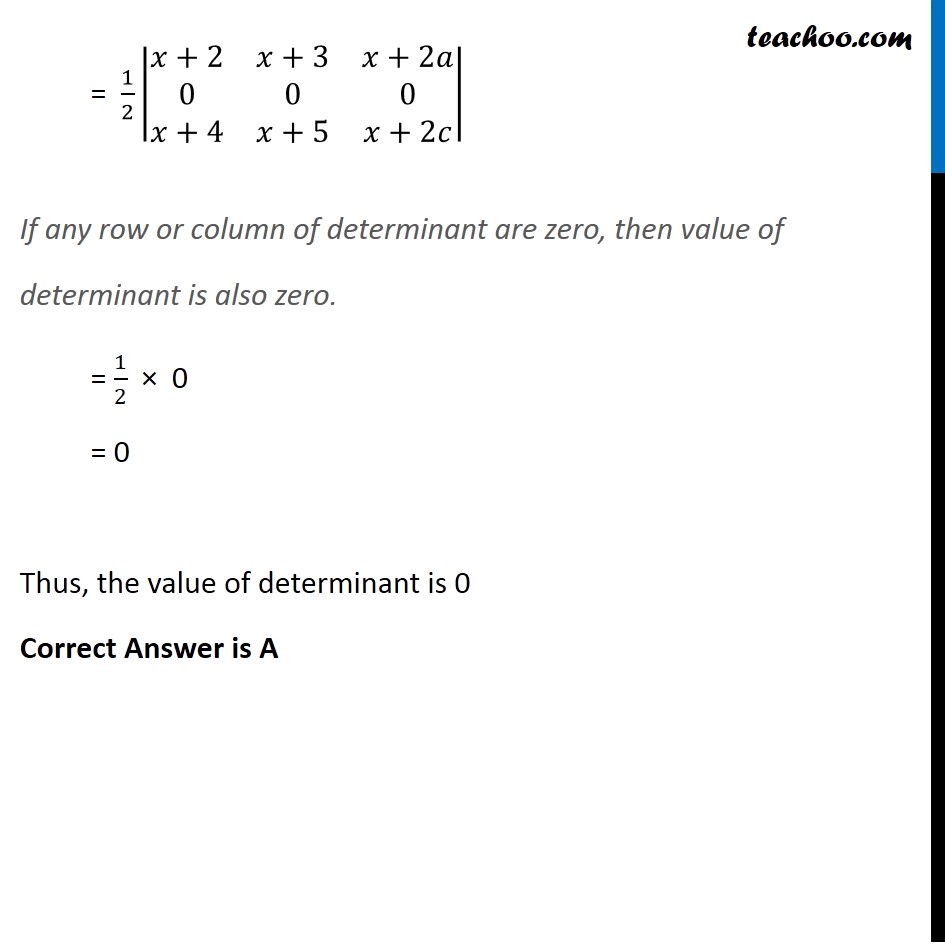
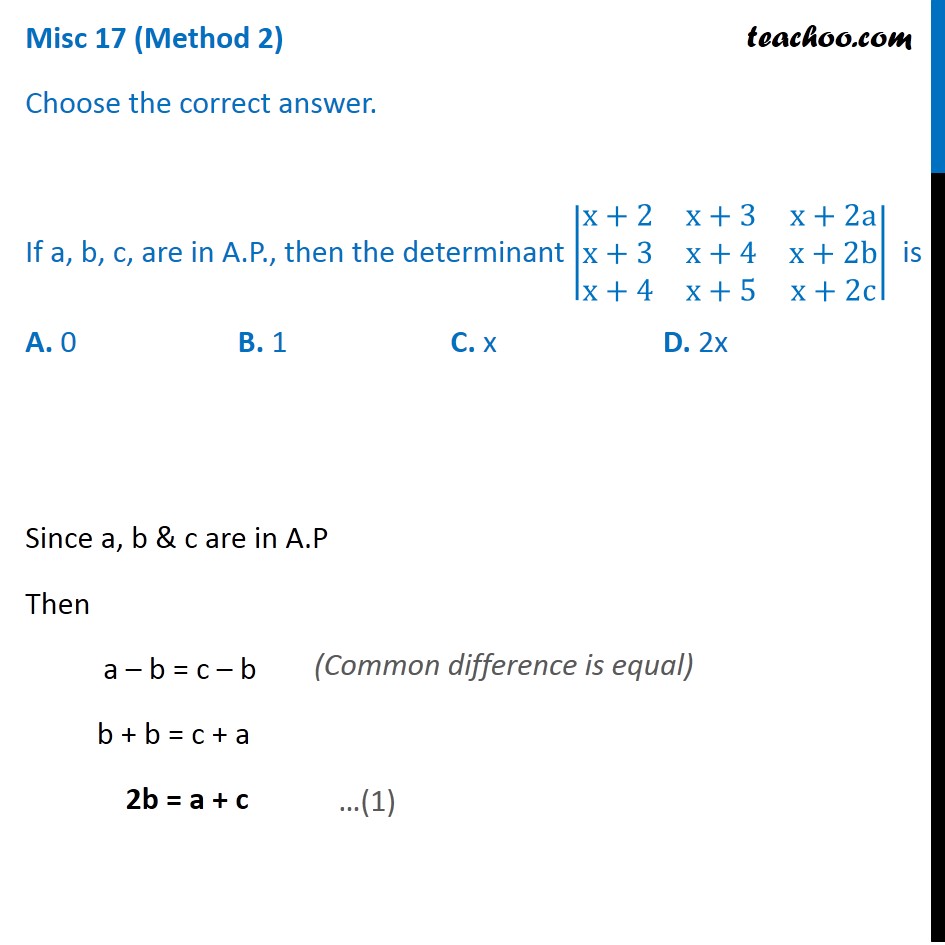
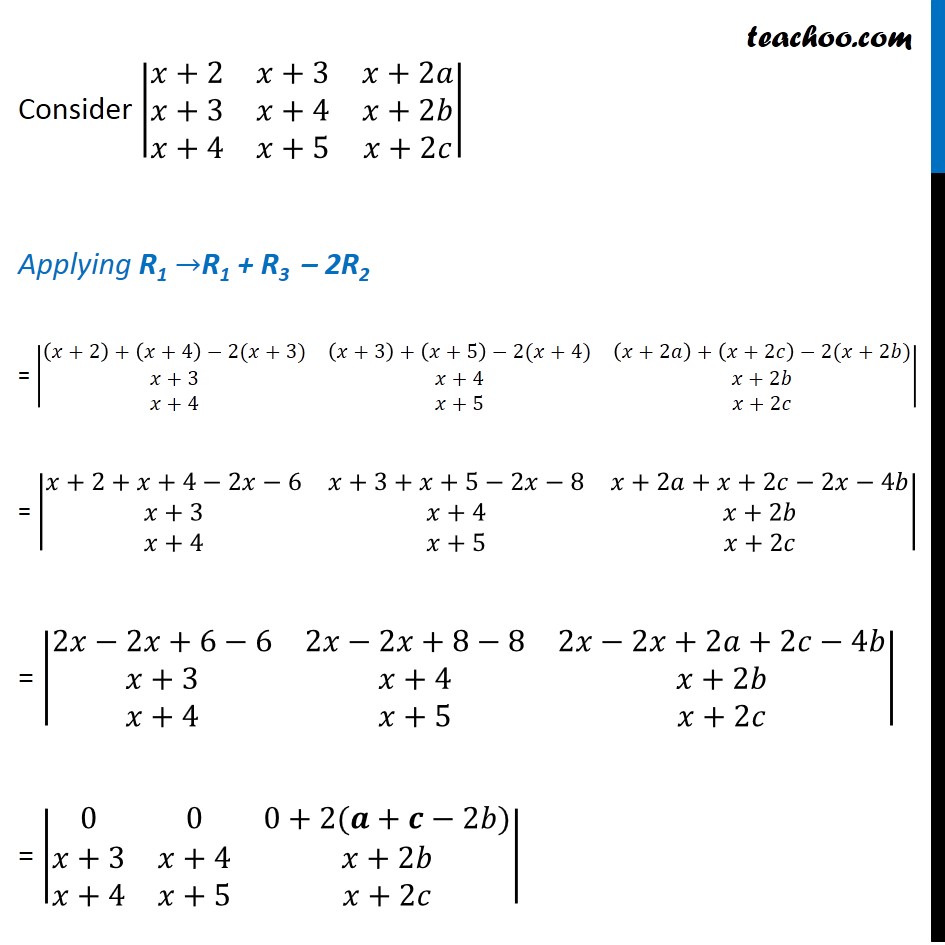
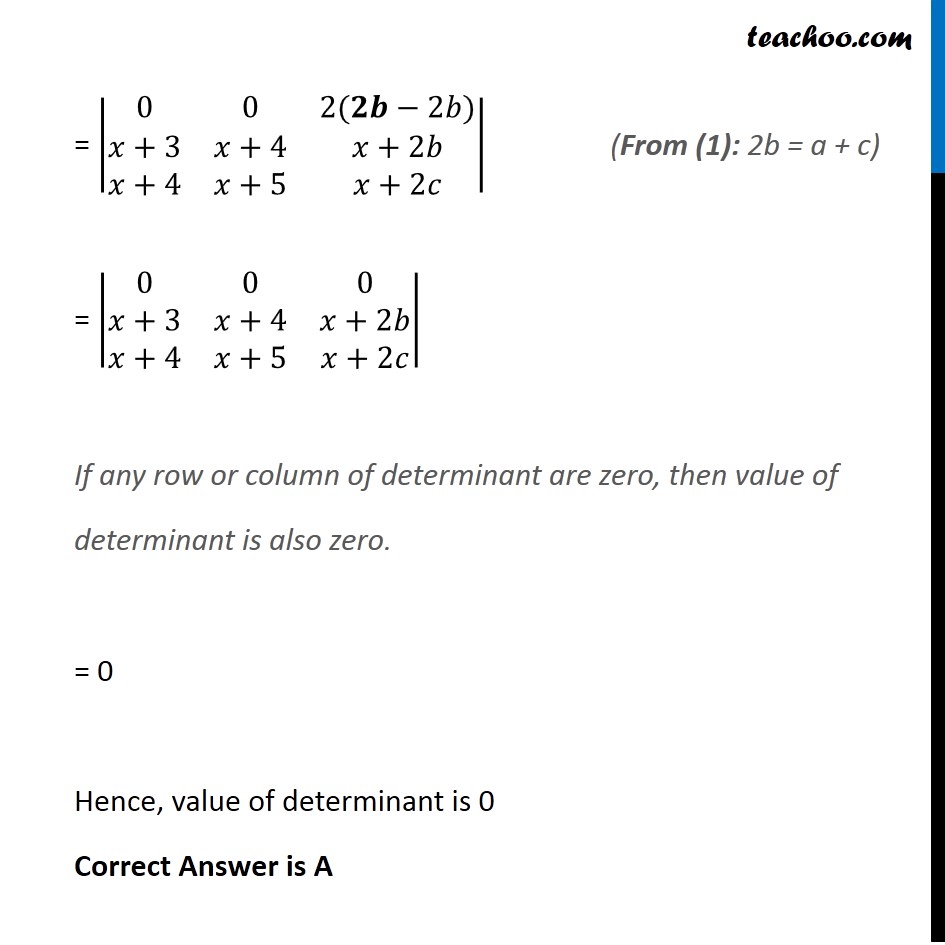
Misc 17 (Method 1) Choose the correct answer. If a, b, c, are in A.P., then the determinant |■8(𝑥+2&𝑥+3&𝑥+2𝑎@𝑥+3&𝑥+4&𝑥+2𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| is A. 0 B. 1 C. x D. 2x Since a, b & c are in A.P Then, b – a = c – b b – a – c + b = 0 2b – a – c = 0 (Common difference is equal) …(1) Solving |■8(x+2&x+3&x+2a@x+3&x+4&x+2b@x+4&x+5&x+2c)| Multiplying and dividing 2 = 2/2 |■8(x+2&x+3&x+2a@x+3&x+4&x+2b@x+4&x+5&x+2c)| Multiplying R2 by 2 = 1/2 |■8(𝑥+2&𝑥+3&𝑥+2𝑎@𝟐(𝑥+3)&𝟐(𝑥+4)&𝟐(𝑥+2𝑏)@𝑥+4&𝑥+5&𝑥+2𝑐)| = 1/2 |■8(𝑥+2&𝑥+3&𝑥+2𝑎@2𝑥+6&2𝑥+8&2𝑥+4𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| Applying R2 → R2 – R1 – R3 = 1/2 |■8(𝑥+2&𝑥+3&𝑥+2𝑎@2𝑥+6−(𝑥+2)−(𝑥+4 )&2𝑥+8−(𝑥+3)−(𝑥+5)&2𝑥+4𝑏−(𝑥+2𝑎)−(𝑥+2𝑐)@𝑥+4&𝑥+5&𝑥+2𝑐)| = 1/2 |■8(𝑥+2&𝑥+3&𝑥+2𝑎@2𝑥+6−𝑥−2−𝑥−4&2𝑥+8−𝑥−3−𝑥−5&2𝑥+4𝑏−𝑥−2𝑎−𝑥−2𝑐@𝑥+4&𝑥+5&𝑥+2𝑐)| = 1/2 |■8(𝑥+2&𝑥+3&𝑥+2𝑎@0&0&4𝑏−2𝑎−2𝑐@𝑥+4&𝑥+5&𝑥+2𝑐)| = 1/2 |■8(𝑥+2&𝑥+3&𝑥+2𝑎@0&0&2(𝟐𝒃−𝒂−𝒄)@𝑥+4&𝑥+5&𝑥+2𝑐)| = 1/2 |■8(𝑥+2&𝑥+3&𝑥+2𝑎@0&0&2(𝟎)@𝑥+4&𝑥+5&𝑥+2𝑐)| (From (1): 2b – b – c = 0) = 1/2 |■8(𝑥+2&𝑥+3&𝑥+2𝑎@0&0&0@𝑥+4&𝑥+5&𝑥+2𝑐)| If any row or column of determinant are zero, then value of determinant is also zero. = 1/2 × 0 = 0 Thus, the value of determinant is 0 Correct Answer is A Misc 17 (Method 2) Choose the correct answer. If a, b, c, are in A.P., then the determinant |■8(x+2&x+3&x+2a@x+3&x+4&x+2b@x+4&x+5&x+2c)| is A. 0 B. 1 C. x D. 2x Since a, b & c are in A.P Then a – b = c – b b + b = c + a 2b = a + c (Common difference is equal) …(1) Consider |■8(𝑥+2&𝑥+3&𝑥+2𝑎@𝑥+3&𝑥+4&𝑥+2𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| Applying R1 →R1 + R3 – 2R2 = |■8((𝑥+2)+(𝑥+4)−2(𝑥+3)&(𝑥+3)+(𝑥+5)−2(𝑥+4)&(𝑥+2𝑎)+(𝑥+2𝑐)−2(𝑥+2𝑏)@𝑥+3&𝑥+4&𝑥+2𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| = |■8(𝑥+2+𝑥+4−2𝑥−6&𝑥+3+𝑥+5−2𝑥−8&𝑥+2𝑎+𝑥+2𝑐−2𝑥−4𝑏@𝑥+3&𝑥+4&𝑥+2𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| = |■8(2𝑥−2𝑥+6−6&2𝑥−2𝑥+8−8&2𝑥−2𝑥+2𝑎+2𝑐−4𝑏@𝑥+3&𝑥+4&𝑥+2𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| = |■8(0&0&0+2(𝒂+𝒄−2𝑏)@𝑥+3&𝑥+4&𝑥+2𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| = |■8(0&0&2(𝟐𝒃−2𝑏)@𝑥+3&𝑥+4&𝑥+2𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| = |■8(0&0&0@𝑥+3&𝑥+4&𝑥+2𝑏@𝑥+4&𝑥+5&𝑥+2𝑐)| If any row or column of determinant are zero, then value of determinant is also zero. = 0 Hence, value of determinant is 0 Correct Answer is A (From (1): 2b = a + c)