




Ex 10.1
Last updated at Dec. 16, 2024 by Teachoo







Transcript
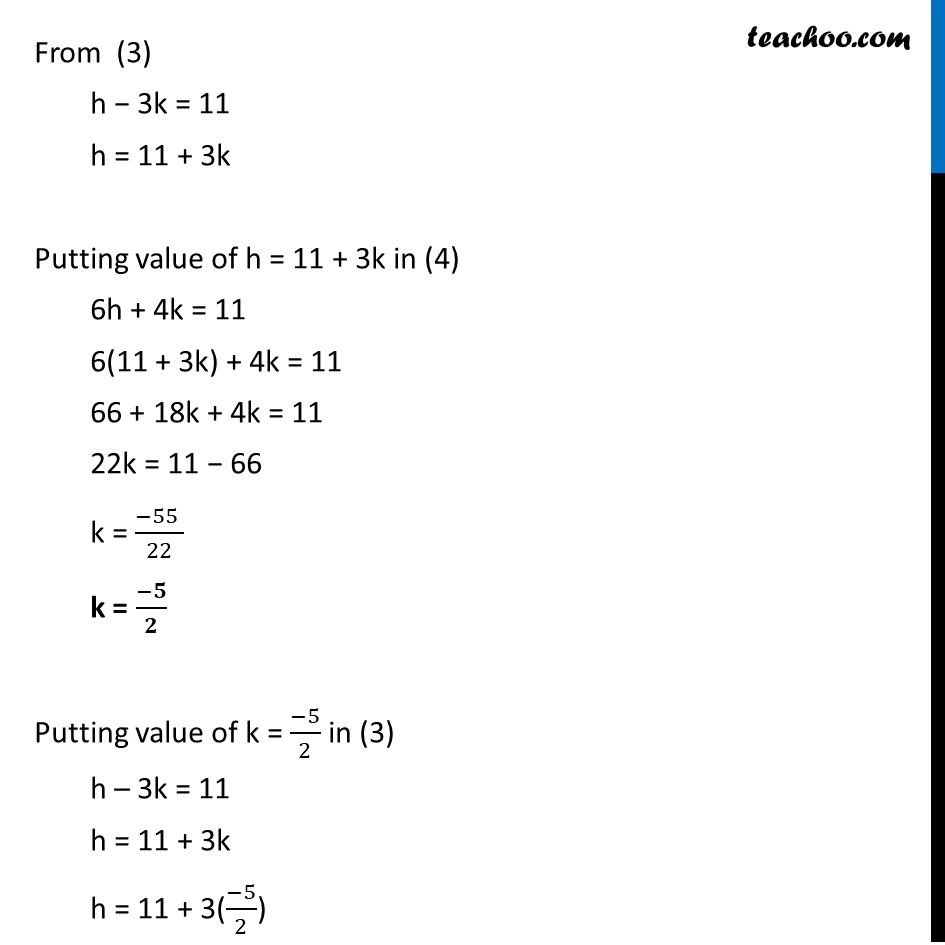
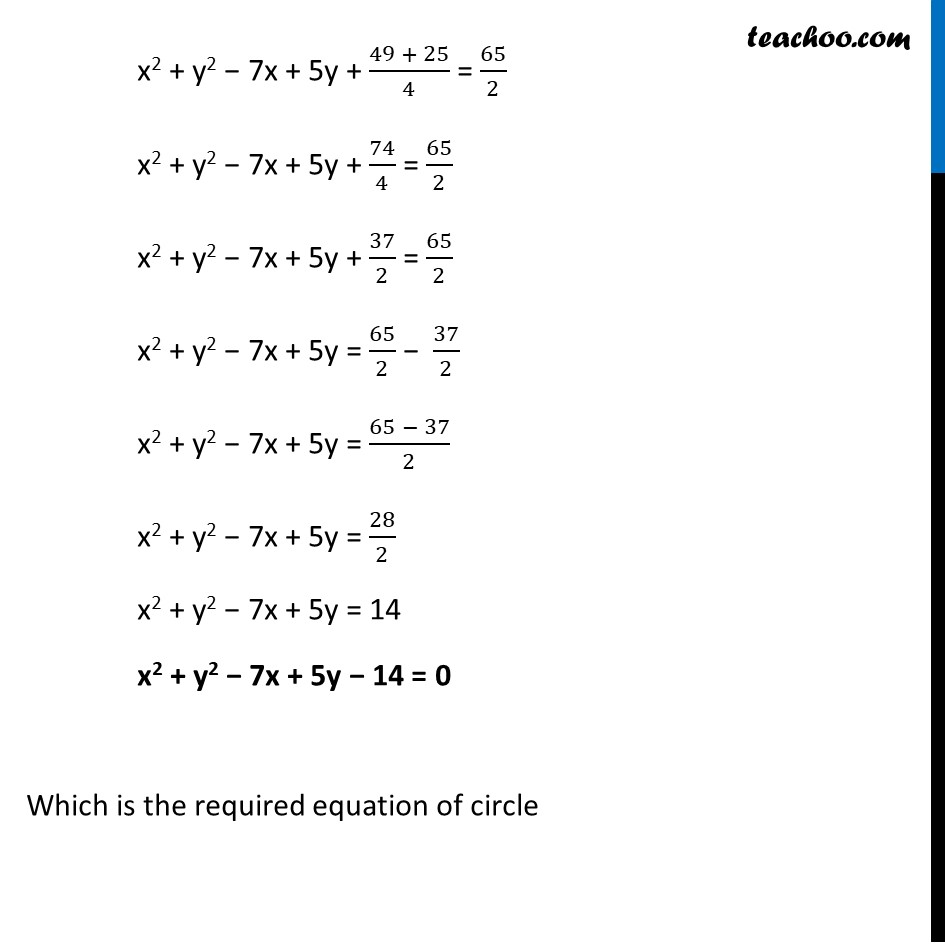
Ex 10.1, 11 Find the equation of the circle passing through the points (2, 3) and (–1, 1) and whose centre is on the line x – 3y – 11 = 0. Let the equation of the circle be (x – h)2 + (y – k)2 = r2. Since the circle passes through points (2, 3) Point (2, 3) will satisfy the equation of circle Putting x = 2, y = 3 in (A) (2 – h)2 + (3 – k)2 = r2 (2)2 + (h)2 − 2(2)(h) + (3)2 + k2 − 2(3)(k) = r2 4 + h2 − 4h + 9 + k2 − 6k = r2 h2 + k2 − 4h − 6k + 4 + 9 = r2 h2 + k2 − 4h − 6k + 13 = r2 Also, circle passes through the (−1, 1) Point (−1, 1) will satisfy the equation of circle Putting x = –1, y = –1 in (A) (−1 − h)2 + (1 − k)2 = r2 (−1)2 (1 + h) 2 + (1 − k) 2 = r2 (1 + h) 2 + (1 − k) 2 = r2 (1)2 + h2 + 2h + 1 + k2 − 2k = r2 1 + h2 + 2h + 1 + k2 − 2k = r2 h2 + k2 + 2h − 2k + 1 + 1 = r2 h2 + k2 + 2h − 2k + 2 = r2 Since centre (h, k) lie on the circle x − 3y − 11 = 0 Point (h, k) will satisfy the equation of line x − 3y − 11 = 0 So, h − 3k − 11 = 0 h − 3k = 11 Solving (1) & (2) h2 + k2 − 4h − 6k + 13 = r2 …(1) h2 + k2 + 2h − 2k + 2 = r2 …(2) Subtracting (1) from (2) (h2 + k2 − 4h − 6k + 13) − (h2 + k2+ 2h − 2k + 2) = r2 − r2 h2 + k2 − 4h − 6k + 13 − h2 − k2 − 2h + 2k − 2 = 0 h2 − h2 + k2 − k2 − 4h − 6k + 13 − 2h + 2k − 2 = 0 0 + 0 − 6h − 4k + 11 = 0 −6h − 4k = −11 6h + 4k = 11 Now our equations are h − 3k = 11 …(3) 6h + 4k = 11 …(4) From (3) h − 3k = 11 h = 11 + 3k Putting value of h = 11 + 3k in (4) 6h + 4k = 11 6(11 + 3k) + 4k = 11 66 + 18k + 4k = 11 22k = 11 − 66 k = −55 22 k = −𝟓𝟐 Putting value of k = −52 in (3) h – 3k = 11 h = 11 + 3k h = 11 + 3(−52) h = 22 − 152 h = 𝟕 𝟐 Hence h = 72 & k = −52 Putting value of (h, k) = 72, −52 in (1) 2 – 722 + 3 – −522 = r2 4 − 722 + 6 + 522 = r2 −322 + 1122 = r2 94 + 1214 = r2 9 + 1214 = r2 1304 = r2 652 = r2 r2 = 𝟔𝟓𝟐 Now putting value of h, k & r2 in (A) (x − h)2 + (y − k)2 = r2 x − 722 + y − −522 = 652 x − 722 + y +522 = 652 x2 + 722 − 2(x) 72 + y2 + 254 + 5y = 652 x2 + 494 − 7x + y2 + 254 + 5y = 652 x2 + y2 − 7x + 5y + 494 + 254 = 652 x2 + y2 − 7x + 5y + 49 + 254 = 652 x2 + y2 − 7x + 5y + 744 = 652 x2 + y2 − 7x + 5y + 372 = 652 x2 + y2 − 7x + 5y = 652 − 372 x2 + y2 − 7x + 5y = 65 − 372 x2 + y2 − 7x + 5y = 282 x2 + y2 − 7x + 5y = 14 x2 + y2 − 7x + 5y − 14 = 0 Which is the required equation of circle