CBSE Class 10 Sample Paper for 2023 Boards - Maths Standard

Practice Questions CBSE - Maths Class 10 (2023 Boards) →
Class 10
Solutions of Sample Papers for Class 10 Boards

Question 2 - CBSE Class 10 Sample Paper for 2023 Boards - Maths Standard - Solutions of Sample Papers for Class 10 Boards

Last updated at August 10, 2026 by

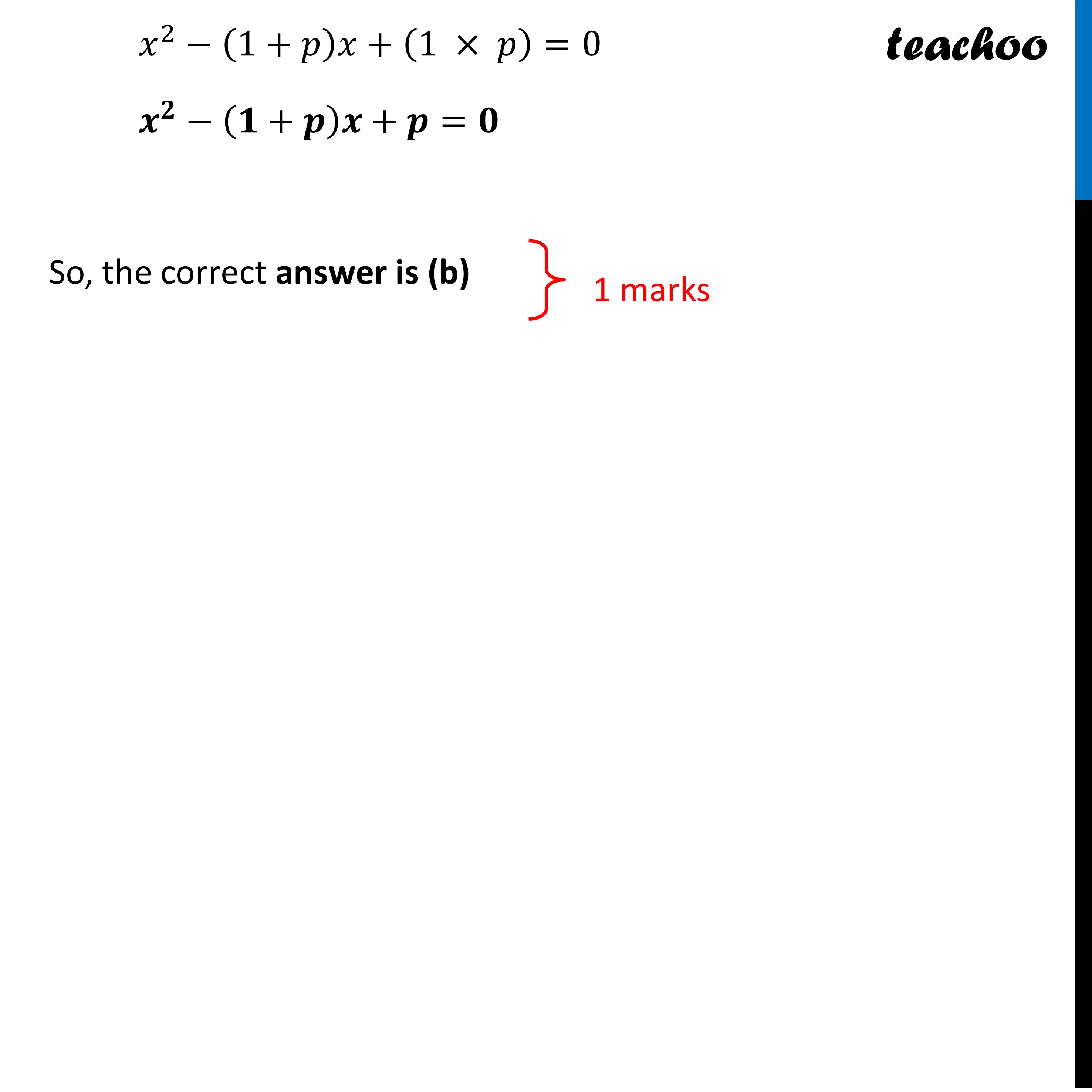
Let p be a prime number. The quadratic equation having its roots as factors of p is

(a) x 2 – px + p = 0 

(b) x 2 – (p + 1)x + p = 0

(c) x 2 + (p + 1)x + p = 0 

(d) x 2 – px + p + 1=0

 

[MCQ] Let p be a prime number. The quadratic equation having its roots - CBSE Class 10 Sample Paper for 2023 Boards - Maths Standard

part 2 - Question 2 - CBSE Class 10 Sample Paper for 2023 Boards - Maths Standard - Solutions of Sample Papers for Class 10 Boards - Class 10

Remove Ads

Transcript

Question 2 Let p be a prime number. The quadratic equation having its roots as factors of p is (a) x2 – px + p = 0 (b) x2 – (p + 1)x + p = 0 (c) x2 + (p + 1)x + p = 0 (d) x2 – px + p + 1=0We need to find quadratic equation having its roots as factors of p is Since p is a prime number Factors of p = 1, p Thus, we need to find quadratic equation with roots 1 and p Required quadratic equation is x2 − (Sum of roots)x + Product of roots = 0 Putting values 𝑥^2−(1+𝑝)𝑥+(1 × 𝑝)=0 𝒙^𝟐−(𝟏+𝒑)𝒙+𝒑=𝟎 So, the correct answer is (b)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.