Question 1 (iv) - Cross Multiplication Method - Chapter 3 Class 10 Pair of Linear Equations in Two Variables

Transcript

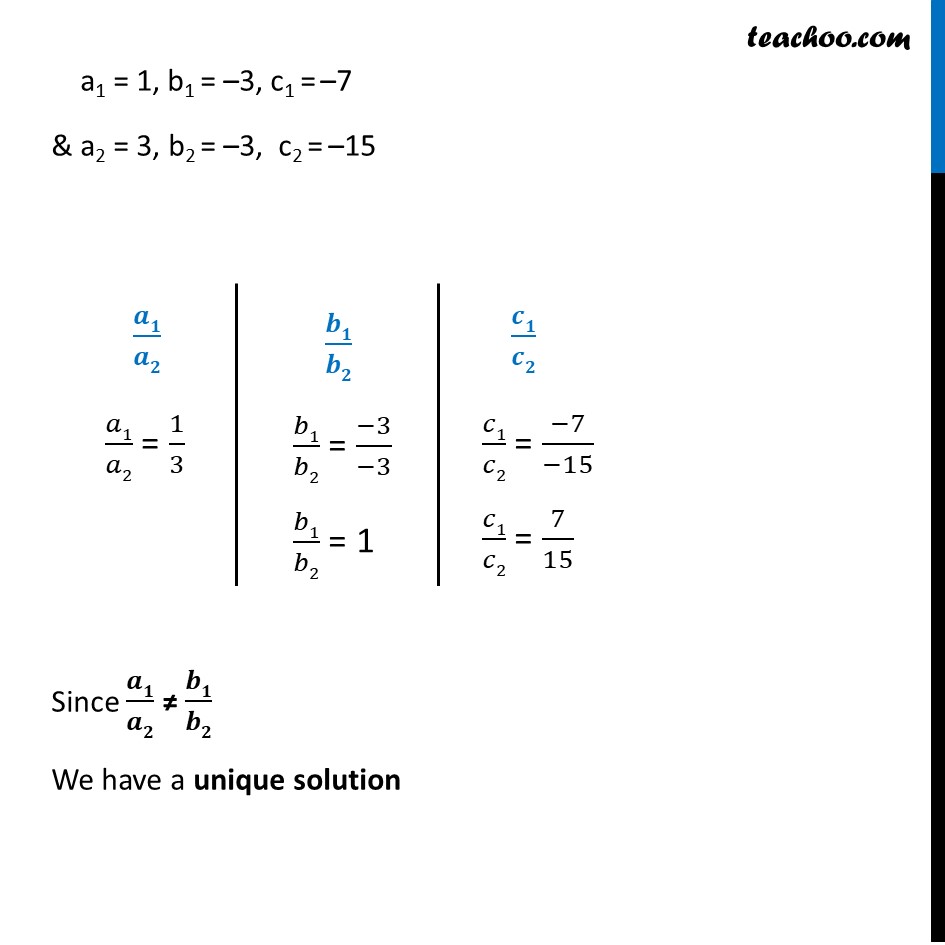
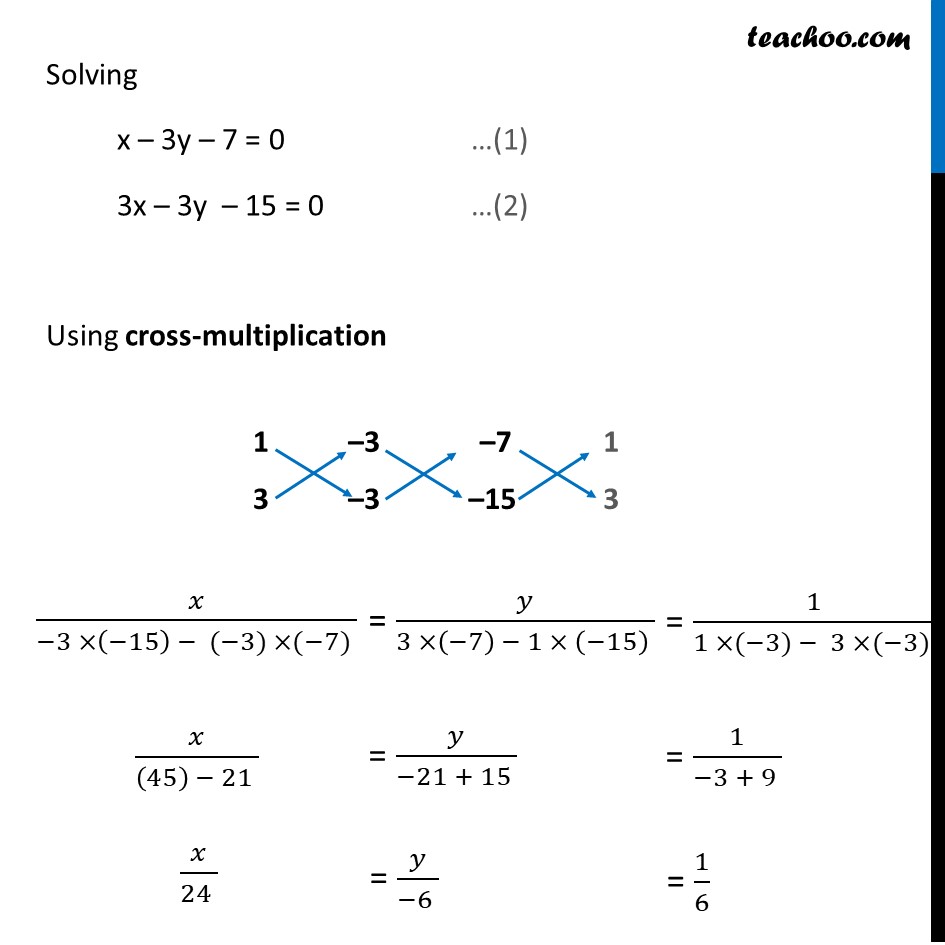
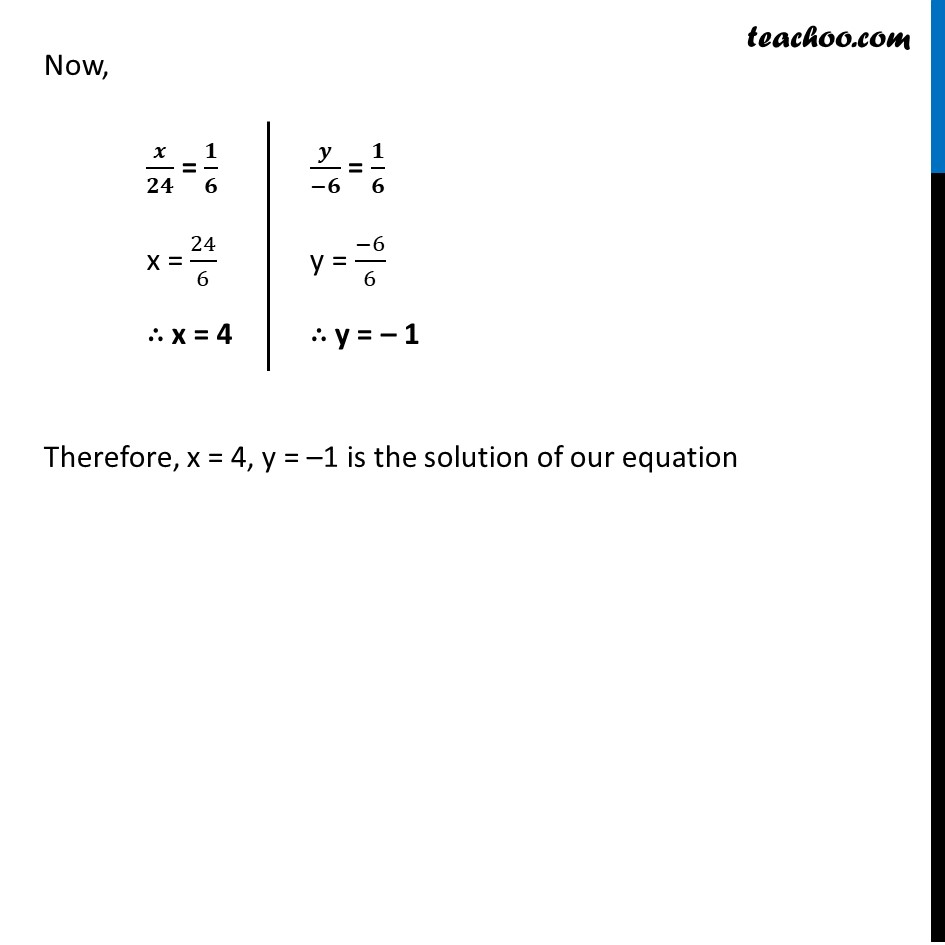
Ex 3.5 ,1 Which of the following pairs of linear equations has unique solution, no solution, or infinitely many solutions. In case there is a unique solution, find it by using cross multiplication method (iv) x – 3y – 7 = 0 3x – 3y – 15 = 0 x – 3y – 7 = 0 3x – 3y – 15 = 0 x – 3y – 7 = 0 Comparing with a1x + b1y + c1 = 0 ∴ a1 = 1, b1 = –3, c1 = –7 3x – 3y – 15 = 0 Comparing with a2x + b2y + c2 = 0 ∴ a2 = 3, b2 = –3, c2 = –15 a1 = 1, b1 = –3, c1 = –7 & a2 = 3, b2 = –3, c2 = –15 𝒂𝟏/𝒂𝟐 𝑎1/𝑎2 = 1/3 𝒃𝟏/𝒃𝟐 𝑏1/𝑏2 = (−3)/(−3) 𝑏1/𝑏2 = 1 𝒄𝟏/𝒄𝟐 𝑐1/𝑐2 = (−7)/(−15) 𝑐1/𝑐2 = 7/15 Since 𝒂𝟏/𝒂𝟐 ≠ 𝒃𝟏/𝒃𝟐 We have a unique solution Solving x – 3y – 7 = 0 …(1) 3x – 3y – 15 = 0 …(2) Using cross-multiplication 𝑥/(−3 ×(−15) − (−3) ×(−7) ) = 𝑦/(3 ×(−7) − 1 × (−15) ) = 1/(1 ×(−3) − 3 ×(−3) ) 𝑥/((45) − 21 ) = 𝑦/(−21 + 15 ) = 1/(−3 + 9 ) 𝑥/(24 ) = 𝑦/(−6 ) = 1/6 Now, 𝒙/𝟐𝟒 = 𝟏/𝟔 x = 24/6 ∴ x = 4 𝒚/(−𝟔) = 𝟏/𝟔 y = (−6)/6 ∴ y = – 1 Therefore, x = 4, y = –1 is the solution of our equation