CBSE Class 12 Sample Paper for 2020 Boards
You are here
CBSE Class 12 Sample Paper for 2020 Boards
Last updated at Dec. 16, 2024 by Teachoo
Note : - This is similar to Example 30 of NCERT – Chapter 7 Class 12
Check the answer here https://www.teachoo.com/4811/727/Example-30---Evaluate-integral--1----2--x3---x--dx/category/Examples/
Transcript
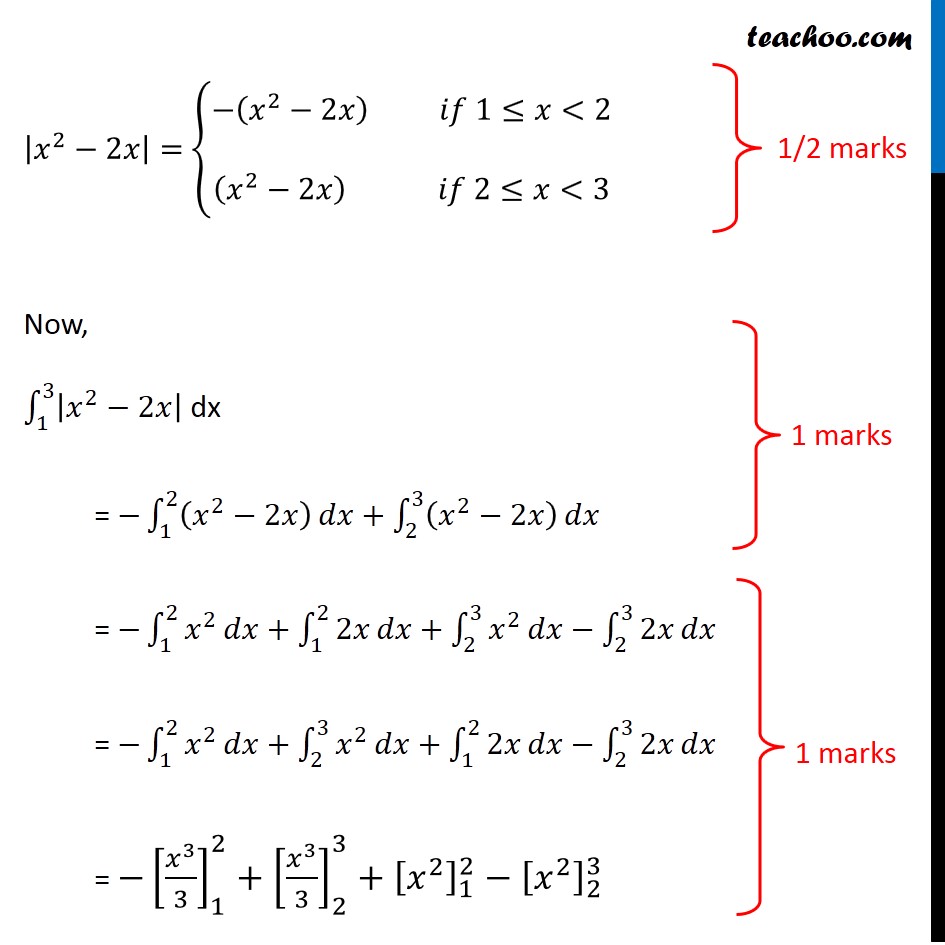
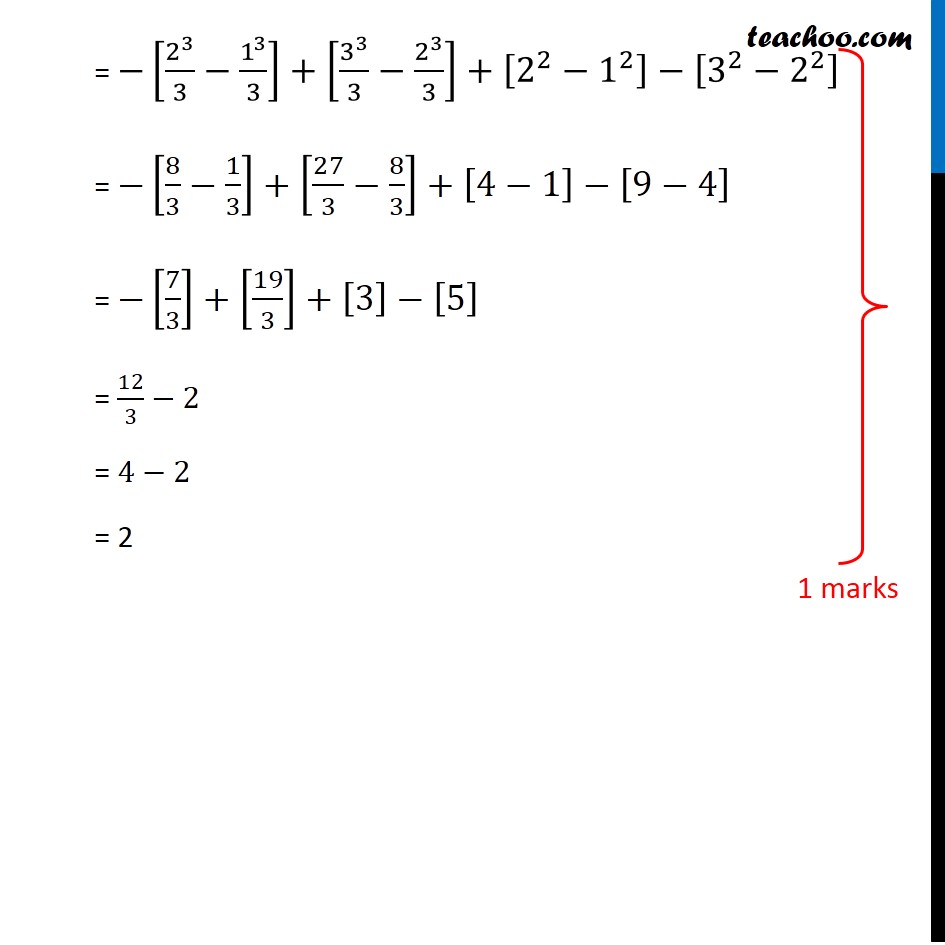
Question 30 Evaluate ∫ 3 1 |𝑥^2−2𝑥| dx |𝑥^2−2𝑥|=|𝑥(𝑥−2)| =|𝑥| |𝑥−2| Thus, 𝑥=0, 𝑥=2 Since our integration is from 1 to 3, we ignore x = 0 ∴ |𝑥^2−2𝑥|= {(𝑥×−(𝑥−2) 𝑖𝑓 1≤𝑥<2𝑥×(𝑥−2) 𝑖𝑓 2≤𝑥<3)┤ |𝑥^2−2𝑥|= {(−(𝑥^2−2𝑥) 𝑖𝑓 1≤𝑥<2(𝑥^2−2𝑥) 𝑖𝑓 2≤𝑥<3)┤ Now, ∫_1^3 |𝑥^2−2𝑥| dx = −∫_1^2▒(𝑥^2−2𝑥) 𝑑𝑥+∫_2^3▒(𝑥^2−2𝑥) 𝑑𝑥 = −∫_1^2▒𝑥^2 𝑑𝑥+∫_1^2▒2𝑥 𝑑𝑥+∫_2^3▒𝑥^2 𝑑𝑥−∫_2^3▒2𝑥 𝑑𝑥 = −∫_1^2▒𝑥^2 𝑑𝑥+∫_2^3▒𝑥^2 𝑑𝑥+∫_1^2▒2𝑥 𝑑𝑥−∫_2^3▒2𝑥 𝑑𝑥 = −[𝑥^3/3]_1^2+[𝑥^3/3]_2^3+[𝑥^2 ]_1^2−[𝑥^2 ]_2^3 = −[2^3/3−1^3/3]+[3^3/3−2^3/3]+[2^2−1^2 ]−[3^2−2^2 ] = −[8/3−1/3]+[27/3−8/3]+[4−1]−[9−4] = −[7/3]+[19/3]+[3]−[5] = 12/3−2 = 4−2 = 2
