Theorem 10.11 - Chapter 10 Class 9 Circles (Term 2)
Last updated at Aug. 13, 2018 by Teachoo
Theorems
Theorem 10.1
Theorem 10.2 Important
Theorem 10.3 Important
Theorem 10.4
Theorem 10.5 Deleted for CBSE Board 2022 Exams
Theorem 10.6 Important
Theorem 10.7
Theorem 10.8 Important
Theorem 10.9
Theorem 10.10 Important
Theorem 10.11 You are here
Theorem 10.12 Important
Angle in a semicircle is a right angle Important
Chapter 10 Class 9 Circles (Term 2)
Serial order wise
Transcript
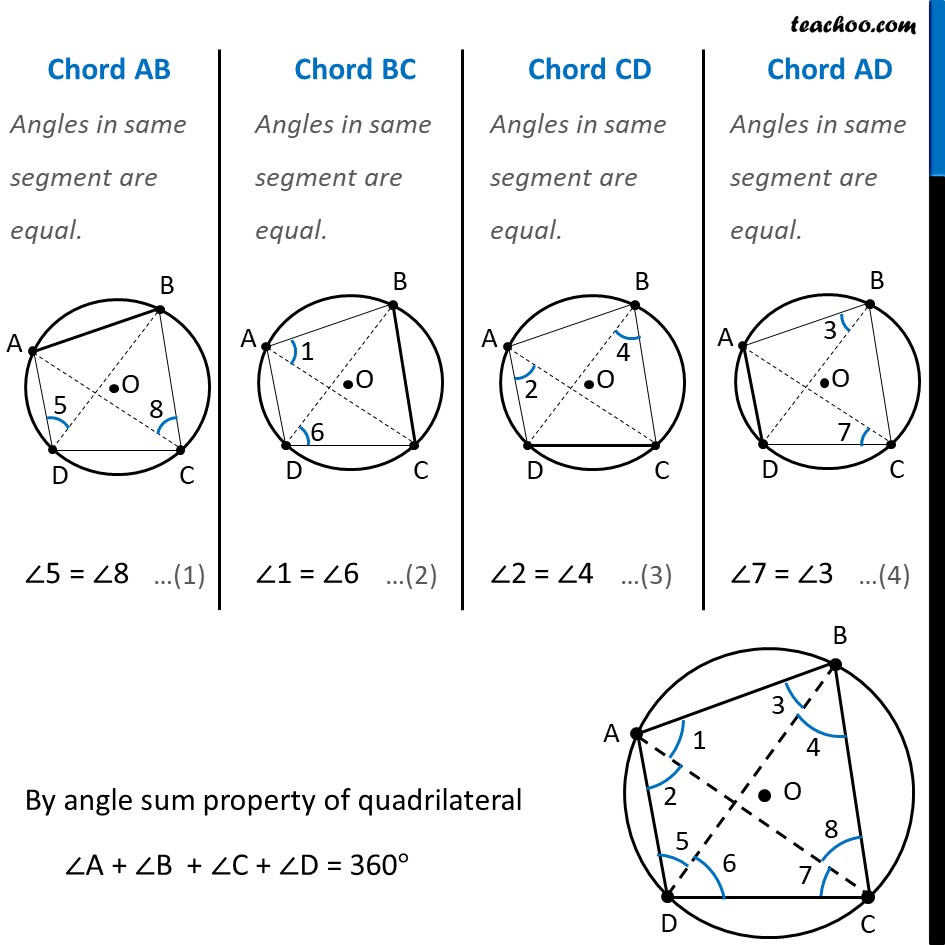
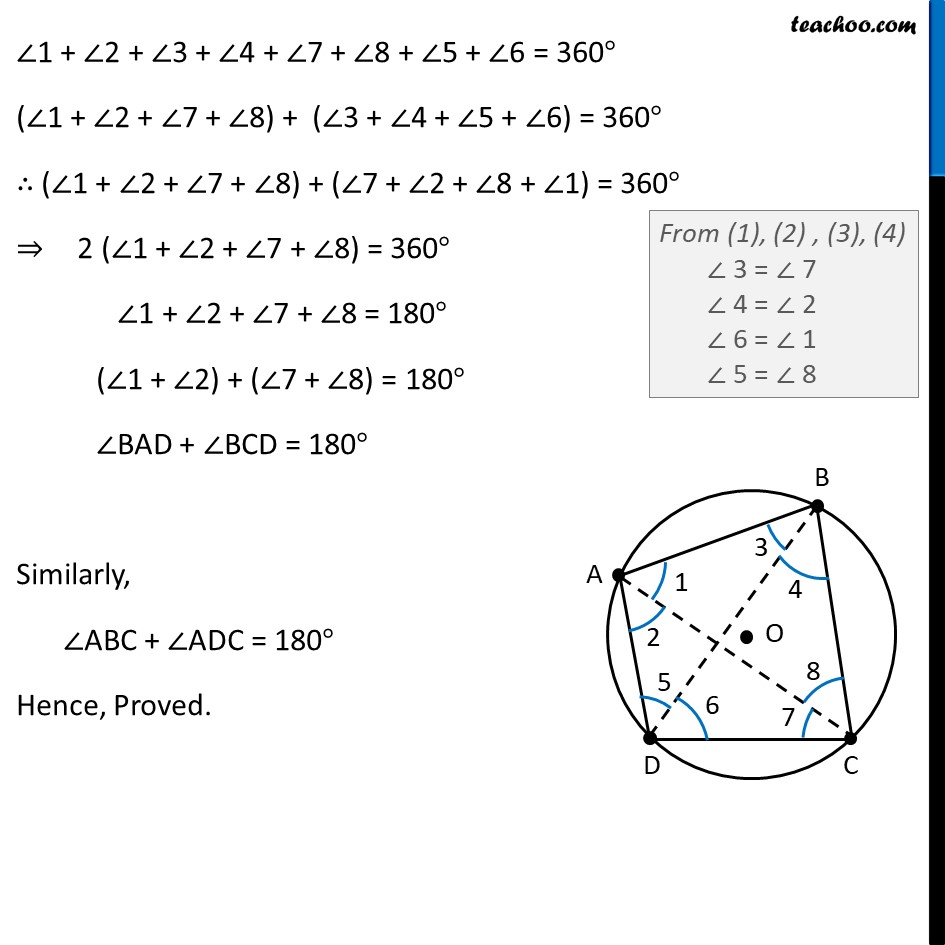
Theorem 10.11 The sum of either pair of opposite angles of a cyclic quadrilateral is 180°. Given : ABCD is a cyclic quadrilateral. of a circle with centre at O To Prove : ∠ BAD + ∠ BCD = 180° ∠ ABC + ∠ ADC = 180° Proof: Chord AB Angles in same segment are equal. ∠5 = ∠8 Chord BC Angles in same segment are equal. ∠1 = ∠6 Chord CD Angles in same segment are equal. ∠2 = ∠4 Chord AD Angles in same segment are equal. ∠7 = ∠3 ∠1 + ∠2 + ∠3 + ∠4 + ∠7 + ∠8 + ∠5 + ∠6 = 360° (∠1 + ∠2 + ∠7 + ∠8) + (∠3 + ∠4 + ∠5 + ∠6) = 360° ∴ (∠1 + ∠2 + ∠7 + ∠8) + (∠7 + ∠2 + ∠8 + ∠1) = 360° ⇒ 2 (∠1 + ∠2 + ∠7 + ∠8) = 360° ∠1 + ∠2 + ∠7 + ∠8 = 180° (∠1 + ∠2) + (∠7 + ∠8) = 180° ∠BAD + ∠BCD = 180° Similarly, ∠ABC + ∠ADC = 180° Hence, Proved. From (1), (2) , (3), (4) ∠ 3 = ∠ 7 ∠ 4 = ∠ 2 ∠ 6 = ∠ 1 ∠ 5 = ∠ 8
