Example 1 - Chapter 9 Class 9 Circles

Last updated at July 28, 2026 by

Example 1 - If two intersecting chords of a circle make - Examples - Examples

part 2 - Example 1 - Examples - Serial order wise - Chapter 9 Class 9 Circles

 

Remove Ads

Transcript

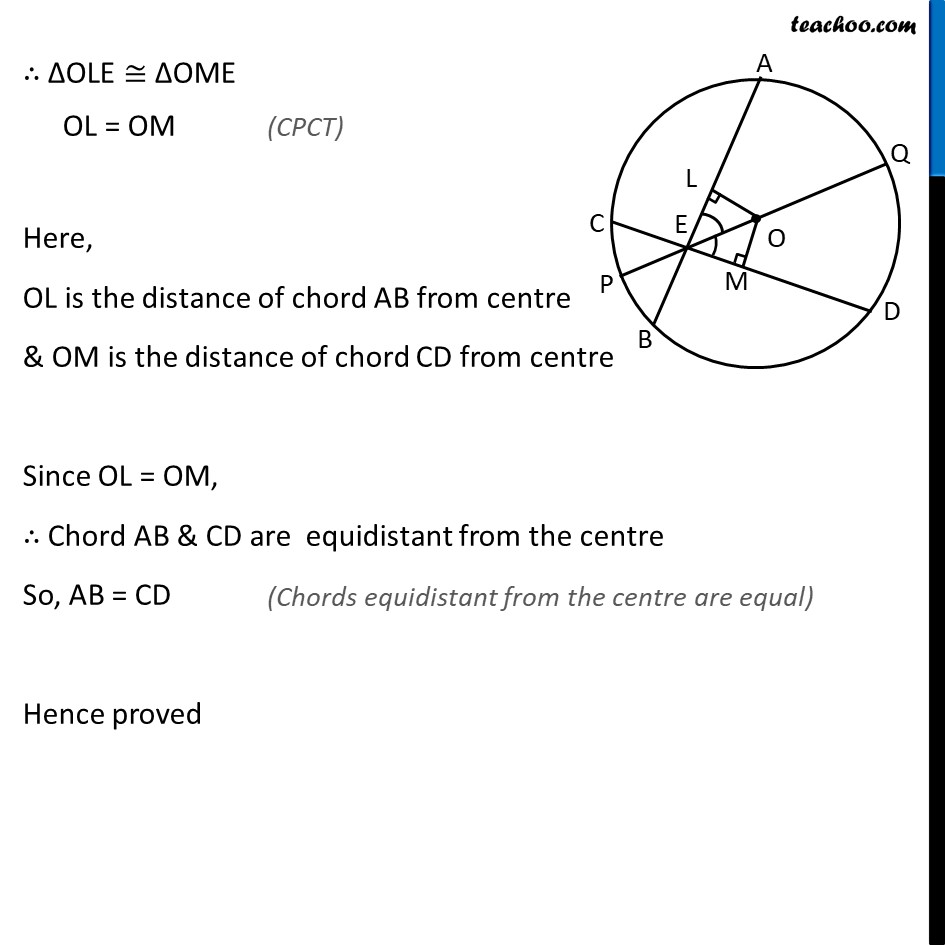
Example 1 If two intersecting chords of a circle make equal angles with the diameter passing through their point of intersection, prove that the chords are equal. Given: Let AB & CD be two chords intersecting at E. PQ is the diameter passing through E AB & CD makes equal angles with diameter PQ &., AEQ = DEQ To prove: Chords are equal i.e., AB = CD Construction: We draw OL AB & OM CD Proof: In OLE and OME, OLE = OME OEL = OEM OE = OE OLE OME OL = OM Here, OL is the distance of chord AB from centre & OM is the distance of chord CD from centre Since OL = OM, Chord AB & CD are equidistant from the centre So, AB = CD Hence proved

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh is an IIT Kanpur graduate and has been teaching for 16+ years. At Teachoo, he breaks down Maths, Science and Computer Science into simple steps so students understand concepts deeply and score with confidence.

Many students prefer Teachoo Black for a smooth, ad-free learning experience.