Example 35 - Chapter 5 Class 12 Continuity and Differentiability

Transcript

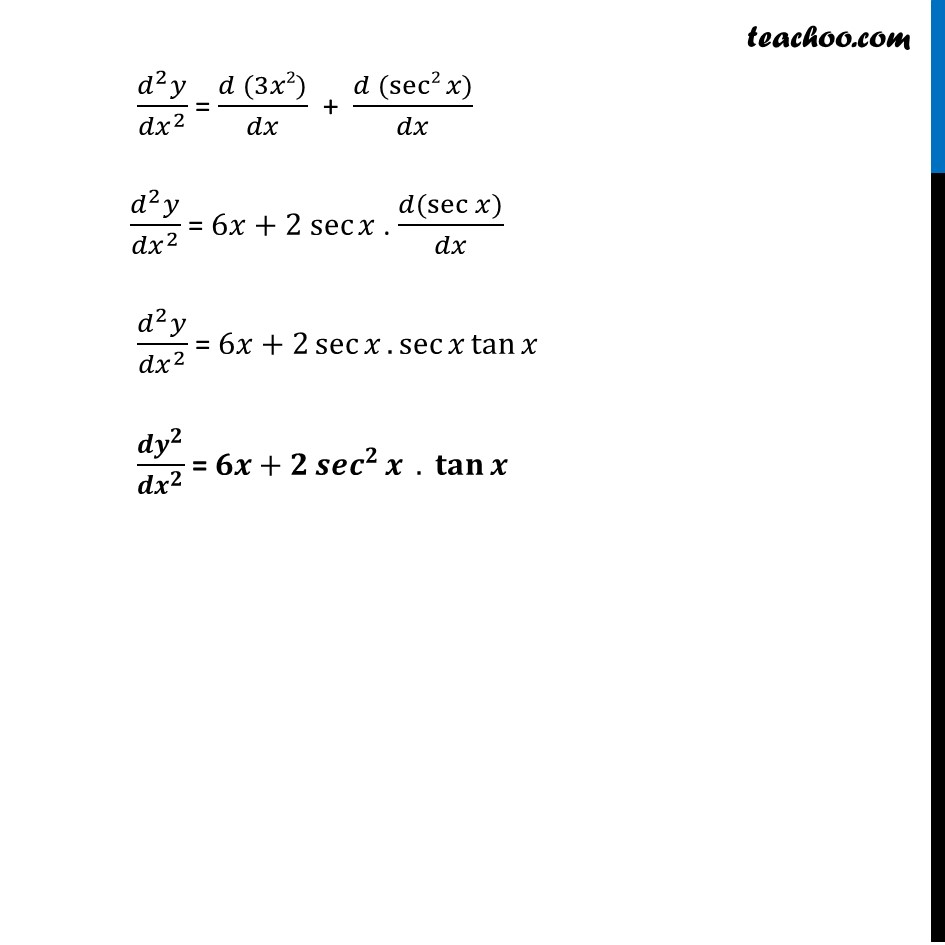
Example 35 Find 𝑑2𝑦/𝑑𝑥2 , if 𝑦 = 𝑥3+tan⁡𝑥. 𝑦 = 𝑥3+tan⁡𝑥 Differentiating 𝑤.𝑟.𝑡.𝑥 𝑑𝑦/𝑑𝑥 = (𝑑(𝑥^3+ tan⁡〖𝑥)〗)/𝑑𝑥 𝑑𝑦/𝑑𝑥 = (𝑑(𝑥^3))/𝑑𝑥 + (𝑑(tan⁡〖𝑥)〗)/𝑑𝑥 𝒅𝒚/𝒅𝒙 = 𝟑𝒙𝟐+𝒔𝒆𝒄𝟐 𝒙 Again Differentiating 𝑤.𝑟.𝑡.𝑥 (𝑑^2 𝑦)/〖𝑑𝑥〗^2 = (𝑑 (3𝑥2 +sec^2⁡𝑥))/𝑑𝑥 (𝑑^2 𝑦)/〖𝑑𝑥〗^2 = (𝑑 (3𝑥2))/𝑑𝑥 + (𝑑 (sec2 𝑥))/𝑑𝑥 (𝑑^2 𝑦)/〖𝑑𝑥〗^2 = 6𝑥+2 sec⁡𝑥 . (𝑑(sec⁡〖𝑥)〗)/𝑑𝑥 (𝑑^2 𝑦)/〖𝑑𝑥〗^2 = 6𝑥+2 sec⁡𝑥.sec⁡〖𝑥 tan⁡𝑥 〗 〖𝒅𝒚〗^𝟐/〖𝒅𝒙〗^𝟐 = 𝟔𝒙+𝟐 〖𝒔𝒆𝒄〗^𝟐⁡𝒙 . 𝐭𝐚𝐧⁡𝒙