Question 14 - NCERT Exemplar - MCQ - Chapter 7 Class 10 Coordinate Geometry

Last updated at Dec. 16, 2024 by

The fourth vertex D of a parallelogram ABCD whose three vertices are A (–2, 3), B (6, 7) and C (8, 3) is

(A) (0, 1)   (B) (0, –1)   (C) (–1, 0)   (D) (1, 0)

 

Slide27.JPG

Slide28.JPG

Go Ad-free

Transcript

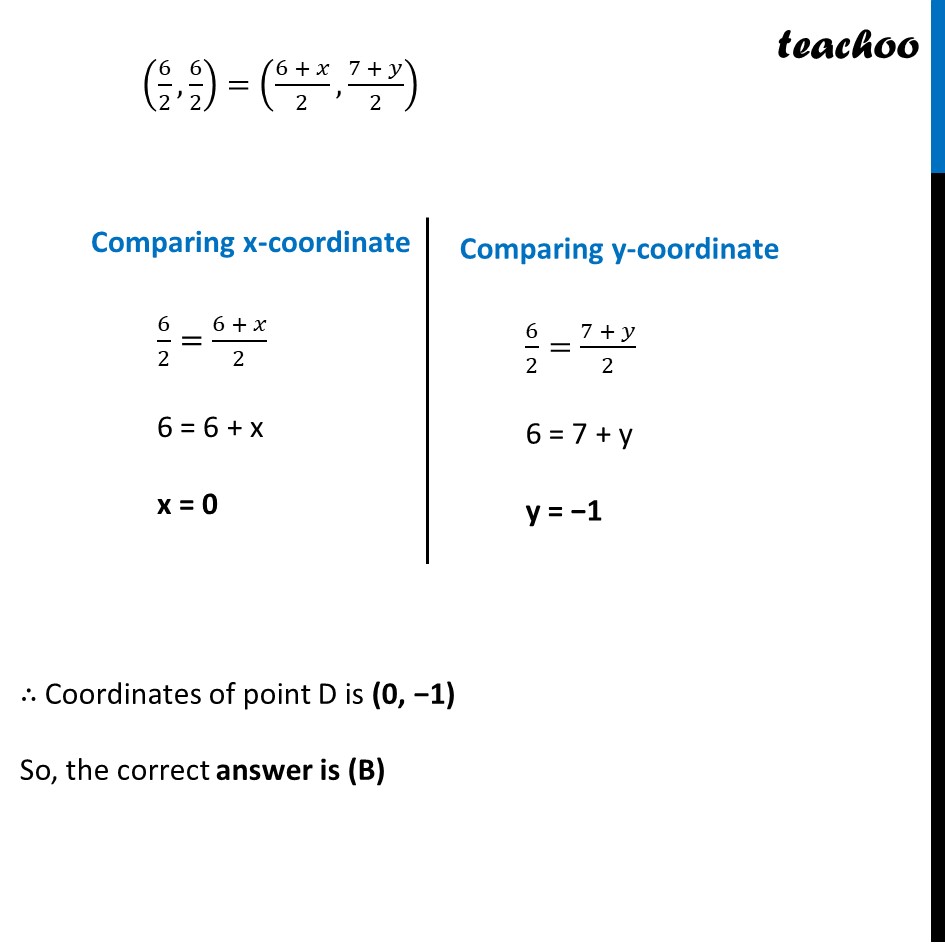
Question 14 The fourth vertex D of a parallelogram ABCD whose three vertices are A (–2, 3), B (6, 7) and C (8, 3) is (A) (0, 1) (B) (0, –1) (C) (–1, 0) (D) (1, 0) Since ABCD is a parallelogram It’s Diagonals bisect each other Therefore, Mid point of AC = Mid point of BD ((−2 + 8)/2,(3 + 3)/2)=((6 + 𝑥)/2,(7 + 𝑦)/2) (6/2,6/2)=((6 + 𝑥)/2,(7 + 𝑦)/2) Comparing x-coordinate 6/2=(6 + 𝑥)/2 6 = 6 + x x = 0 Comparing y-coordinate 6/2=(7 + 𝑦)/2 6 = 7 + y y = −1 ∴ Coordinates of point D is (0, −1) So, the correct answer is (B)

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo