Binary Operations

Relations - Definition →
Chapter 1 Class 12 Relation and Functions
Serial order wise

Ex 1.4, 2 (vi) - Chapter 1 Class 12 Relation and Functions

Last updated at April 16, 2024 by

Ex 1.4, 2 - Chapter 1 Class 12 Relation and Functions - Part 12

Ex 1.4, 2 - Chapter 1 Class 12 Relation and Functions - Part 13

Transcript

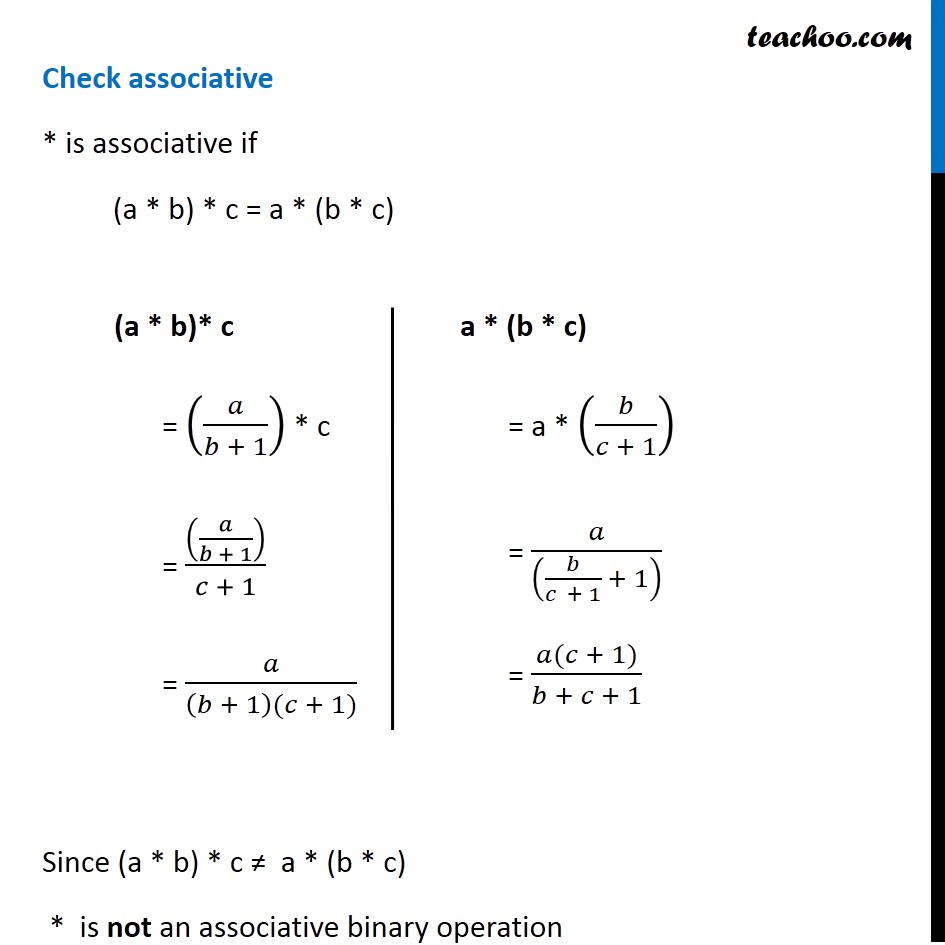
Ex 1.4, 2 For each binary operation * defined below, determine whether * is commutative or associative. (vi) On R − {−1}, define a * b = a/(b + 1) Check commutative * is commutative if a * b = b * a Since a * b ≠ b * a * is not commutative a * b = 𝑎/(𝑏 + 1) b * a = 𝑏/(𝑎 + 1) Check associative * is associative if (a * b) * c = a * (b * c) Since (a * b) * c ≠ a * (b * c) * is not an associative binary operation (a * b)* c = (𝑎/(𝑏 + 1)) * c = ((𝑎/(𝑏 + 1)))/(𝑐 + 1) = 𝑎/((𝑏 + 1)(𝑐 + 1)) a * (b * c) = a * (𝑏/(𝑐 + 1)) = 𝑎/((𝑏/(𝑐 + 1) + 1) ) = (𝑎(𝑐 + 1))/(𝑏 + 𝑐 + 1)

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.