Question 6 - Solving easy equations - Word Problems - Chapter 2 Class 8 Linear Equations in One Variable

Last updated at April 16, 2024 by

Ex 2.2, 6 - Three consecutive integers add up to 51. What are

Ex 2.2, 6 - Chapter 2 Class 8 Linear Equations in One Variable - Part 2

Transcript

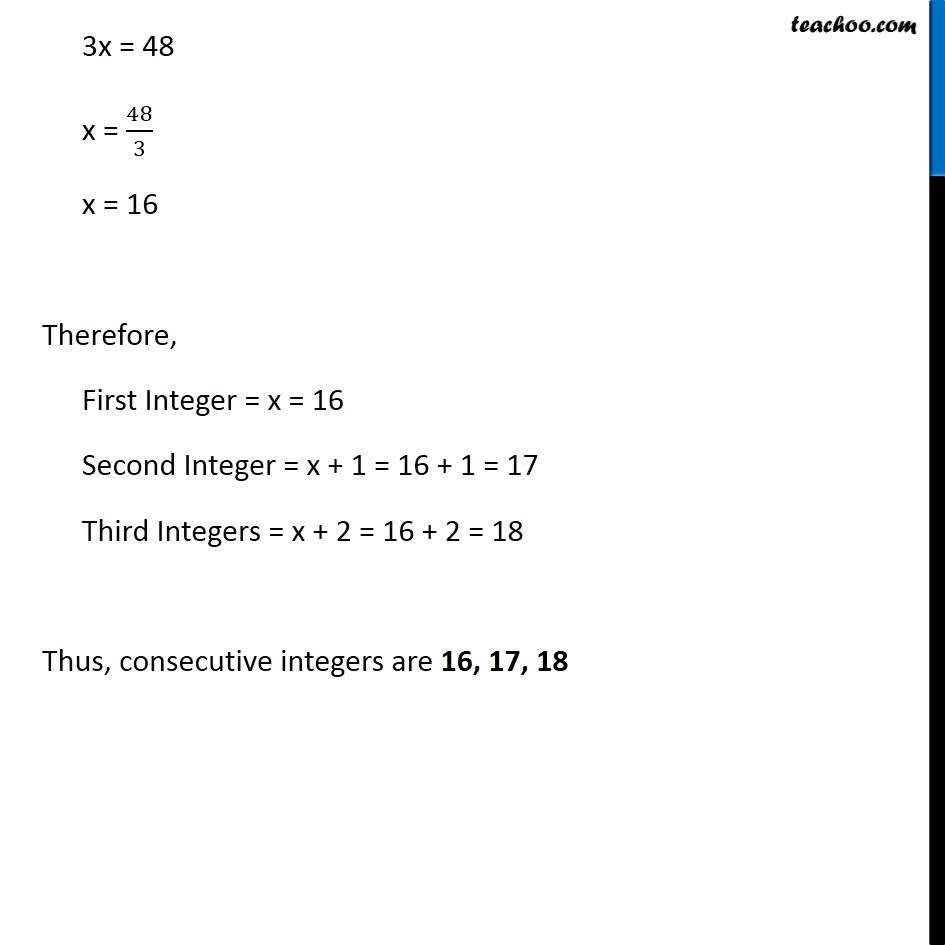
Question 6 Three consecutive integers add up to 51. What are these integers?Let first Integer = x Second Integer = x + 1 Third Integer = (x + 1) + 1 = x + 2 Now, Sum of Integers = 51 x + (x + 1) + (x + 2) = 51 x + x + x + 1 + 2 = 51 3x + 3 = 51 3x = 51 − 3 Rough Integers are −2, −1, 0, 1, 2, … Consecutive integers are 1, 2, 3 Difference between Consecutive Integers = 1 If number starting from 1, then First number = 1 Second number = 1 + 1 = 2 Third number = 2 + 1 = 3 3x = 48 x = 48/3 x = 16 Therefore, First Integer = x = 16 Second Integer = x + 1 = 16 + 1 = 17 Third Integers = x + 2 = 16 + 2 = 18 Thus, consecutive integers are 16, 17, 18

Ask a doubt
Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science, Social Science, Physics, Chemistry, Computer Science at Teachoo.