Ex 3.2, 1 - Chapter 3 Class 12 Matrices

Transcript

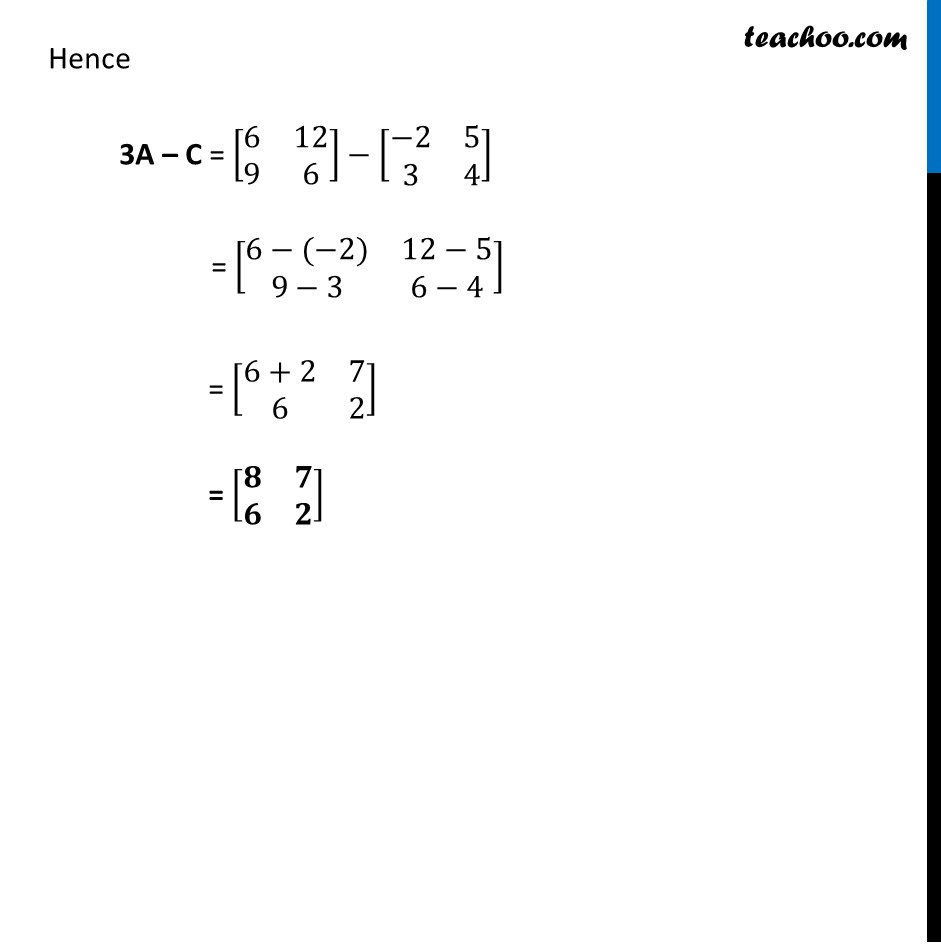
Ex 3.2, 1 Let A = [■8(2&4@3&2)], B = [■8(1&3@−2&5)], C = [■8(−2&5@3&4)] Find each of the following (i) A + B A + B = [■8(2&4@3&2)] + [■8( 1&3@−2&5)] = [■8(2+1&4+3@3−2&2+5)] = [■8(𝟑&𝟕@𝟏&𝟕)] Ex 3.2,1 Let A = [■8(2&4@3&2)], B = [■8(1&3@−2&5)], C = [■8(−2&5@3&4)] Find each of the following (ii) A – B A – B = [■8(2&4@3&2)]− [■8( 1&3@−2&5)] = [■8(2−1&4−3@3−(−2)&2−5)] = [■8(1&1@3+2&−3)] = [■8(𝟏&𝟏@𝟓&−𝟑)] Ex 3.2, 1 Let A = [■8(2&4@3&2)], B = [■8(1&3@−2&5)], C = [■8(−2&5@3&4)] Find each of the following 3A – C Finding 3A 3A = 3[■8(2&4@3&2)] = [■8(3 × 2&3 × 4@3 × 3 &3 × 2)] = [■8(𝟔&𝟏𝟐@𝟗&𝟔)] Hence 3A – C = [■8(6&12@9&6)] ⤶7− [■8(−2&5@3&4)] = [■8(6−(−2)&12−5@9−3&6−4)] = [■8(6+2&7@6&2)] = [■8(𝟖&𝟕@𝟔&𝟐)] Ex 3.2, 1 Let A = [■8(2&4@3&2)] B = [■8(1&3@−2&5)] , C = [■8(−2&5@3&4)]. Find each of the following (iv)AB AB = [■8(2&4@3&2)] [■8(1&3@−2&5)] = [■8(2 × 1+4 × −2 &2 × 3+4 × 5@3 × 1+2 × −2&3 × 3+2 × 5)] = [■8(2−8&6+20@3−4&9+10)] = [■8(−𝟔&𝟐𝟔@−𝟏&𝟏𝟗)] Ex 3.2, 1 Let A = [■8(2&4@3&2)] B = [■8(1&3@−2&5)] , C = [■8(−2&5@3&4)]. Find each of the following (v) BA BA = [■8(1&3@−2&5)] [■8(2&4@3&2)] = [■8(1 × 2+3 × 3 &1 × 4+3 × 2@−2 × 2+5 × 3&−2 × 4+5 × 2)] = [■8(2+9&4+6@−4+15&−8+10)] = [■8(𝟏𝟏&𝟏𝟎@𝟏𝟏&𝟐)]