Example 13 - Chapter 9 Class 12 Differential Equations (Important Question)

Transcript

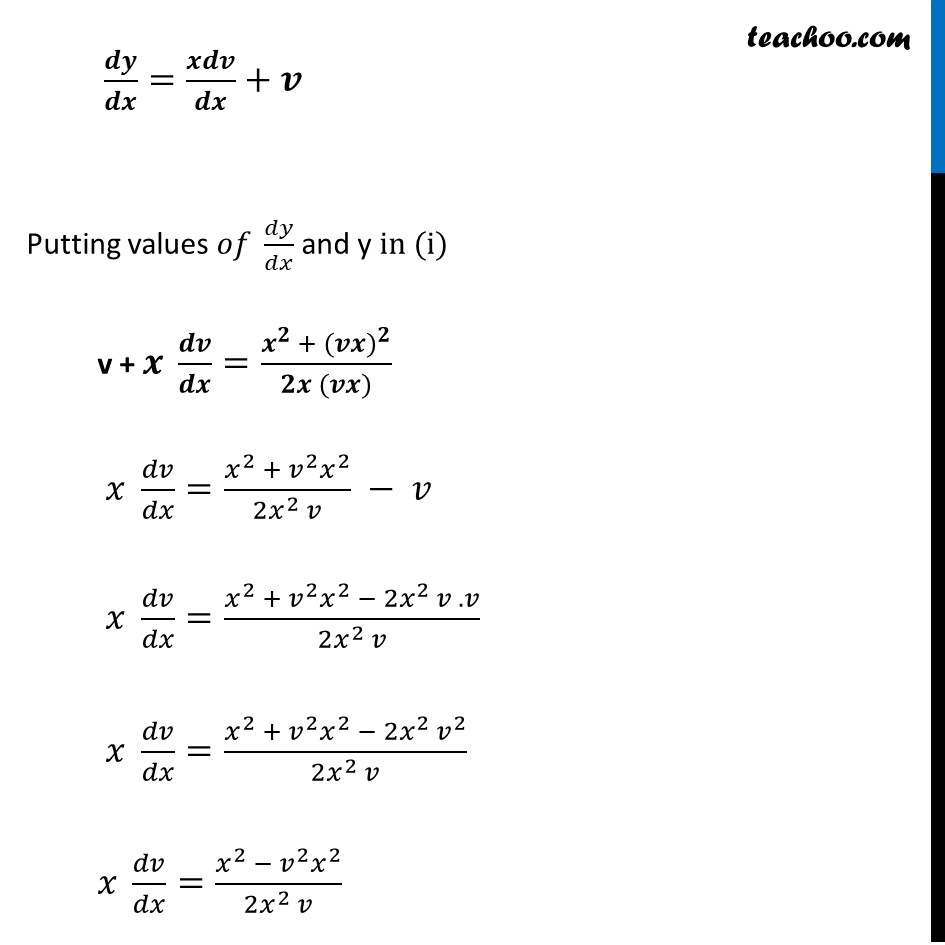
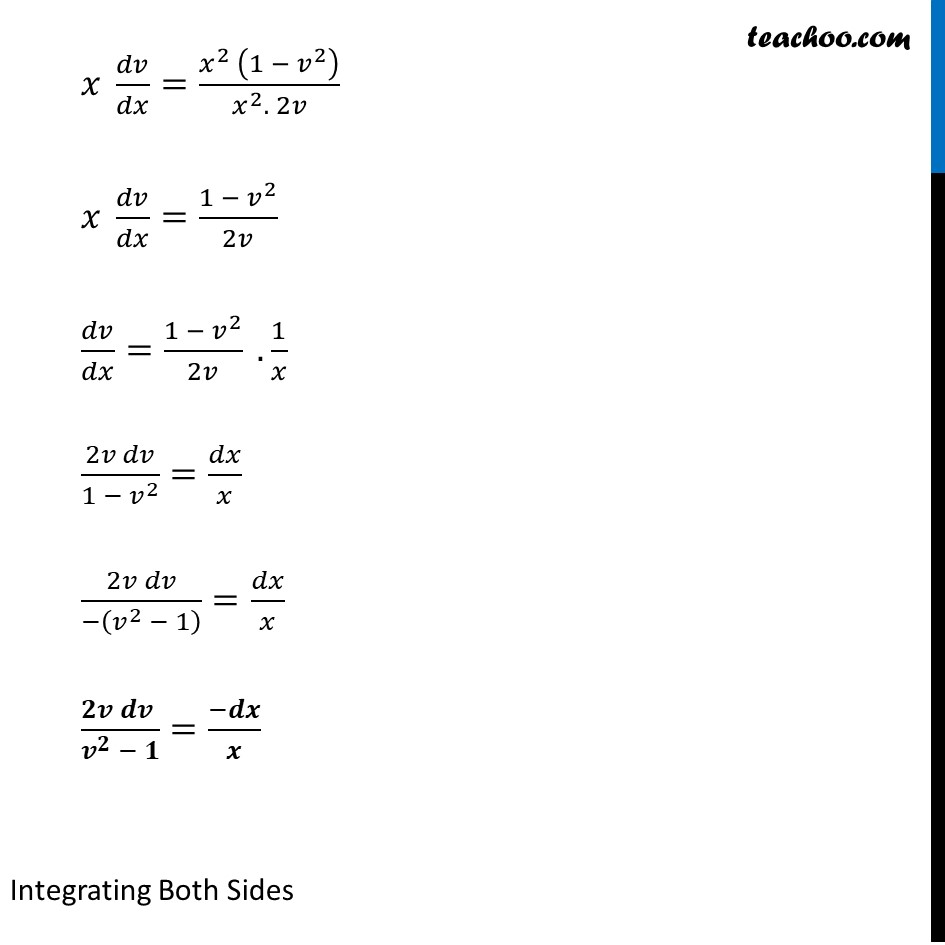
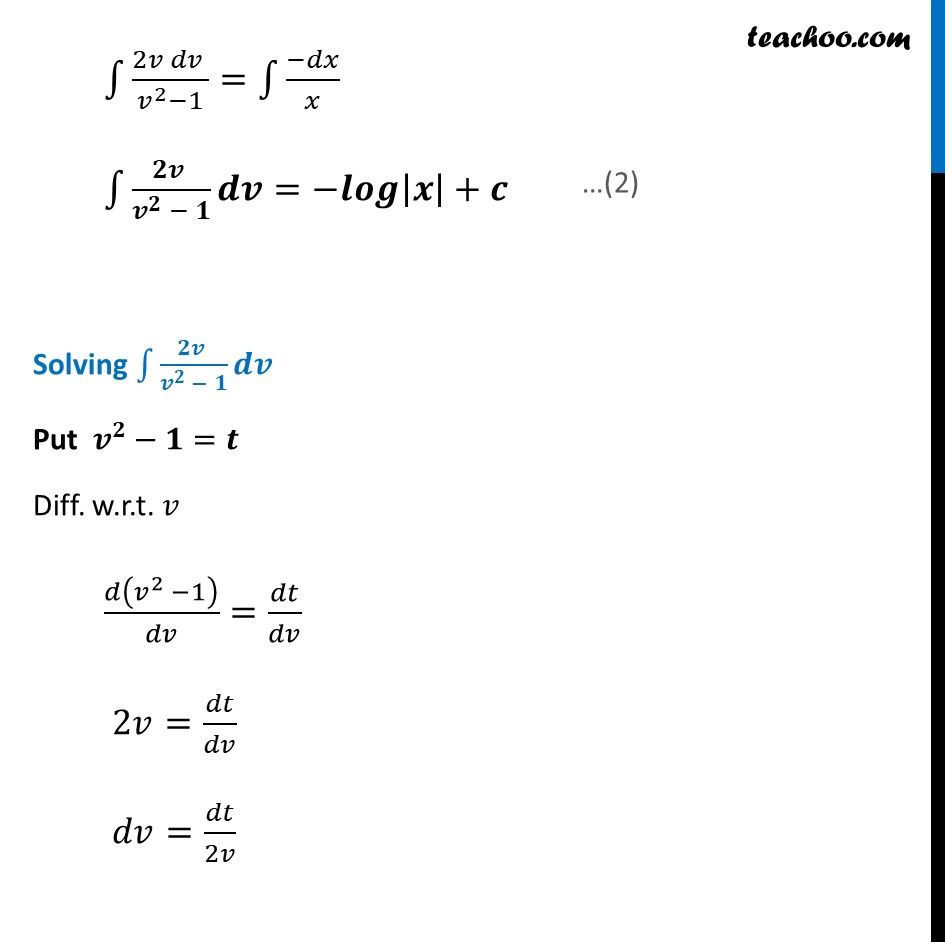
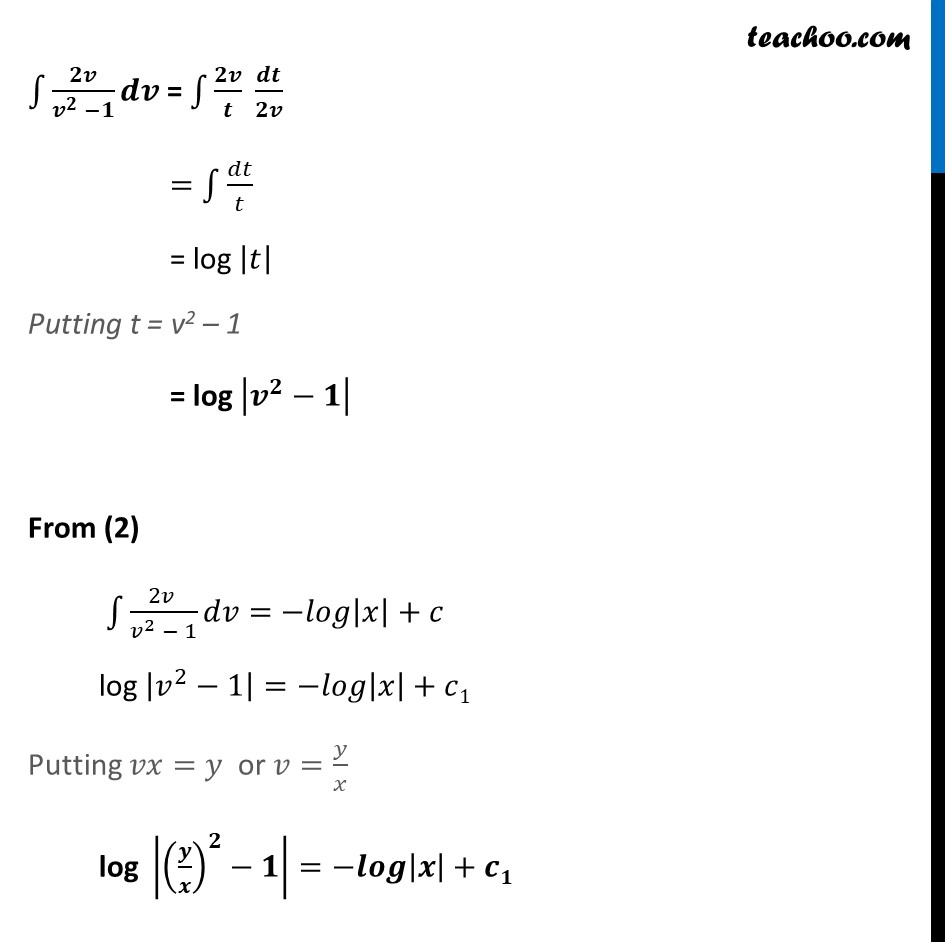
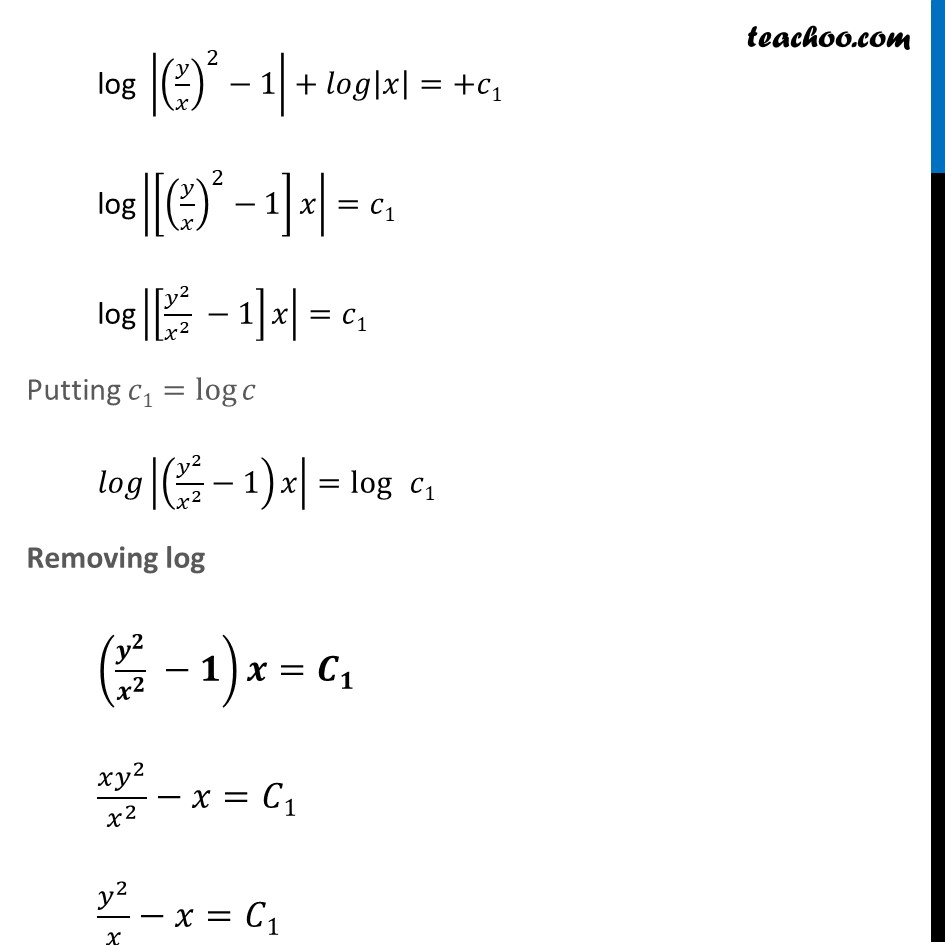
Example 13 Show that the family of curves for which the slope of the tangent at any point (𝑥 , 𝑦) on its (𝑥^2+𝑦^2)/2𝑥𝑦 , is given by 𝑥^2−𝑦^2=𝑐𝑥 We know that the slope of the tangent at (𝑥 ,𝑦) of a curve is 𝑑𝑦/𝑑𝑥 Given slope of tangent at (𝑥 , 𝑦) is (𝑥^2 +〖 𝑦〗^2)/2𝑥𝑦 Therefore 𝒅𝒚/𝒅𝒙=(𝒙^𝟐 +〖 𝒚〗^𝟐)/𝟐𝒙𝒚 Step 1: Find 𝑑𝑦/𝑑𝑥 𝑑𝑦/𝑑𝑥=(𝑥^2 +𝑦^2)/2𝑥𝑦 Step 2: Put 𝑑𝑦/𝑑𝑥= F(𝑥 𝑦) So, F(𝑥 𝑦)=(𝑥^2 + 𝑦^2)/2𝑥𝑦 Finding F(𝜆𝑥 ,𝜆𝑦) F(𝜆𝑥 ,𝜆𝑦)=((𝜆𝑥)^2 + (𝜆𝑦)^2)/2(𝜆𝑥)(𝜆𝑦) =(𝜆^2 𝑥^2 + 𝜆^2 𝑦^2)/(2𝜆^2 𝑥𝑦) =(𝜆^2 (𝑥^2 +〖 𝑦〗^2 ))/(𝜆^2 2𝑥𝑦) = ((𝑥^2 +〖 𝑦〗^2 ))/( 2𝑥𝑦) = F(𝑥 , 𝑦) So , F(𝝀𝒙 ,𝝀𝒚)= F(𝑥 , 𝑦) =𝝀° "F" (𝒙 , 𝒚) So , "F" (𝑥 , 𝑦) is homogeneous function of degree zero, Therefore given equation is a homogeneous differential equation Step 3: Solving 𝑑𝑦/𝑑𝑥 by putting 𝑦=𝑣𝑥 𝑑𝑦/𝑑𝑥=(𝑥^2 + 𝑦^2)/2𝑥𝑦 Put 𝒚=𝒗𝒙 Diff. w.r.t. 𝑥 𝑑𝑦/𝑑𝑥=𝑑/𝑑𝑥 (𝑣𝑥) 𝑑𝑦/𝑑𝑥=𝑥 𝑑𝑣/𝑑𝑥+𝑣 𝑑𝑥/𝑑𝑥 𝒅𝒚/𝒅𝒙=𝒙𝒅𝒗/𝒅𝒙+𝒗 Putting values 𝑜𝑓 𝑑𝑦/𝑑𝑥 and y in (i") " v + 𝒙 𝒅𝒗/𝒅𝒙=(𝒙^𝟐 + (𝒗〖𝒙)〗^𝟐)/(𝟐𝒙 (𝒗𝒙)) " " 𝑥 𝑑𝑣/𝑑𝑥=(𝑥^2 + 𝑣^2 𝑥^2)/(2𝑥^2 𝑣) − 𝑣" " 𝑥 𝑑𝑣/𝑑𝑥=(𝑥^2 + 𝑣^2 𝑥^2 − 2𝑥^2 𝑣 . 𝑣)/(2𝑥^2 𝑣) 𝑥 𝑑𝑣/𝑑𝑥=(𝑥^2 + 𝑣^2 𝑥^2 − 2𝑥^2 𝑣^2)/(2𝑥^2 𝑣) 𝑥 𝑑𝑣/𝑑𝑥=(𝑥^2 − 𝑣^2 𝑥^2)/(2𝑥^2 𝑣) 𝑥 𝑑𝑣/𝑑𝑥=(𝑥^2 (1 − 𝑣^2 ))/(𝑥^2. 2𝑣) 𝑥 𝑑𝑣/𝑑𝑥=(1 − 𝑣^2)/2𝑣 𝑑𝑣/𝑑𝑥=(1 − 𝑣^2)/2𝑣 . 1/𝑥 (2𝑣 𝑑𝑣)/(1 − 𝑣^2 )=𝑑𝑥/𝑥 (2𝑣 𝑑𝑣)/(−(𝑣^2 − 1) )=𝑑𝑥/𝑥 (𝟐𝒗 𝒅𝒗 )/(𝒗^𝟐 − 𝟏)=(−𝒅𝒙)/𝒙 Integrating Both Sides ∫1▒〖(2𝑣 𝑑𝑣 )/(𝑣^2−1)=∫1▒(−𝑑𝑥)/𝑥〗 ∫1▒(𝟐𝒗 )/(𝒗^𝟐 − 𝟏) 𝒅𝒗=−𝒍𝒐𝒈|𝒙|+𝒄 Solving ∫1▒(𝟐𝒗 )/(𝒗^𝟐 − 𝟏) 𝒅𝒗 Put 𝒗^𝟐−𝟏=𝒕 Diff. w.r.t. 𝑣 𝑑(𝑣^2 −1)/𝑑𝑣=𝑑𝑡/𝑑𝑣 2𝑣=𝑑𝑡/𝑑𝑣 " " 𝑑𝑣=𝑑𝑡/2𝑣 " " ∫1▒𝟐𝒗/(𝒗^𝟐 −𝟏) 𝒅𝒗 = ∫1▒〖𝟐𝒗/𝒕 〗 𝒅𝒕/𝟐𝒗 =∫1▒𝑑𝑡/𝑡 = log |𝑡| Putting t = v2 – 1 = log |𝒗^𝟐−𝟏| From (2) ∫1▒(2𝑣 )/(𝑣^2 − 1) 𝑑𝑣=−𝑙𝑜𝑔|𝑥|+𝑐 "log " |𝑣^2−1|=−𝑙𝑜𝑔|𝑥|+𝑐1 Putting 𝑣𝑥=𝑦 or 𝑣=𝑦/𝑥 "log " |(𝒚/𝒙)^𝟐−𝟏|=−𝒍𝒐𝒈|𝒙|+𝒄𝟏 "log " |(𝑦/𝑥)^2−1|+𝑙𝑜𝑔|𝑥|=+𝑐1 "log" |[(𝑦/𝑥)^2−1]𝑥|=𝑐1 "log" |[𝑦^2/𝑥^2 −1]𝑥|=𝑐1 Putting 𝑐1=log⁡𝑐 𝑙𝑜𝑔|(𝑦^2/𝑥^2 −1)𝑥|=log⁡ 𝑐1 Removing log (𝒚^𝟐/𝒙^𝟐 −𝟏)𝒙=𝑪𝟏 (𝑥𝑦^2)/𝑥^2 −𝑥=𝐶1 𝑦^2/𝑥−𝑥=𝐶1 (𝑦^2−𝑥^2)/𝑥=𝐶1" " 𝑦^2−𝑥^2=𝐶1𝑥 𝑥^2−𝑦^2=−𝐶1𝑥 Put 𝑐=−𝐶1 𝒙^𝟐−𝒚^𝟐=𝒄𝒙 Hence Proved