You are here
Question 6 - Solving by simplifying det. - Chapter 4 Class 12 Determinants
Last updated at Dec. 16, 2024 by Teachoo
Chapter 4 Class 12 Determinants
Concept wise
- Finding determinant of a 2x2 matrix
- Evalute determinant of a 3x3 matrix
- Area of triangle
- Equation of line using determinant
- Finding Minors and cofactors
- Evaluating determinant using minor and co-factor
- Find adjoint of a matrix
- Finding Inverse of a matrix
- Inverse of two matrices and verifying properties
- Finding inverse when Equation of matrice given
- Checking consistency of equations
- Find solution of equations- Equations given
- Find solution of equations- Statement given
- Verifying properties of a determinant
- Two rows or columns same
- Whole row/column zero
- Whole row/column one
- Making whole row/column one and simplifying
- Proving Determinant 1 = Determinant 2
-
Solving by simplifying det.
- Using Property 5 (Determinant as sum of two or more determinants)
Transcript
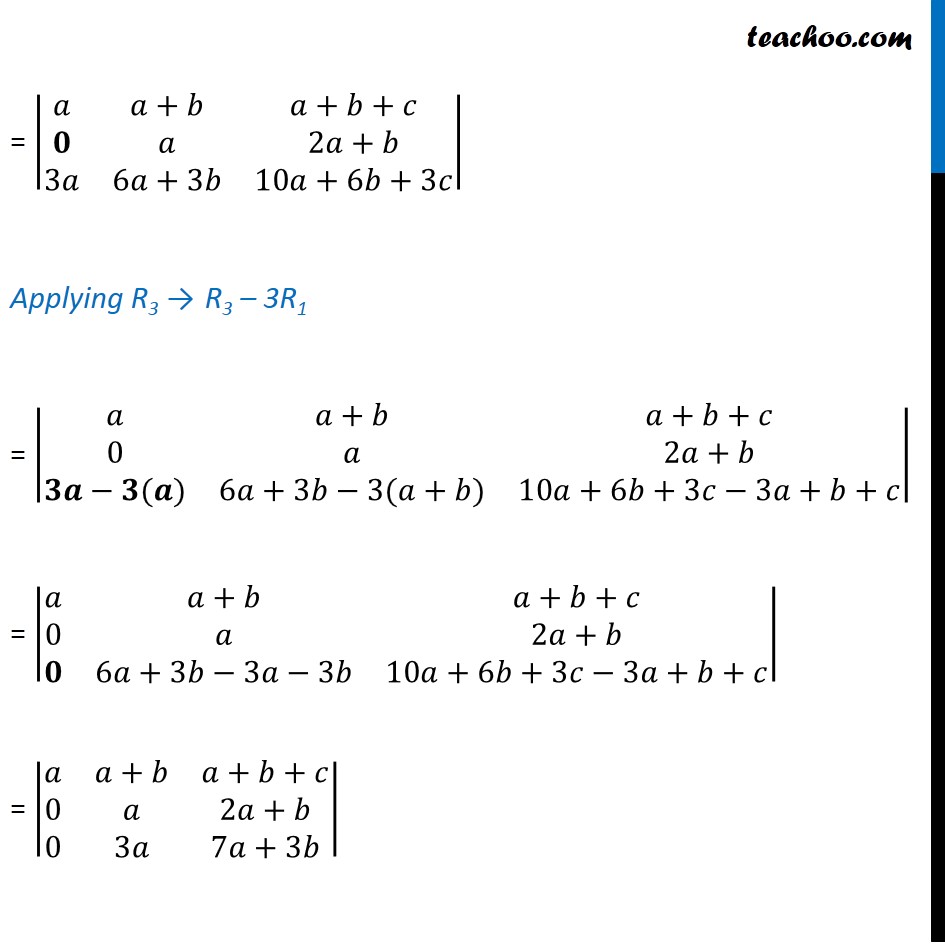
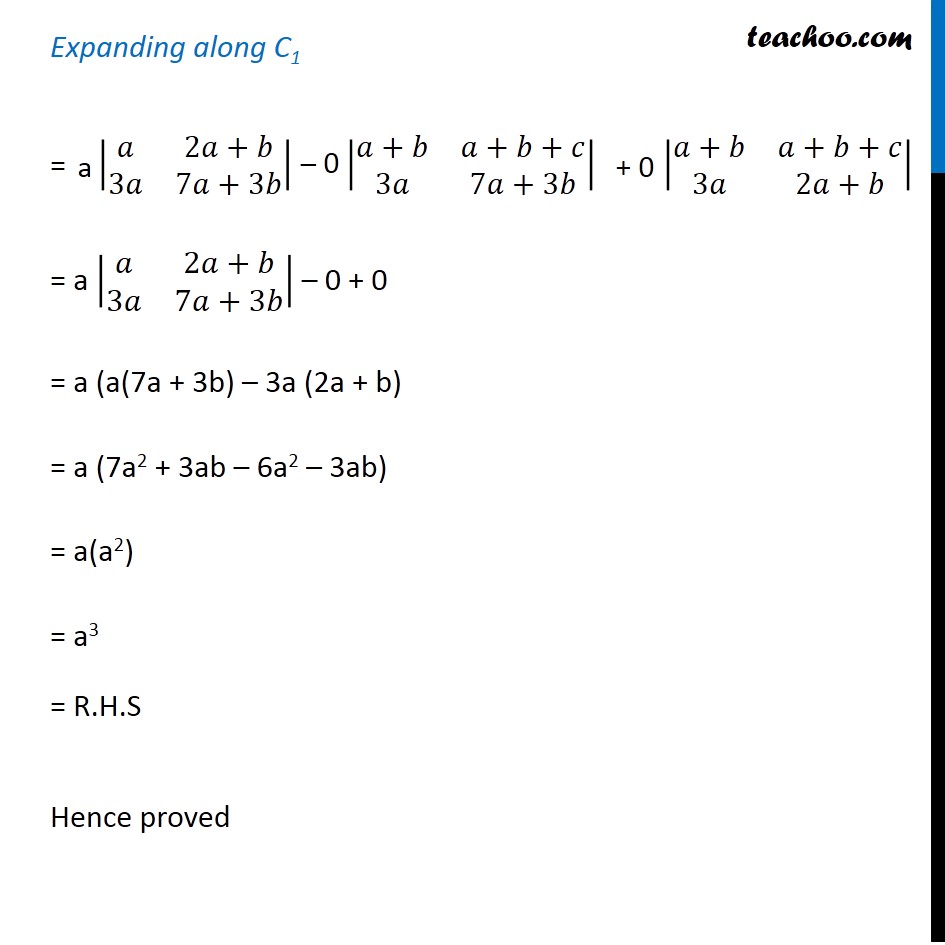
Question 6 Prove that |■8(𝑎&𝑎+𝑏&𝑎+𝑏+𝑐@2𝑎&3𝑎+2𝑏&4𝑎+3𝑏+2𝑐@3𝑎&6𝑎+3𝑏&10𝑎+6𝑏+3𝑐)| = a3 Let Δ = |■8(𝑎&𝑎+𝑏&𝑎+𝑏+𝑐@2𝑎&3𝑎+2𝑏&4𝑎+3𝑏+2𝑐@3𝑎&6𝑎+3𝑏&10𝑎+6𝑏+3𝑐)| Applying R2 → R2 – 2R1 = |■8(𝑎&𝑎+𝑏&𝑎+𝑏+𝑐@𝟐𝒂−𝟐(𝒂)&3𝑎+3𝑏−2(𝑎+𝑏)&4𝑎+3𝑏+2𝑐−2(𝑎+𝑏+𝑐)@3𝑎&6𝑎+3𝑏&10𝑎+6𝑏+3𝑐)| = |■8(𝑎&𝑎+𝑏&𝑎+𝑏+𝑐@𝟎&3𝑎+3𝑏−2𝑎−2𝑏&4𝑎+3𝑏+2𝑐−2𝑎−2𝑏−2𝑐@3𝑎&6𝑎+3𝑏&10𝑎+6𝑏+3𝑐)| = |■8(𝑎&𝑎+𝑏&𝑎+𝑏+𝑐@𝟎&𝑎&2𝑎+𝑏@3𝑎&6𝑎+3𝑏&10𝑎+6𝑏+3𝑐)| Applying R3 → R3 – 3R1 = |■8(𝑎&𝑎+𝑏&𝑎+𝑏+𝑐@0&𝑎&2𝑎+𝑏@𝟑𝒂−𝟑(𝒂)&6𝑎+3𝑏−3(𝑎+𝑏)&10𝑎+6𝑏+3𝑐−3𝑎+𝑏+𝑐)| = |■8(𝑎&𝑎+𝑏&𝑎+𝑏+𝑐@0&𝑎&2𝑎+𝑏@𝟎&6𝑎+3𝑏−3𝑎−3𝑏&10𝑎+6𝑏+3𝑐−3𝑎+𝑏+𝑐)| = |■8(𝑎&𝑎+𝑏&𝑎+𝑏+𝑐@0&𝑎&2𝑎+𝑏@0&3𝑎&7𝑎+3𝑏)| Expanding along C1 = = a |■8(𝑎&2𝑎+𝑏@3𝑎&7𝑎+3𝑏)| – 0 + 0 = a (a(7a + 3b) – 3a (2a + b) = a (7a2 + 3ab – 6a2 – 3ab) = a(a2) = a3 = R.H.S Hence proved |■8(𝑎&2𝑎+𝑏@3𝑎&7𝑎+3𝑏)| |■8(𝑎+𝑏&𝑎+𝑏+𝑐@3𝑎&7𝑎+3𝑏)|
