You are here
Ex 4.1, 4 - Chapter 4 Class 12 Determinants
Last updated at Dec. 16, 2024 by Teachoo
Evalute determinant of a 3x3 matrix
Chapter 4 Class 12 Determinants
Concept wise
- Finding determinant of a 2x2 matrix
-
Evalute determinant of a 3x3 matrix
- Area of triangle
- Equation of line using determinant
- Finding Minors and cofactors
- Evaluating determinant using minor and co-factor
- Find adjoint of a matrix
- Finding Inverse of a matrix
- Inverse of two matrices and verifying properties
- Finding inverse when Equation of matrice given
- Checking consistency of equations
- Find solution of equations- Equations given
- Find solution of equations- Statement given
- Verifying properties of a determinant
- Two rows or columns same
- Whole row/column zero
- Whole row/column one
- Making whole row/column one and simplifying
- Proving Determinant 1 = Determinant 2
- Solving by simplifying det.
- Using Property 5 (Determinant as sum of two or more determinants)
Transcript
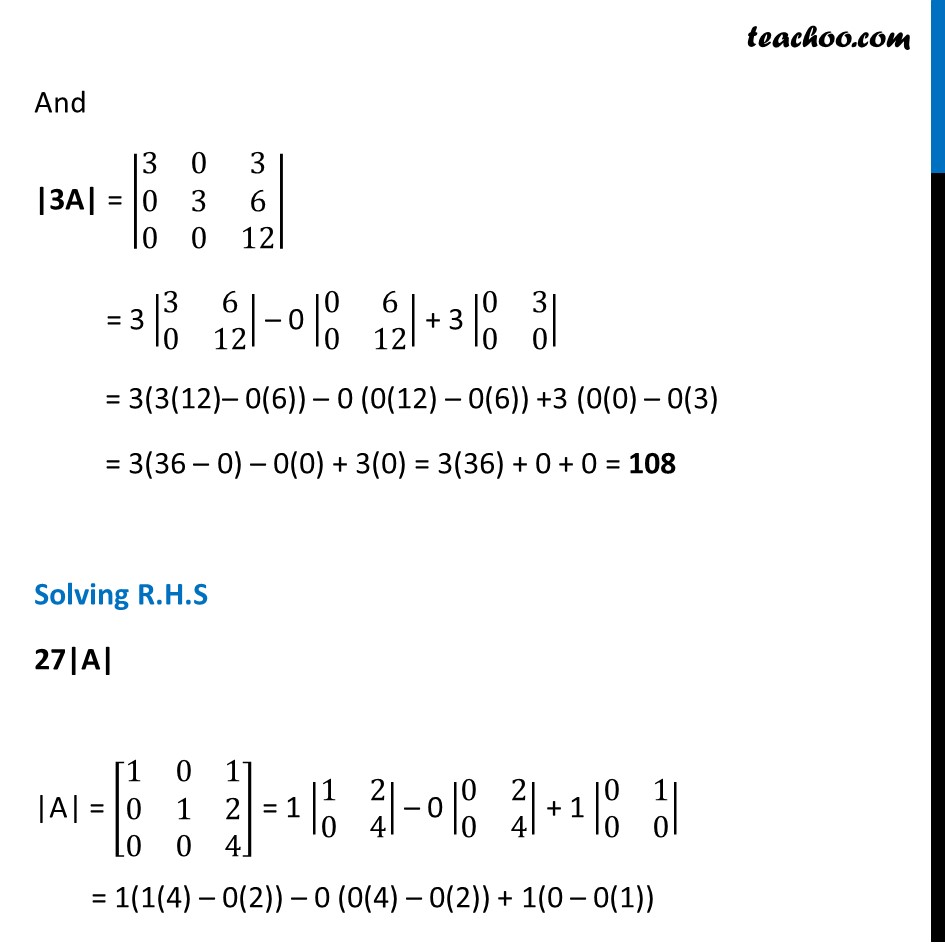
Ex 4.1, 4 If A = [■8(1&0&1@0&1&2@0&0&4)] , then show that |3A| = 27 |A| We have to prove |3A| = 27 |A| Solving L.H.S |3A| First Calculating 3A 3A = 3 [■8(1&0&1@0&1&2@0&0&4)] = [■8(3 × 1&3 × 0&3 × 1@3 × 0&3 × 1&3 × 2@3 × 0&3 × 0&3 × 4)] = [■8(3&0&3@0&3&6@0&0&12)] And |3A| = |■8(3&0&3@0&3&6@0&0&12)| = 3 |■8(3&6@0&12)| – 0 |■8(0&6@0&12)| + 3 |■8(0&3@0&0)| = 3(3(12)– 0(6)) – 0 (0(12) – 0(6)) +3 (0(0) – 0(3) = 3(36 – 0) – 0(0) + 3(0) = 3(36) + 0 + 0 = 108 Solving R.H.S 27|A| |A| = [■8(1&0&1@0&1&2@0&0&4)] = 1 |■8(1&2@0&4)| – 0 |■8(0&2@0&4)| + 1 |■8(0&1@0&0)| = 1(1(4) – 0(2)) – 0 (0(4) – 0(2)) + 1(0 – 0(1)) = 1 (4 – 0) – 0 (0) + 1(0) = 4 Now, 27|A| = 27 (4) = 108 = |3A| Hence L.H.S = R.H.S Hence proved
