Question 2 - Miscellaneous - Chapter 3 Class 12 Matrices

Transcript

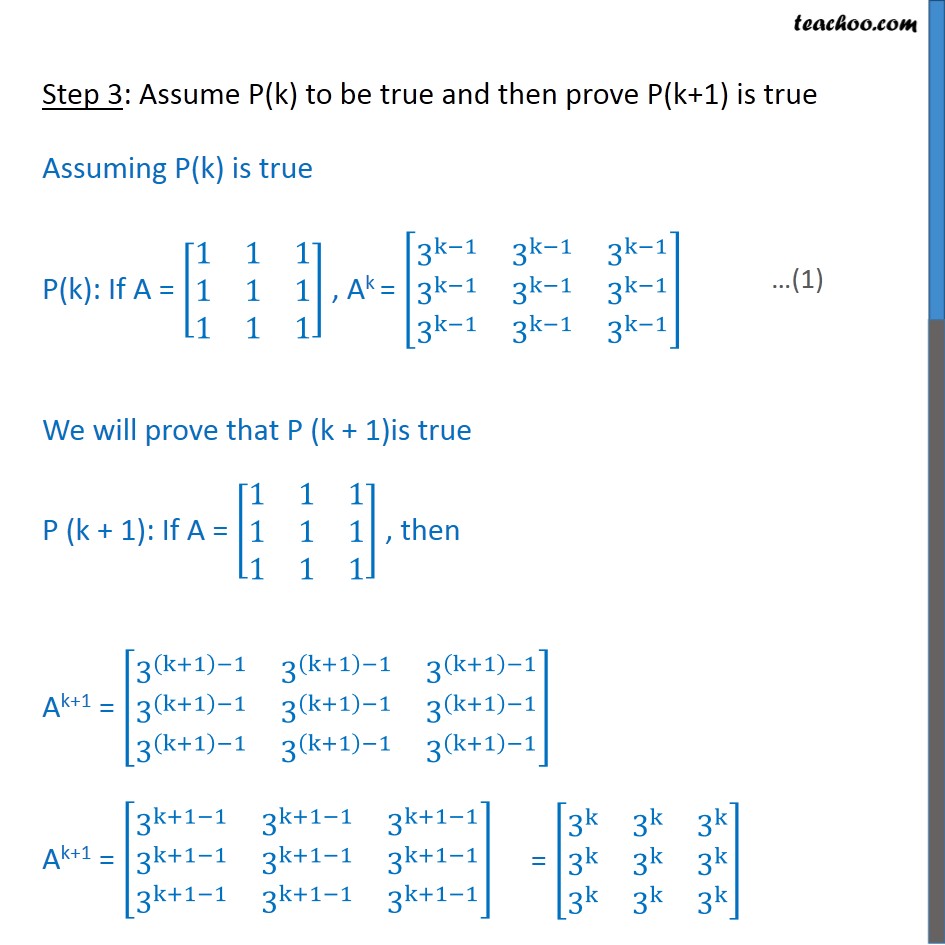
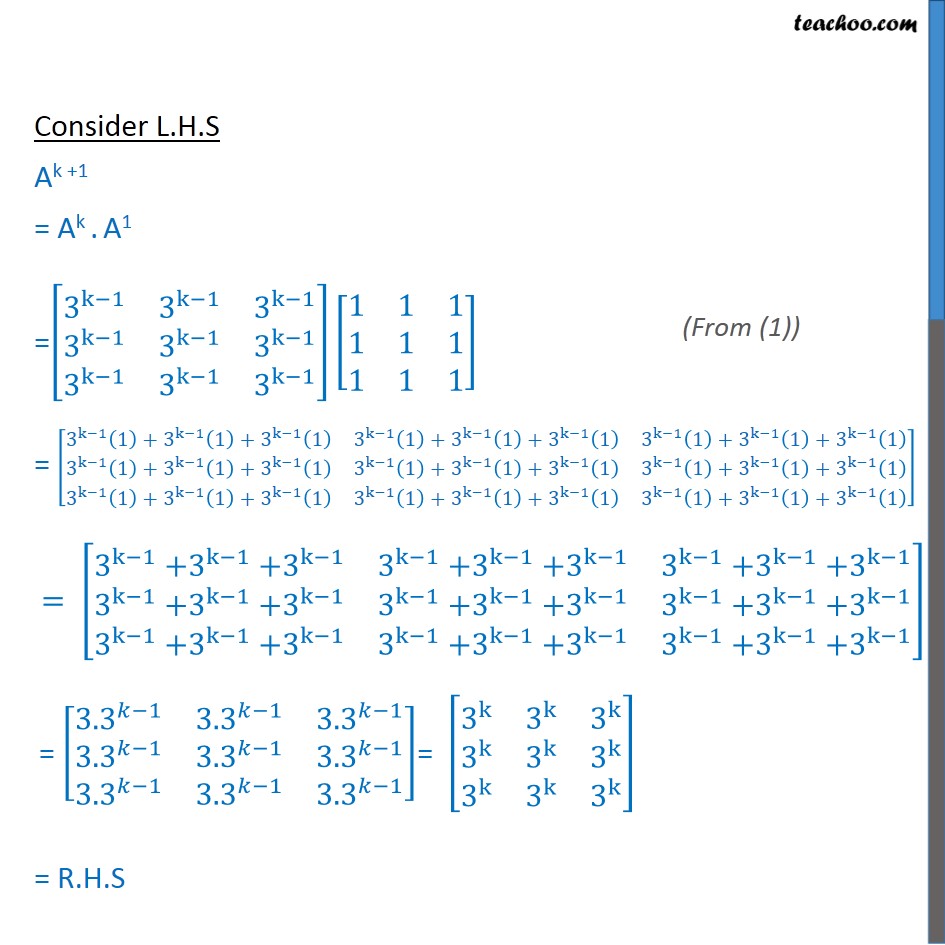
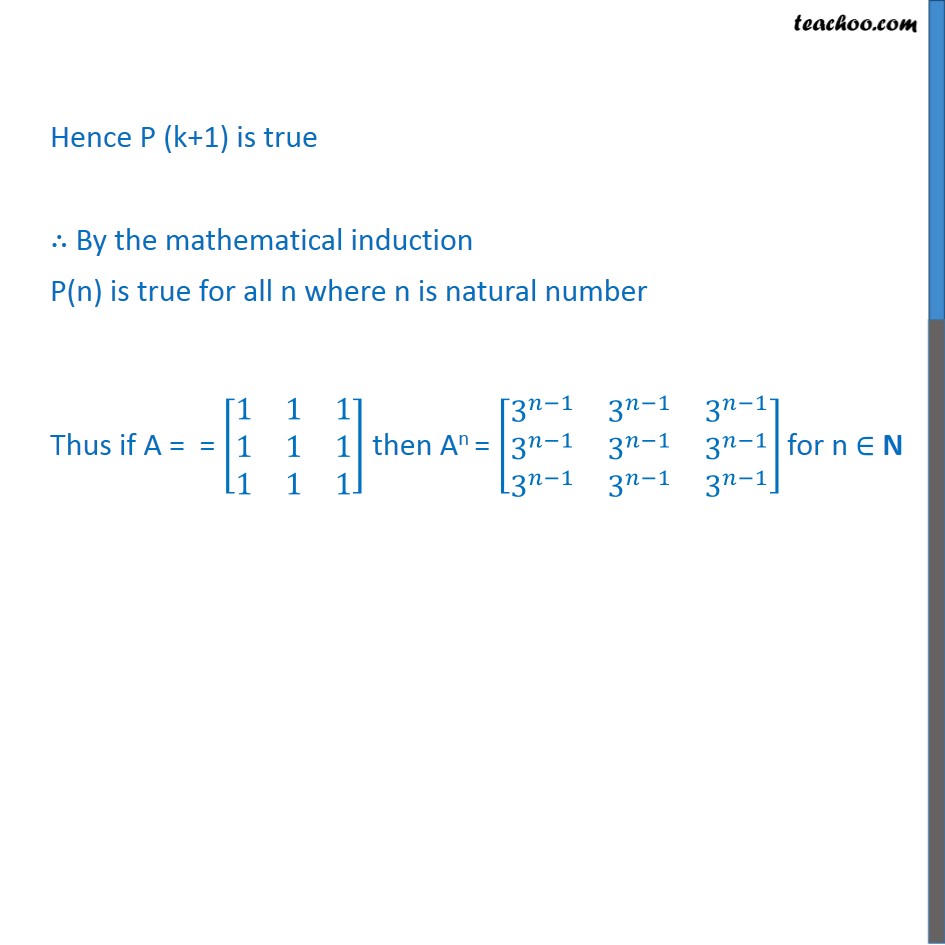
Misc. 2 If A = [ 8(1&1&1@1&1&1@1&1&1)] , prove that An = [ 8(3^( 1)&3^( 1)&3^( 1)@3^( 1)&3^( 1)&3^( 1)@3^( 1)&3^( 1)&3^( 1) )], n N We shall prove the result by using mathematical induction Step 1: P(n) : If A = [ 8(1&1&1@1&1&1@1&1&1)] , An = [ 8(3^( 1)&3^( 1)&3^( 1)@3^( 1)&3^( 1)&3^( 1)@3^( 1)&3^( 1)&3^( 1) )] Step 2: Prove for n = 1 For n = 1 L.H.S = A1 = A = [ 8(1&1&1@1&1&1@1&1&1)] R.H.S = [ 8(3^(1 1)&3^(1 1)&3^(1 1)@3^(1 1)&3^(1 1)&3^(1 1)@3^(1 1)&3^(1 1)&3^(1 1) )] = [ 8(30&30&30@30&30&30@30&30&30)] = [ 8(1&1&1@1&1&1@1&1&1)] So, L.H.S = R.H.S P(n) is true for n = 1 Step 3: Assume P(k) to be true and then prove P(k+1) is true Assuming P(k) is true P(k): If A = [ 8(1&1&1@1&1&1@1&1&1)] , Ak = [ 8(3^(k 1)&3^(k 1)&3^(k 1)@3^(k 1)&3^(k 1)&3^(k 1)@3^(k 1)&3^(k 1)&3^(k 1) )] We will prove that P (k + 1)is true P (k + 1): If A = [ 8(1&1&1@1&1&1@1&1&1)] , then Ak+1 = [ 8(3^((k+1) 1)&3^((k+1) 1)&3^((k+1) 1)@3^((k+1) 1)&3^((k+1) 1)&3^((k+1) 1)@3^((k+1) 1)&3^((k+1) 1)&3^((k+1) 1) )] Ak+1 = [ 8(3^(k+1 1)&3^(k+1 1)&3^(k+1 1)@3^(k+1 1)&3^(k+1 1)&3^(k+1 1)@3^(k+1 1)&3^(k+1 1)&3^(k+1 1) )] Consider L.H.S Ak +1 = Ak . A1 =[ 8(3^(k 1)&3^(k 1)&3^(k 1)@3^(k 1)&3^(k 1)&3^(k 1)@3^(k 1)&3^(k 1)&3^(k 1) )][ 8(1&1&1@1&1&1@1&1&1)] = [ 8(3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1)&3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1)&3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1)@3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1)&3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1)&3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1)@3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1)&3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1)&3^(k 1) (1)+3^(k 1) (1)+3^(k 1) (1) )] = [ 8(3^(k 1) +3 ^(k 1)+3^(k 1)&3^(k 1) +3 ^(k 1)+3^(k 1)&3^(k 1) +3 ^(k 1)+3^(k 1)@3^(k 1) +3 ^(k 1)+3^(k 1)&3^(k 1) +3 ^(k 1)+3^(k 1)&3^(k 1) +3 ^(k 1)+3^(k 1)@3^(k 1) +3 ^(k 1)+3^(k 1)&3^(k 1) +3 ^(k 1)+3^(k 1)&3^(k 1) +3 ^(k 1)+3^(k 1) )] = [ 8( 3.3 ^( 1)& 3.3 ^( 1)& 3.3 ^( 1)@ 3.3 ^( 1)& 3.3 ^( 1)& 3.3 ^( 1)@ 3.3 ^( 1)& 3.3 ^( 1)& 3.3 ^( 1) )]= [ 8(3^k&3^k&3^k@3^k&3^k&3^k@3^k&3^k&3^k )] = R.H.S Hence P (k+1) is true By the mathematical induction P(n) is true for all n where n is natural number Thus if A = = [ 8(1&1&1@1&1&1@1&1&1)] then An = [ 8(3^( 1)&3^( 1)&3^( 1)@3^( 1)&3^( 1)&3^( 1)@3^( 1)&3^( 1)&3^( 1) )] for n N