Question 7 - Chapter 2 Class 12 Inverse Trigonometric Functions (Important Question)

Last updated at Dec. 16, 2024 by

Example 13 - Solve tan-1 2x + tan-1 3x = pi/4 - Class 12

Example 13 - Chapter 2 Class 12 Inverse Trigonometric Functions - Part 2
Example 13 - Chapter 2 Class 12 Inverse Trigonometric Functions - Part 3

Go Ad-free

Transcript

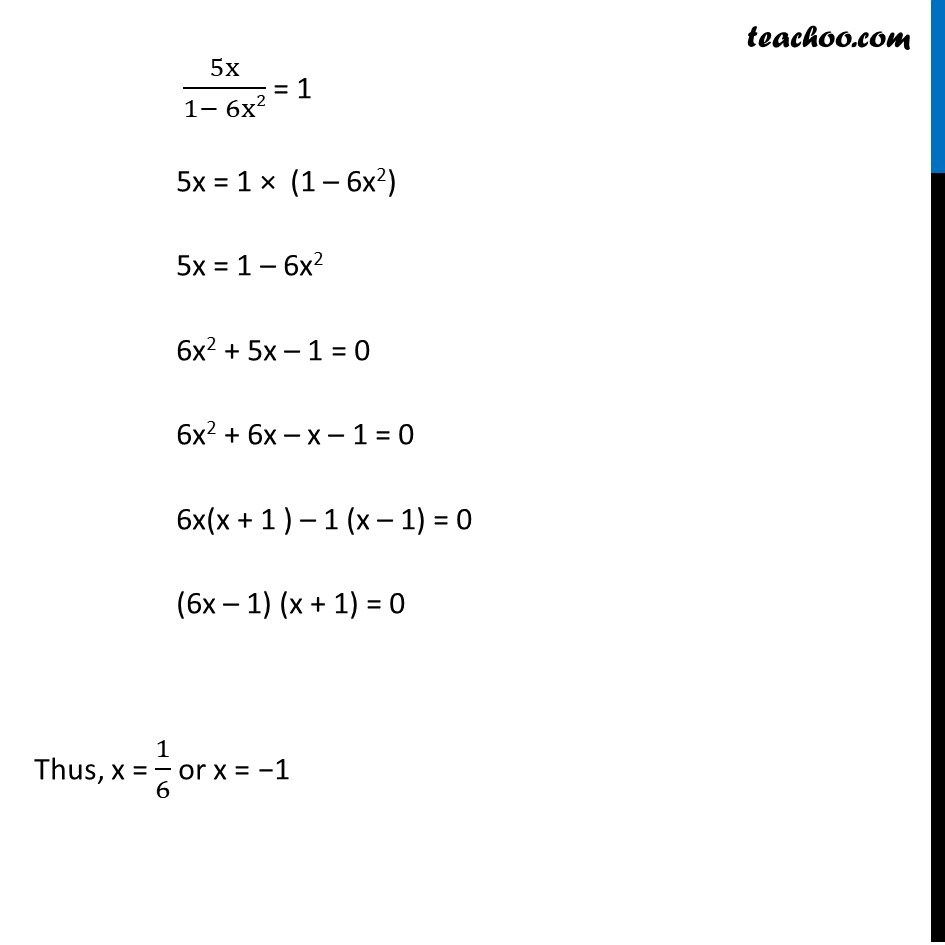
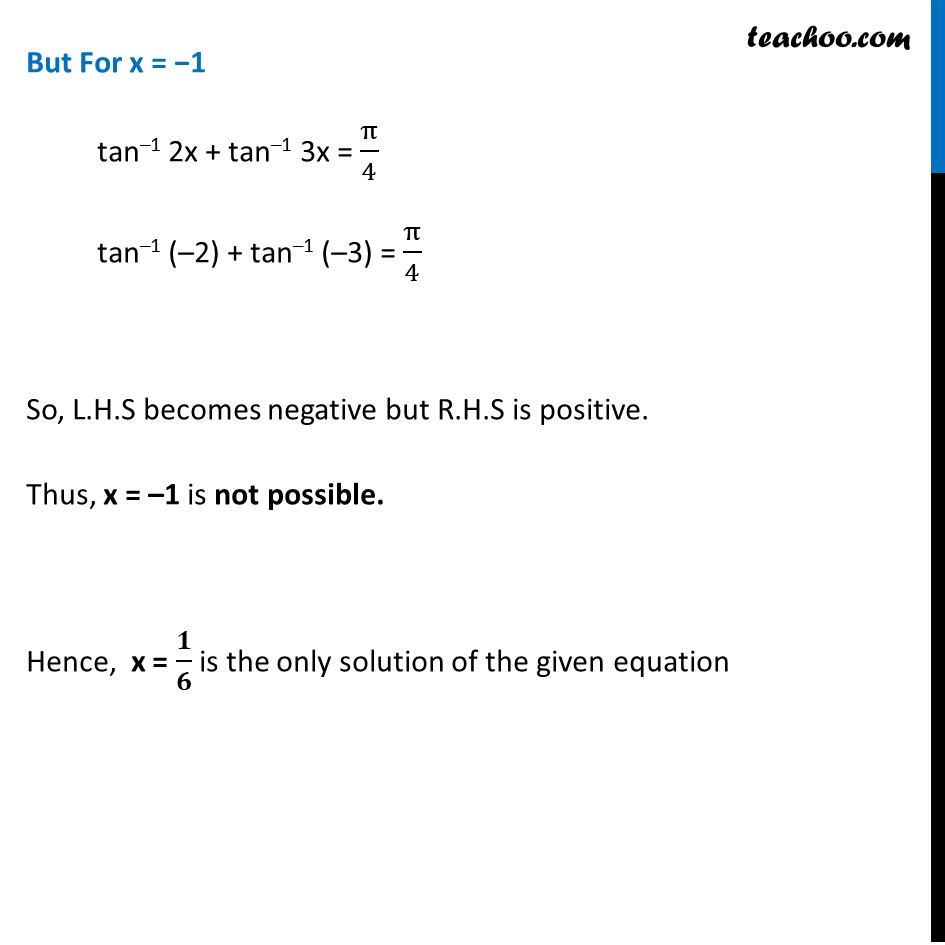
Question 7 Solve tan–1 2x + tan–1 3x = π/4 Given tan–1 2x + tan 3x = π/4 tan–1 ((2x + 3x)/(1 − 2x × 3x)) = π/4 𝟓𝐱/(𝟏 − 𝟔𝐱𝟐) = tan 𝝅/𝟒 We know that tan–1 x + tan–1 y = tan–1 ((𝐱 + 𝐲)/(𝟏 − 𝐱𝐲)) Replacing x by 2x & y by 3x 5x/(1− 6x2) = 1 5x = 1 × (1 – 6x2) 5x = 1 – 6x2 6x2 + 5x – 1 = 0 6x2 + 6x – x – 1 = 0 6x(x + 1 ) – 1 (x – 1) = 0 (6x – 1) (x + 1) = 0 Thus, x = 1/6 or x = −1 But For x = −1 tan–1 2x + tan–1 3x = π/4 tan–1 (–2) + tan–1 (–3) = π/4 So, L.H.S becomes negative but R.H.S is positive. Thus, x = –1 is not possible. Hence, x = 𝟏/𝟔 is the only solution of the given equation

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo