Ex 8.2, 7 - Chapter 8 Class 11 Sequences and Series
Last updated at Dec. 16, 2024 by Teachoo
Geometric Progression(GP): Formulae based
Ex 8.2, 6
You are here
You are here
Chapter 8 Class 11 Sequences and Series
Concept wise
- Finding Sequences
- Arithmetic Progression (AP): Formulae based
- Arithmetic Progression (AP): Statement
- Arithmetic Progression (AP): Calculation based/Proofs
- Inserting AP between two numbers
- Arithmetic Mean (AM)
-
Geometric Progression(GP): Formulae based
- Geometric Progression(GP): Statement
- Geometric Progression(GP): Calculation based/Proofs
- Inserting GP between two numbers
- Geometric Mean (GM)
- AM and GM (Arithmetic Mean And Geometric mean)
- AP and GP mix questions
- Finding sum from series
- Finding sum from nth number
Transcript
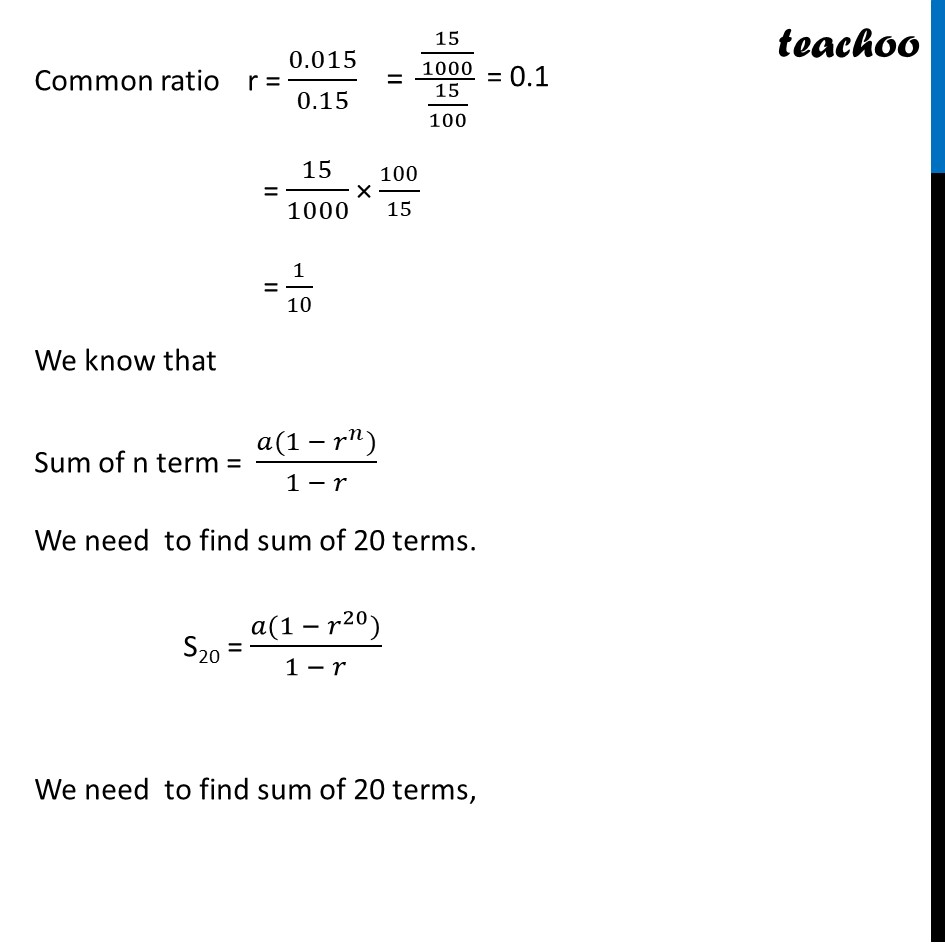
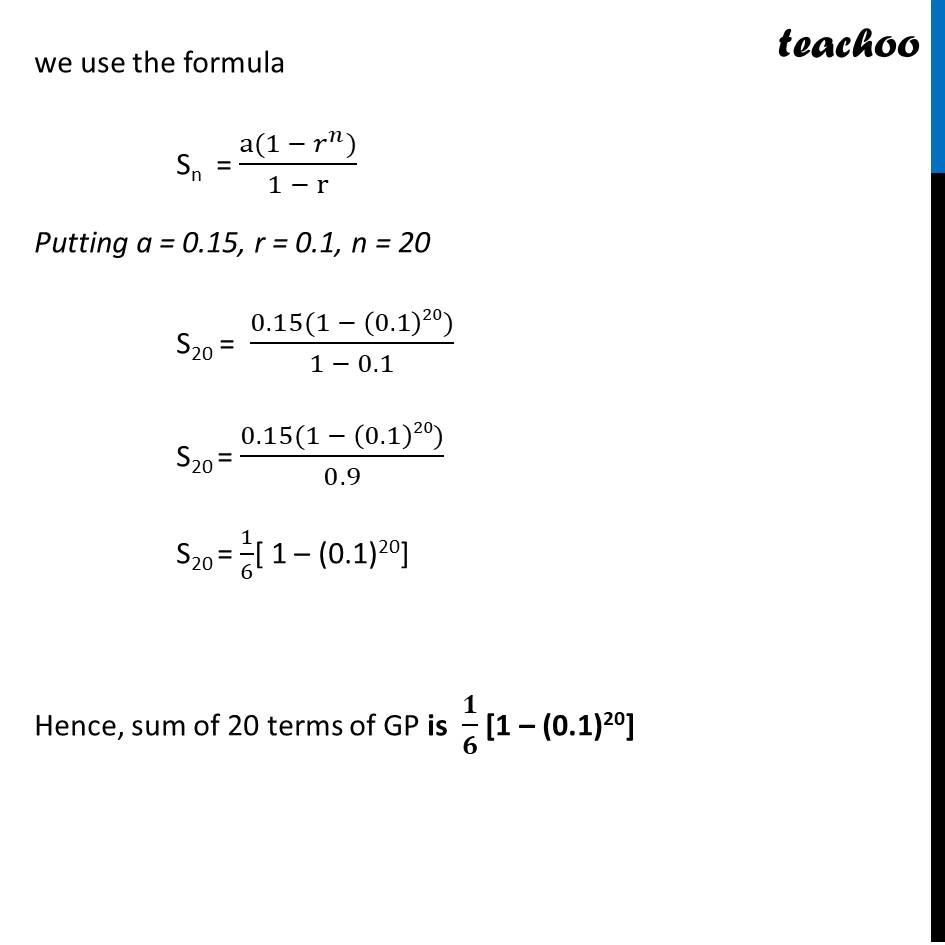
Ex 8.2,7 Find the sum to 20 terms in the geometric progression 0.15, 0.015, 0.0015 0.15, 0.015, 0.0015 We know that Sn = (a(1 ^ ))/(1 r) where Sn = sum of n terms of GP n is the number of terms a is the first term r is the common ratio First term a = 0.15 , Common ratio r = 0.015/0.15 ` = 15/1000 100/15 = 1/10 We know that Sum of n term = ( (1 ^ ))/(1 ) We need to find sum of 20 terms. S20 = ( (1 ^20))/(1 ) We need to find sum of 20 terms, we use the formula Sn = (a(1 ^ ))/(1 r) Putting a = 0.15, r = 0.1,n=20 S20 = (0.15(1 (0.1)20))/(1 0.1) S20 =(0.15(1 (0.1)20))/0.9 S20 = 1/6[ 1 (0.1)20] Hence, sum of 20 terms of GP is 1/6[ 1 (0.1)20]
