Ex 8.2, 1 (iv) - Chapter 8 Class 10 Introduction to Trignometry

Transcript

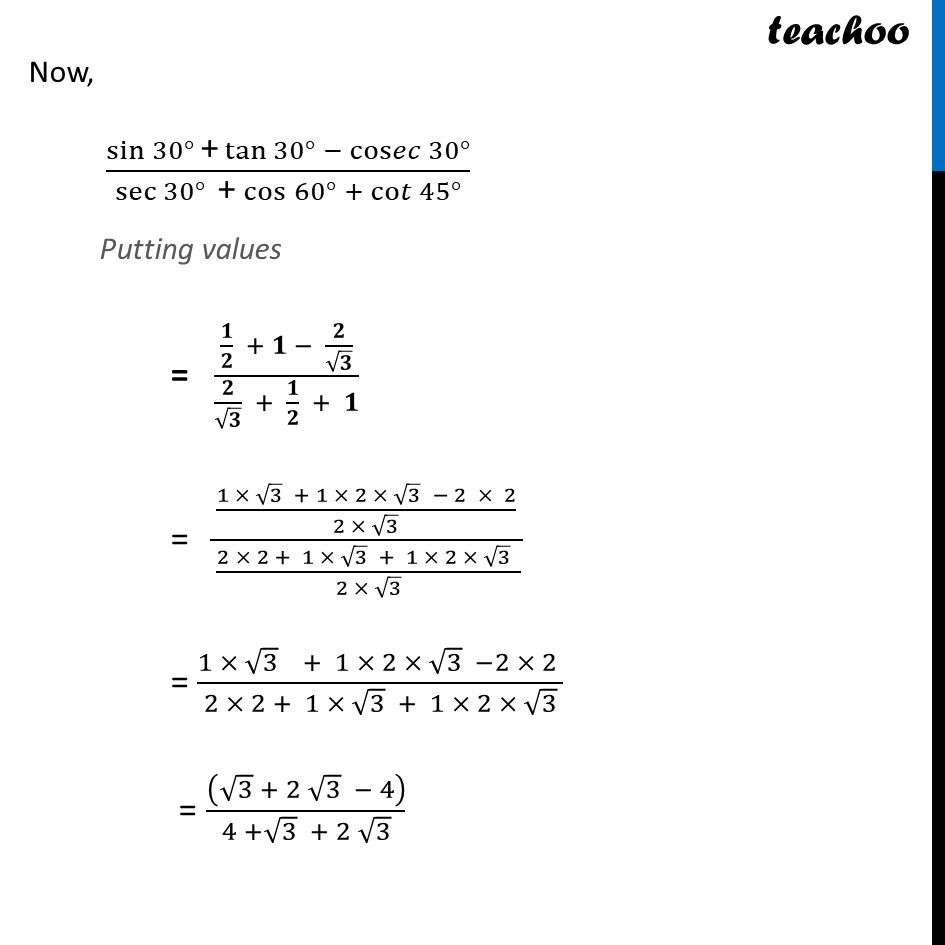
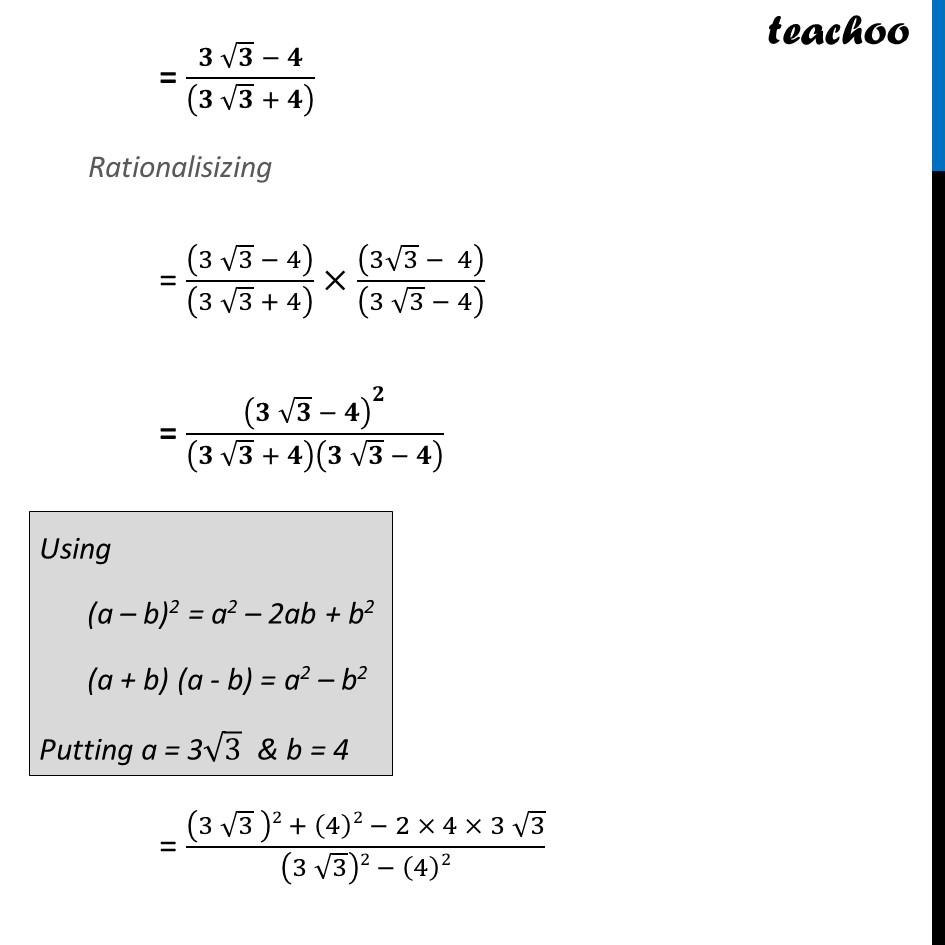
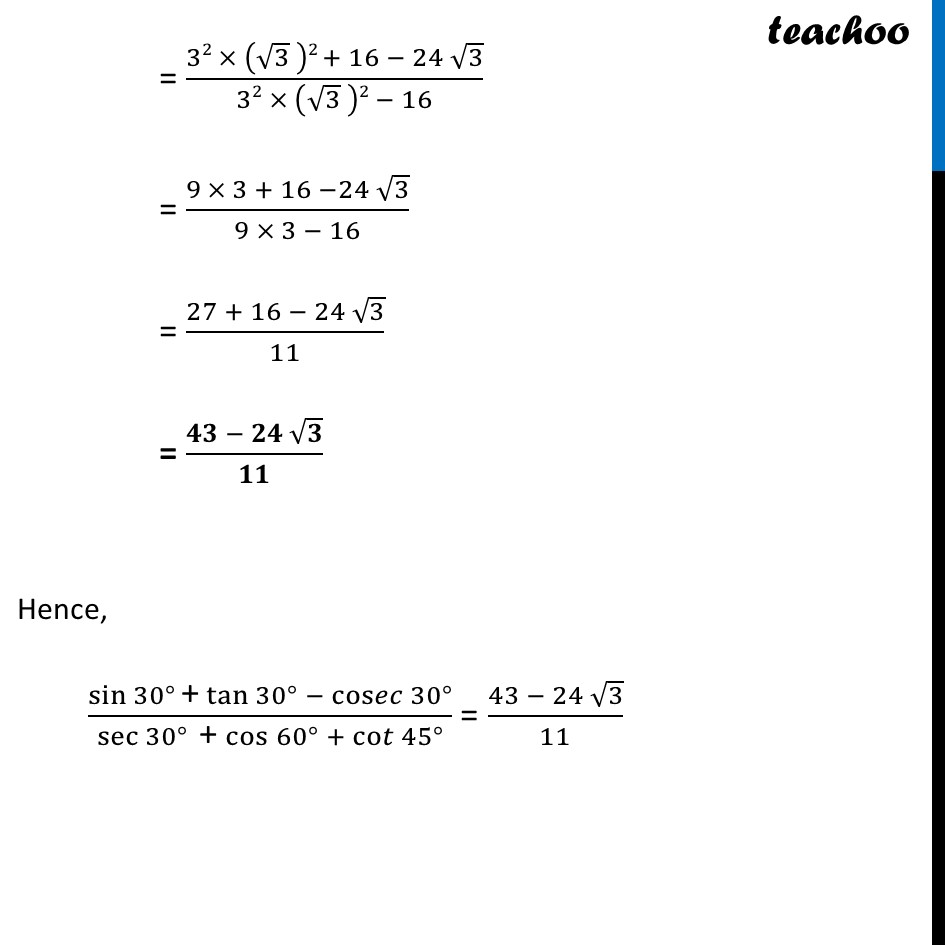
Ex 8.2, 1 Evaluate the following : "sin 30° + tan 45° – cosec 60°" /"sec 30° + cos 60° + cot 45°" We know that, sin "30°" = 1/2 tan "45°" = 1 cosec 𝟔"0°" = 1/sin⁡〖60°〗 = 2/√3 sec "30°" = 1/cos⁡〖30°〗 = 2/√3 cos "60°" = 1/2 cot "45°" = 1/〖tan 〗⁡〖45°〗 = 1/1 = 1 Now, (sin⁡〖30°〗 "+" tan 30°" " −〖 cos𝑒𝑐〗⁡〖30°〗)/(sec⁡〖30°〗 "+ " cos 60°" " + co𝑡⁡〖45°〗 ) Putting values = (𝟏/𝟐 + 𝟏 − 𝟐/√𝟑)/(𝟐/√𝟑 + 𝟏/𝟐 + 𝟏) = ((1 × √3 + 1 × 2 × √3 − 2 × 2)/(2 × √3) )/((2 × 2 + 1 × √3 + 1 × 2 × √3 )/(2 × √3)) = (1 × √3 + 1 × 2 × √3 −2 × 2 )/(2 × 2 + 1 × √3 + 1 × 2 × √3) = ((√3 + 2 √3 − 4))/(4 +√3 + 2 √3) = (𝟑 √𝟑 − 𝟒)/((𝟑 √𝟑 + 𝟒) ) Rationalisizing = ((3 √3 − 4))/((3 √3 + 4) )×((3√3 − 4))/((3 √3 − 4) ) = (𝟑 √𝟑 − 𝟒)^𝟐/(𝟑 √𝟑 + 𝟒)(𝟑 √𝟑 − 𝟒) = ((3 √3 )2 + (4)2 − 2 × 4 × 3 √3)/((3 √3)2 − (4)2) Using (a – b)2 = a2 – 2ab + b2 (a + b) (a - b) = a2 – b2 Putting a = 3√3 & b = 4 = (32 × (√3 )2 + 16 − 24 √3)/(32 × (√3 )2 − 16) = (9 × 3 + 16 −24 √3)/(9 × 3 − 16) = (27 + 16 − 24 √3)/11 = (𝟒𝟑 − 𝟐𝟒 √𝟑)/𝟏𝟏 Hence, (sin⁡〖30°〗 "+" tan 30°" " −〖 cos𝑒𝑐〗⁡〖30°〗)/(sec⁡〖30°〗 "+ " cos 60°" " + co𝑡⁡〖45°〗 ) = (43 − 24 √3)/11