Question 33 - CBSE Class 10 Sample Paper for 2021 Boards - Maths Standard - Solutions of Sample Papers for Class 10 Boards

Last updated at Dec. 16, 2024 by Teachoo

The mode of the following data is 67. Find the missing frequency x.

The mode of the following data is 67. Find the missing frequency x

Question 33 - CBSE Class 10 Sample Paper for 2021 Boards - Maths Standard - Part 2

Next: Question 34 (Choice - 1) → Remove Ads

Transcript

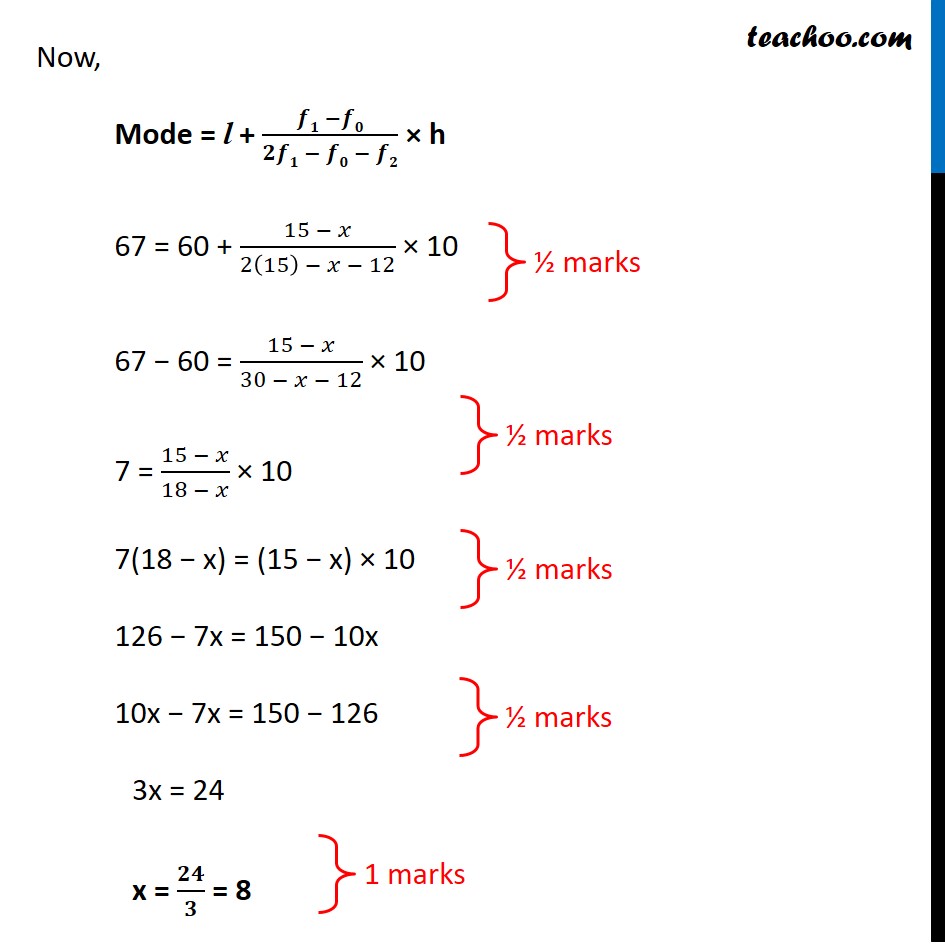
Question 33 The mode of the following data is 67. Find the missing frequency x.Given Mode = 67 ∴ Modal class is 60 − 70 Mode = l + (𝒇𝟏 −𝒇𝟎)/(𝟐𝒇𝟏 − 𝒇𝟎 − 𝒇𝟐) × h where l = lower limit of modal class h = class-interval f1 = frequency of the modal class f0 = frequency of the class before modal class f2 = frequency of the class after modal class Now, Mode = l + (𝒇𝟏 −𝒇𝟎)/(𝟐𝒇𝟏 − 𝒇𝟎 − 𝒇𝟐) × h 67 = 60 + (15 − 𝑥)/(2(15) − 𝑥 − 12) × 10 67 − 60 = (15 − 𝑥)/(30 − 𝑥 − 12) × 10 7 = (15 − 𝑥)/(18 − 𝑥) × 10 7(18 − x) = (15 − x) × 10 126 − 7x = 150 − 10x 10x − 7x = 150 − 126 3x = 24 x = 𝟐𝟒/𝟑 = 8

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo

Class 6

Class 7

Class 8

Class 9

Class 10

Class 11

Class 12

Courses

Interesting

Popular