Example 6 - Chapter 9 Class 7 Perimeter and Area

Last updated at Dec. 16, 2024 by

Slide11.JPG

Slide12.JPG
Slide13.JPG

Go Ad-free

Transcript

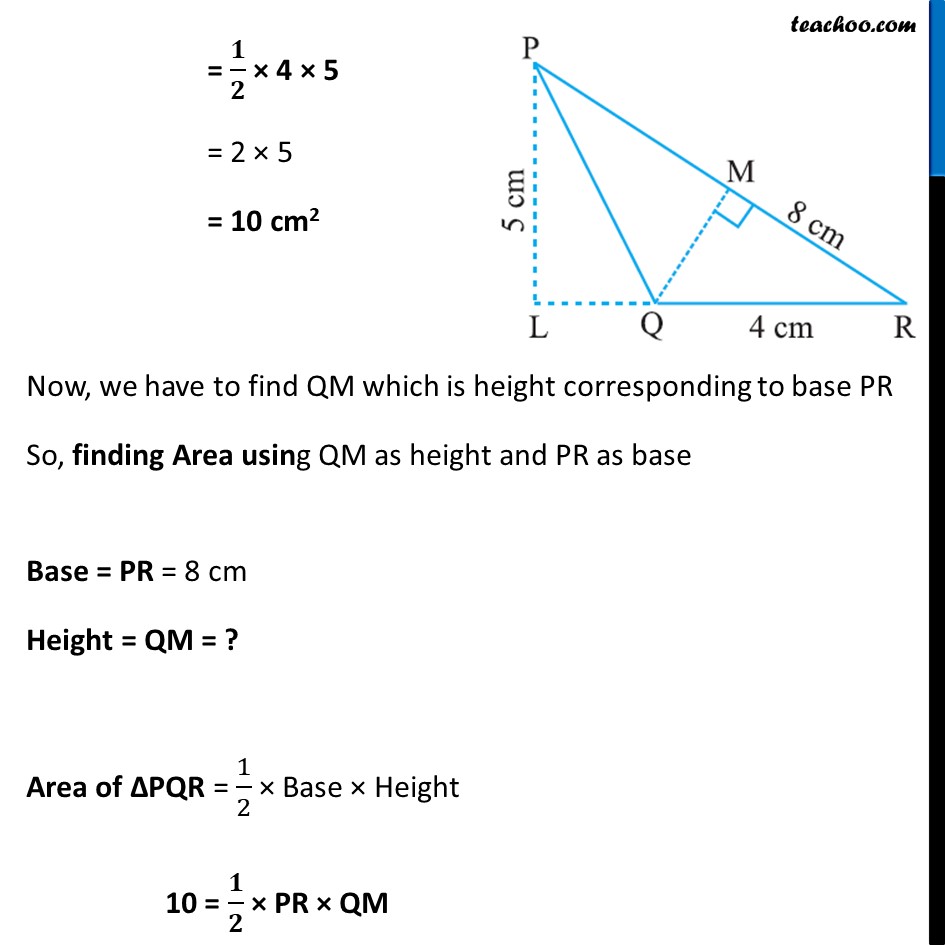
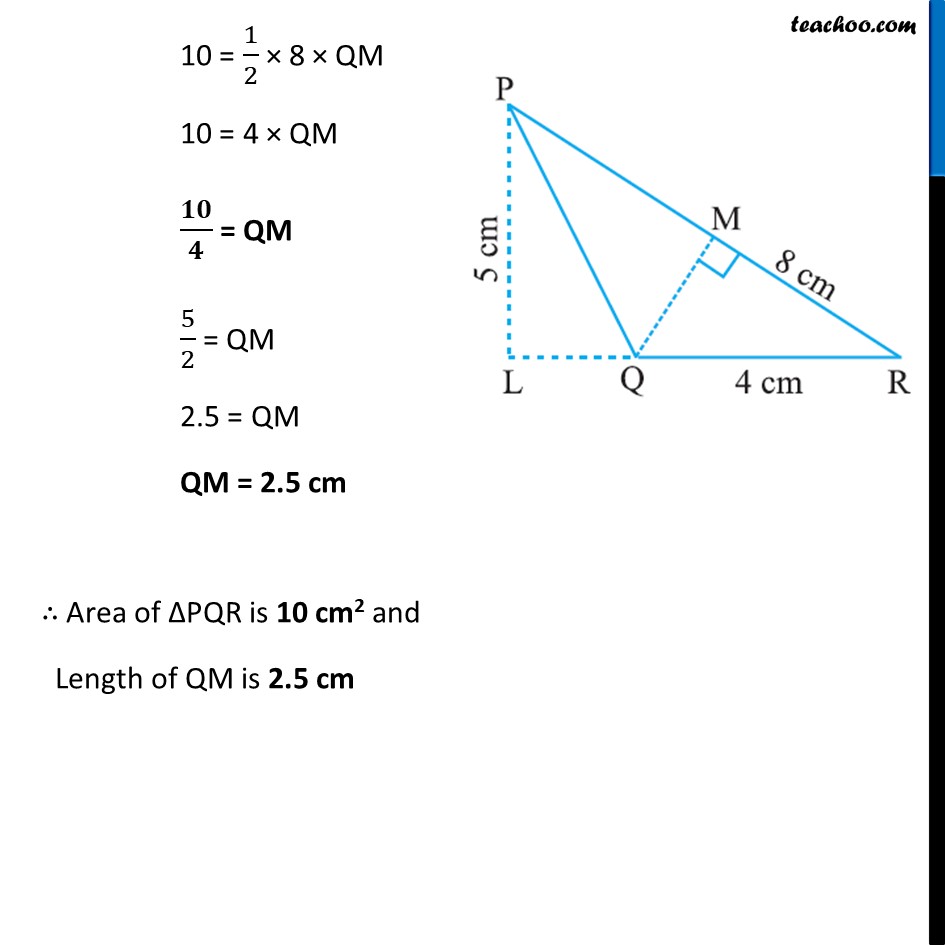
Example 6 In ΔPQR, PR = 8 cm, QR = 4 cm and PL = 5 cm (Fig 11.22). Find: (i) the area of the ΔPQR Find: (ii) QMFinding area of ∆PQR using QR as base and PL as height Here, Base = QR = 4 cm Height = PL = 5 cm Area of ∆PQR = 1/2 × Base × Height = 𝟏/𝟐 × 4 × 5 = 2 × 5 = 10 cm2 Now, we have to find QM which is height corresponding to base PR So, finding Area using QM as height and PR as base Base = PR = 8 cm Height = QM = ? Area of ∆PQR = 1/2 × Base × Height 10 = 𝟏/𝟐 × PR × QM 10 = 1/2 × 8 × QM 10 = 4 × QM 𝟏𝟎/𝟒 = QM 5/2 = QM 2.5 = QM QM = 2.5 cm ∴ Area of ∆PQR is 10 cm2 and Length of QM is 2.5 cm

Davneet Singh's photo - Co-founder, Teachoo

Made by

Davneet Singh

Davneet Singh has done his B.Tech from Indian Institute of Technology, Kanpur. He has been teaching from the past 14 years. He provides courses for Maths, Science and Computer Science at Teachoo